Geodesics in $\mathbb{R}^2 \times \mathbb{S}^1$ under "segment" metric

Question

Represent the position of a unit-length, oriented segment $s$ in the plane by the location $a$ of its basepoint and an orientation $\theta$: $s = (a,\theta)$. So $s$ can be viewed as a point in $\mathbb{R^2} \times \mathbb{S^1}$. Now I'll define a metric on this space. Define the distance $d(s_1,s_2)$ between two positions of unit-length segments as the average distance between their corresponding points:
     
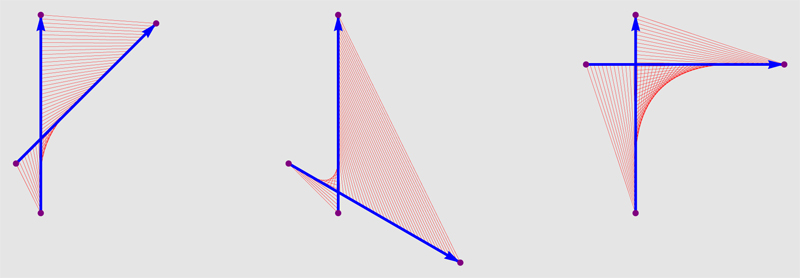
Above the distances are about 0.31, 0.61, and 0.53, left-to-right.

So if the endpoints of $s_i$ are $a_i$ and $b_i$, then $d(s_1,s_2)$ is the average of the Euclidean distances between $(1-t) a_1 + t b_1$ and $(1-t) a_2 + t b_2$ as $t$ varies in $[0,1]$. This is indeed a metric, I believe, because the triangle inequality holds between corresponding points in three positions of the segment. This metric is intended to capture the intuitive notion of how much work is required to move $s_1$ to $s_2$.

My question is: What are the geodesics in this space under this metric? Certainly a pure translation of $s$ is a geodesic. It seems that a pure rotation by at most $\pi$ of $s$ about a point $p \in s$ should also be a geodesic, but even this is not so clear to me. Certainly a rotation about a point not on $s$ is (generally) not a geodesic. Of course the main interest would be in geodesics that mix translation and rotation, showing (locally) optimal repositioning paths.

I investigated this long ago when working on motion-planning algorithms ("moving a ladder"), but got quite blocked on this natural question. This superficially seems related to the Kakeya needle problem, but the metric I propose does not measure swept area. Perhaps it has been studied in some guise previously. If so, a pointer would be appreciated. Thanks!

Addenda. (26Sep11.) I just ran across this book, by V. A. Dubovit͡s︡kiĭ, which seems relevant: The Ulam problem of optimal motion of line segments, Translation Series in Mathematics and Engineering, Optimization Software, 1985. It may take some time for me to locate a copy...

(11Nov11). I finally have this book in my hands. The Preface by Hestenes says,

Dubovitskij has succeeded in solving in closed form a generalization of a problem of S[.] Ulam..: Among all continuous motions of an oriented line segment $S$ in $\mathbb{E}^n$ from one position to another, which preserves its length [...], find one for which the sum of the lengths of the paths swept by its endpoints is minimal.

The concentration here on the motion of the endpoints—in contrast to the average distance metric I proposed—seems to render these results as not directly relevant, although nevertheless quite interesting.

Answer 1

Let us start with the metric on $\mathbb R^4=\mathbb R^2\times \mathbb R^2$ defined by the norm $\|{*}\|$ defined by $$\|(x,y)\|=\int_0^1|t\cdot x+(1-t)\cdot y|\,dt,$$ where $|{ * }|$ denotes the Euclidean norm on $\mathbb R^2$. This norm is not strongly convex, so you should expect many geodesics between close points.

Now, your metric is the intrinsic metric induced on the hypersurface $\Sigma$ described by $|x-y|=1$. So, you have a Finsler metric on $\Sigma=\mathbb S^1\times \mathbb R^2$. The unit ball in the tangent plane is isometric the intersection of the ball in the above norm with the 3-dimensional subspace in one special direction. It seems that this intersection is strongly convex. The metric is smooth (since there is a transitive isometric group action on $\mathbb S^1\times \mathbb R^2$). It remains to write a differential equation for the geodesics; this should be in any book on Finsler geometry. (It should be a pain, but it might help.)

Answer 2

In addition to Anton Petrunin's answer, here is a trick to simplify (and in some sense solve) the geodesic equation.

Since the metric has three-dimensional group of isometries (generated by rigid motions of the plane), the corresponding Noether's integrals make the geodesic flow completely integrable. More precisely, the first derivative is uniquely determined by the position and the values of the integrals. Actually a two-dimensional group is sufficient because for the third integral one can always take the speed (= the norm of the velocity vector) of a geodesic.

So let's use invariance under parallel translations only. We have a manifold of all unit segments in the plane, and a tangent vector to that manifold can be thought of as the vector field along a segment (representing velocities of all its points). This vector field has a form $$ v(t) = tx + (1-t)y, \qquad t\in[0,1] $$ where $x,y\in\mathbb R^2$ are such that the vector $x-y$ is orthogonal to the segment. The norm of this tangent vector is $\int |v(t)| dt$ (as in Anton's answer), and the Noether integral corresponding to translations (if my quick computation is correct) boils down to the following: if $v$ is a velocity vector of a geodesic represented in the above form, then $$ \int_0^1 \frac{v(t)}{|v(t)|} \ dt = V_0 $$ where $V_0$ is a constant (for each geodesic) vector in the plane. This vector $V_0$ (and, say, the assumption that the geodesic is unit-speed) determines the parameters $x$ and $y$ in the formula for $v(t)$ uniquely (as a function of the segment's current direction), so the geodesic equation is reduced to a 1st order ODE. Furthermore, the above integral can be found as an explicit (but weird) function of $x$ and $y$, so the equation becomes easy to solve, at least numerically.

By the way, I second Jean-Marc Schlenker's proposal to consider quadratic mean of the distances (i.e. $L^2$ norm rather than $L^1$), especially if you have any physics-related application in mind. In this case the metric is Riemannian, the energy is just the standard kinetic energy of the moving segment, so the Noether intergrals are just the standard conservation laws: the linear momentum and the angular momentum. And the geodesics are very simple: the segment rotates at a fixed angular speed while its midpoint (the barycenter) moves along a straight line with a constant speed.

Answer 3

What follows are just some illustrations, not a full answer; please refer to Anton Petrunin's answer for a nice description of the 4-dimensional geometry that the original question is embedded in.

Here's a bit of Mathematica code to generate some crude discrete approximations to geodesics. Given the two endpoint segments $s_0,s_1$, I create $n$ segments on the naïve $s_\alpha$ path I defined in the comments above, normalize their lengths to one, and then vary the positions of the endpoints of these intermediate segments with Mathematica's FindMinimum function to find an approximate geodesic. The code I wrote looks for a local minimum of an objective function with two terms: one is just the sum of the distances between all the intermediate segments and the other is a constraint that forces the distances between each pair of adjacent segments on the path to be equal (otherwise the intermediate segments all flow to the endpoints). The segments are all constrained to have unit length.

As Mathematica is not really good at a serious minimization problem, the code runs rather slowly (finding a discrete path with $n=10$ takes about 7 minutes), but perhaps you might still be able to get some more direct intuition for the geodesics by playing around with it in different cases. It's a start, anyways.

Below is an image of one example. The endpoints are a segment with endpoints $(0,0)$ to $(1,0)$ (orange) and $(1,0)$ to $(1,1)$ (red), and I approximated a geodesic with a chain of $n=10$ segments. The path begins with the orange segment sliding upwards a tiny bit to "yellow", and then the segments rotates counter clockwise and translate right until they reach red.

The segment distance between red and orange is $\frac{1}{8}\left(4+\sqrt{2}\log(3+2\sqrt{2})\right)\approx0.8116$, but the length of the approximate geodesic is $0.865$. Each pair of "adjacent" segments in the picture has a segment distance roughly 0.096 between them.

With $n=10$, the length has not converged to high accuracy! For $n=7,8,9$ the lengths of my approximations are $0.857,0.884,0.876$, respectively. In any case, it's clear that the length of the true geodesic will be greater than the distance between the endpoints. You might stare at this picture and imagine the true geodesic "hugging" the 3 dimensional unit length segment hypersurface in the 4D space, whereas the distance measures a "chord" through the 4 dimensional space of segments with arbitrary length.

update

As Joseph O'Rourke points out in the comments, the code is not very good with (anti-)parallel configurations. What seems to work is to give either segment a slight perturbation.

As an example, here's an approximate geodesic ($n=10$ points) between a segment with endpoints $(1,0)$ to $(2,0)$ (orange) and a segment with endpoints $(-1,0)$ to $(-1+\cos(\pi+0.001),\sin(\pi+0.001))\approx(-2+5\times10^{-7},0.001)$. The distance between the endpoints is $\approx3-2\times10^{-7}$, but the length of the depicted approximate geodesic is 3.30 (with steps of about 0.367).

Interestingly, this approximate geodesic seems to break symmetry in two ways. First, the segments rotate clockwise while traveling left. Second, the picture doesn't have left-right symmetry, which means that the first half of the journey is different from the second half (an analogue of this can be seen in the example above too, which doesn't have reflection symmetry across the -45º line). Is the second effect just due to discretization or non-convergence of the minimization? I don't know how to show that the true geodesics must be symmetric if there's some symmetry relating the two endpoints.

code snippet for this:

a1 = {1, 0}; b1 = {2, 0}; a2 = {-1, 0}; b2 = {-1 + Cos[\[Pi] + .001], Sin[\[Pi] + .001]};
Timing[anti3 = FindChain[{a1, b1}, {a2, b2}, 10]]
SegmentDist2[{a1, b1}, {a2, b2}]
Table[SegmentDist2[anti3[[i]], anti3[[i + 1]]], {i, 9}]
Sum[SegmentDist2[anti3[[i]], anti3[[i + 1]]], {i, 9}]
Graphics[Table[{Hue[i/Length[anti3]], Line[anti3[[i]]]}, {i, Length[anti3]}]]

Answer 4

A useful keyword for this problem is the Wasserstein distance, see wikipedia. I believe that this Wasserstein distance, for $p=1$, provides a variant of the distance you're considering but for unoriented segments. There is a well-developed theory here, in particular concerning the geodesics and their behavior. Incidentally things my turn out to be easier if you take the means of the squares of the distances, rather than of the distances.