Tiling with incommensurate triangles

Question

Say that two triangles are incommensurate if they do not share an edge length or a vertex angle, and their areas differ. Suppose you'd like to tile the plane with pairwise incommensurate triangles. I can think of at least one strategy.

Spiral out from an initial triangle in the pattern depicted below, with the red extensions chosen to avoid length/angle/area coincidences with all previously constructed triangles.

---

         
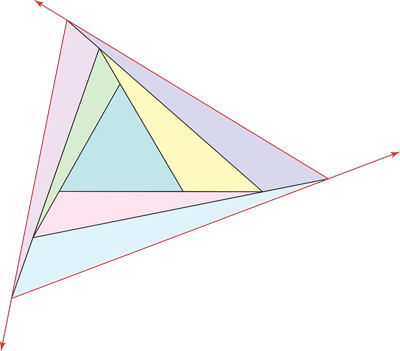
          ^{The central $\triangle$ is slightly non-equilateral. All $\triangle$s incommensurate.}

---

It seems clear that this approach could work, although it might not be straightforward to formalize to guarantee incommensurate triangles. Which brings me to my question:

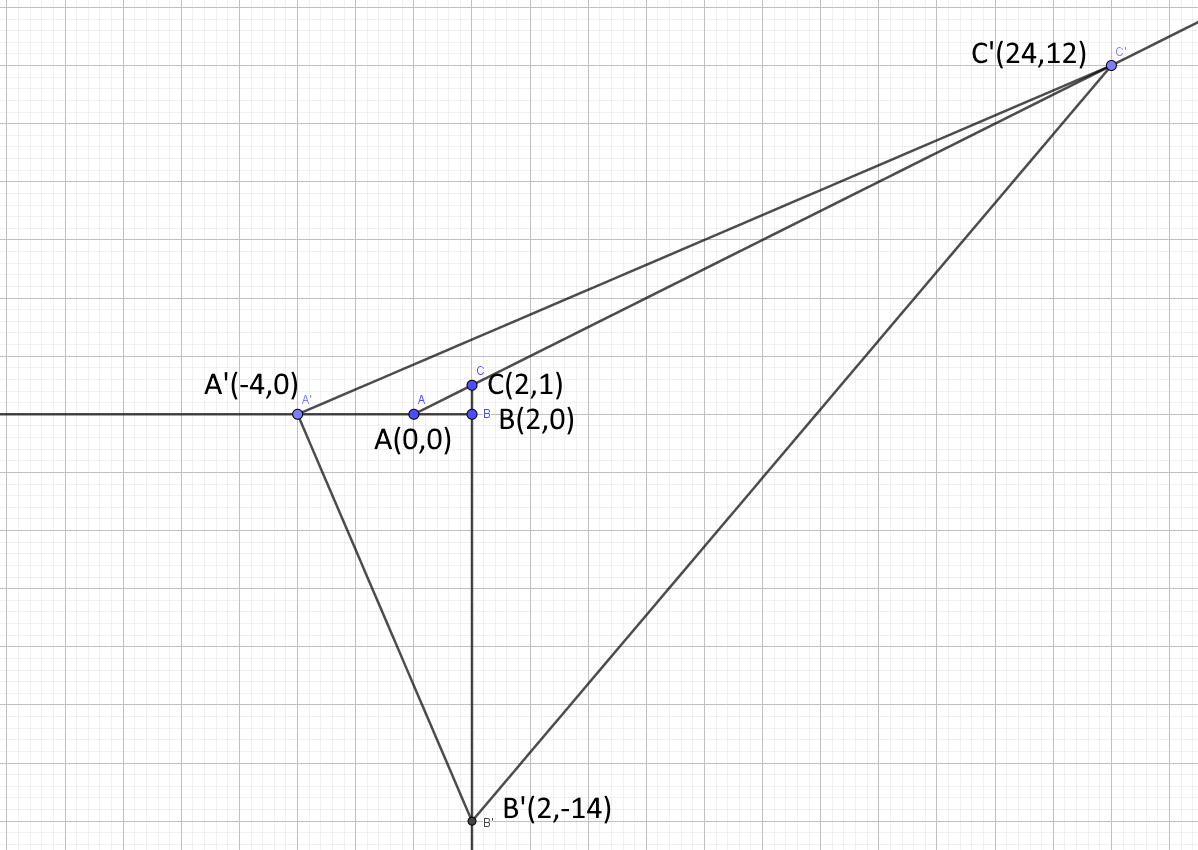
Q. What is a scheme that details a lattice tiling—all vertices at points of $\mathbb{Z}^2$—composed of pairwise incommensurate triangles?

This requires a more explicit design that effectively describes the triangle corner coordinates in a way that makes it evident that no lengths/angles/areas are duplicated. Without such a clear description, it is not even immediately evident (to me) that it is possible.

The same question may be asked for incommensurate simplex tilings with vertices in $\mathbb{Z}^d$.

---

See also: Tiling the plane with incongruent isosceles triangles.

Answer 1

Edit: This is a solution for non-commensurate side lengths only. The question also asks for non-commensurate angles, which this example does not provide.

I follow your approach. I start with a triangle with points in $\mathbb{Z}^2$ and add three incommensurate triangles, which leads to a new large triangle that is congruent to the first one and has points in $\mathbb{Z}^2$. We can repeat this process to tile the plane.

The first points are $A(0,0), B(2,0), C(2,1)$ as in the following image.

We now add three new points $A'(-4,0), B'(2,-14), C'(24,12)$ on the rays. We note:

(1) All new side lengths are strictly larger than the side lengths of the triangle $A,B,C$.

(2) All new side lengths are distinct (exercise).

(3) The new triangle $\Delta C'A'B'$ is congruent to $\Delta ABC$, in fact there is a right angle at $A'$ and the side $A'C'$ is double as large than $A'B'$.

The new arrangement of the four triangles is incommensurate by (1) and (2).

We can now repeat this process. Since (3) holds, we can use the congruency from $\Delta ABC$ to $\Delta A'B'C'$ to get the next larger triangle. By (1), all new lengths are larger than all old lengths and the new lengths are also distinct by (2). Moreover all points lie on $\mathbb{Z}^2$.

We can fill up all of $\mathbb{R}^2$ with incommensurate triangles.

Interesting follow up questions:

• Is there a tiling in which the size of the triangles is bounded?
• How fast do the lengths grow?