5
$\begingroup$

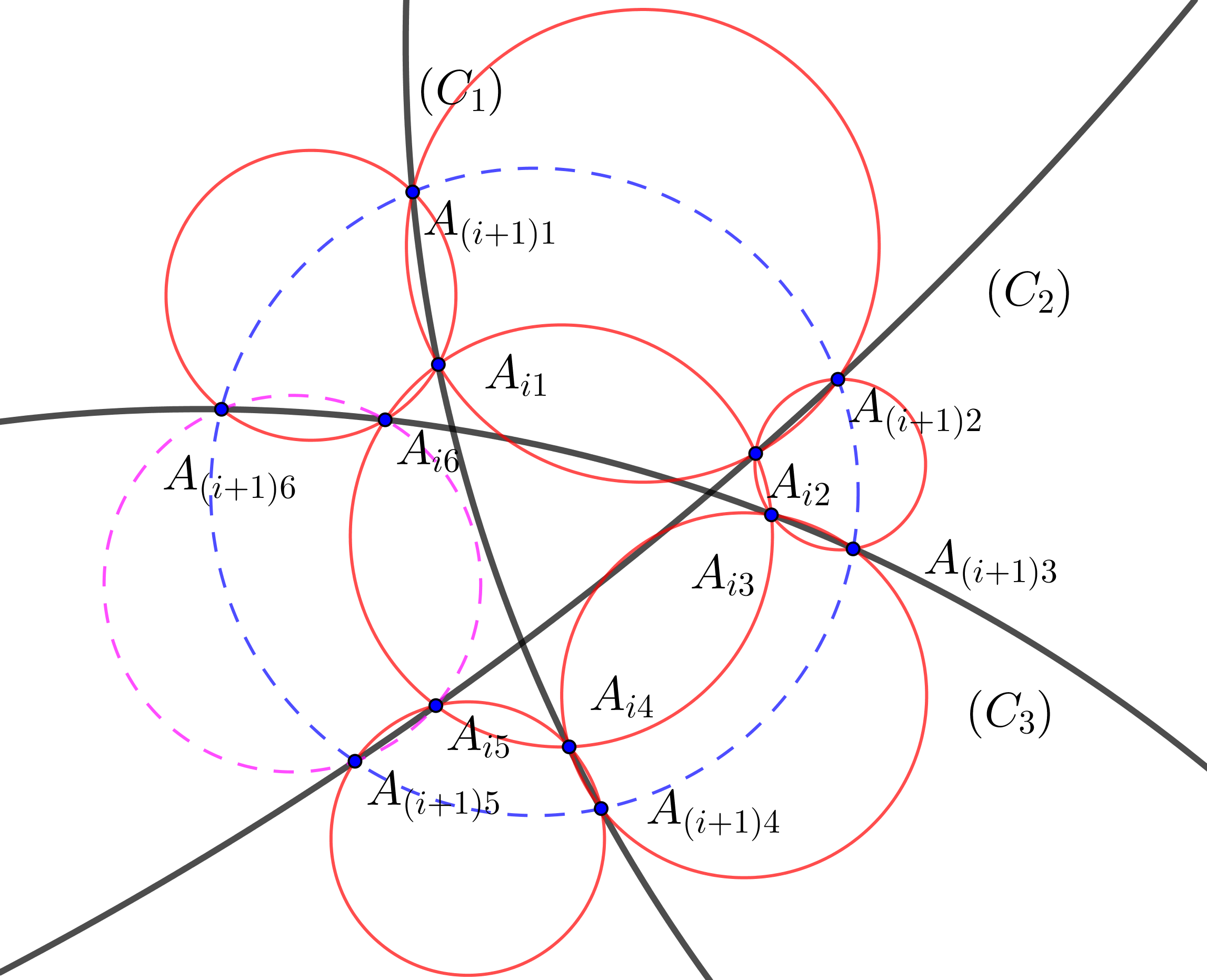
Since 2013, I found a very nice configuration: $N$-th closed chain of six circles. This is a generalization of theorem 1, problem 2 in here and theorem 2 in here and here (and is also generalization of Pascal theorem even with $1$st-chain of six circles). I am looking for a proof of one of these following problems:

enter image description here

Let $(C_{1})$, $(C_{2})$, $(C_{3})$ be three circles in a plane. Let $A_{i1}, A_{i4}$ be points lie on circle $(C_{1})$; $A_{i2}, A_{i5}$ be points lie on circle $(C_{2})$; $A_{i3}, A_{i6}$ be points lie on circle $(C_{3})$ for $i=1, 2, \cdots n$.

So that six points $A_{11}$, $A_{12}$ , $A_{13}$, $A_{14}$, $A_{15}$, $A_{16}$, lie on a circle and four poins $A_{ij}$, $A_{i{j+1}}$, $A_{{i+1}{j+1}}$, $A_{{i+1}{j}}$ lie on a circle for $i=1$, $2$, $\cdots$, $n$ and $j=1, 2, \cdots, 5$

Problem 1.(Already has a proof in here) Four points $A_{i5}, A_{i6}, A_{{(i+1)}5}, A_{{(i+1)}6}$ lie on a circle and six points $A_{i1}$, $A_{i2}$, $A_{i3}$, $A_{i4}$, $A_{i5}$, $A_{i6}$ lie on a circle for $i=1$, $2$, $\cdots$, $n$.

Denote $(O_{ij})$ is the circle through $A_{ij}$, $A_{i{j+1}}$, $A_{{i+1}{j+1}}$, $A_{{i+1}{j}}$ for $i=1$, $2$, $\cdots$, $n$ and $j=1$, $2$, $3$, $4$, $5$, $6$

Problem 2. Three lines $O_{i1}O_{k4}$, $O_{i2}O_{k5}$, $O_{i3}O_{k6}$ are concurrent for all $i, k =1, 2, \cdots$

Problem 3. Three lines $O_{i1}O_{k1}$, $O_{i3}O_{k3}$, $O_{i5}O_{k5}$ are concurrent, for all $i, k =1, 2, \cdots$

Problem 4. Three lines $O_{i2}O_{k2}$, $O_{i4}O_{k4}$, $O_{i6}O_{k6}$ are concurrent for all $i, k =1, 2, \cdots$

Problem 5. If $(O_{i1})$ meets $(O_{k1})$ at wo points $P_1$, $P'_1$; $(O_{i2})$ meets $(O_{k2})$ at wo points $P_2$, $P'_2$, $\cdots$, similarly $(O_{i6})$ meets $(O_{k6})$ at wo points $P_6$, $P'_6$ then $12$ points $P_1, \cdots, P_6, P'_1, \cdots, P'_6$ lie on a circle.

Problem 6. If $(O_{i1})$ meets $(O_{k4})$ at wo points $P_1$, $P_4$, $(O_{i2})$ meets $(O_{k5})$ at wo points $P_2, P_5$, $(O_{i3})$ meets $(O_{k6})$ at wo points $P_3$, $P_6$. Then six the six points lie on a circle $P_1,\cdots, P_6$ lie on a circle. $i = 1, 2, \cdots$

Note: The case $1$-st. Dao Thanh Oai, Problem 3845, Crux mathematicorum, issue May, 2013

enter image description here

See also:

Improve this question
$\endgroup$
7
  • $\begingroup$ Is "7. More and more properties" part of the question? And what is the status of the stuff that comes after it ("2-The case 1st...")? $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Dec 16, 2018 at 3:25
  • $\begingroup$ I mean there are many peroperties of this configuration. $\endgroup$
    – Đào Thanh Oai
    Commented Dec 16, 2018 at 3:30
  • $\begingroup$ I agree, and it is beautiful, but you should separate out a clearly stated question. Your post is meant to be a question. $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Dec 16, 2018 at 3:32
  • $\begingroup$ @მამუკაჯიბლაძე I deleted 7. $\endgroup$
    – Đào Thanh Oai
    Commented Dec 16, 2018 at 3:34
  • $\begingroup$ I don't think you should. It was completely OK to inform the reader that this configuration has a lot more properties. What is not OK (for me) is that by the time I finished reading, I could not figure out what exactly do you ask. Presumably how to prove that certain six points lie on a circle and certain other four points lie on another circle? Why don't you clearly formulate this? $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Dec 16, 2018 at 3:37

0

Reset to default

You must log in to answer this question.