From a talk, we learned that
- The symplectic volume of the space $M$ of gauge equivalence classes of flat G connections is given by the “Witten zeta function”:
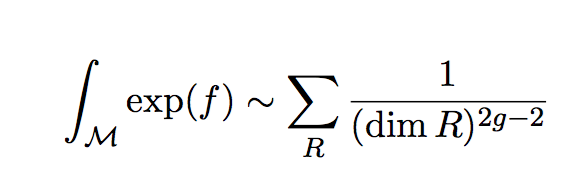
where we sum over irreducible representations $R$ of $G$. In the formulas, the symbol $∼$ means that the left hand side is proportional to the right hand side by a known proportionality constant.
In the special case of SU(2), we have
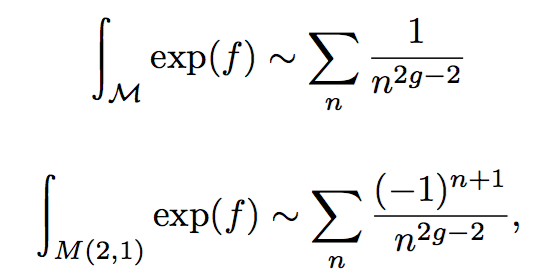
where we sum over the irreducible representations of SU(2), which are parametrized by their dimensions n. In this case, the Witten zeta function reduces to the Riemann zeta function.
Question:
Is it correct to say the known proportionality constant is given by Reidemeister–Ray–Singer torsion? Is it correct to say the symplectic volume forms is also given by Reidemeister–Ray–Singer torsion? How is that mathematically rigorous derived?
For SU(2), $$ \text{Witten zeta function = Riemann zeta function} $$ What is the analogy for U(1)? What is the analogy for SU($N$)? $$ \text{Witten zeta function for U(1) or SU($N$) = ?} $$
What is the simple intuition behind based on "the asymptotics of the Verlinde formula" to obtain Witten zeta function (in the Ref below)?
Ref: E. Witten, ‘On quantum gauge theories in two dimensions’, Commun. Math. Phys. 141 (1991) 153–209.
