0
$\begingroup$

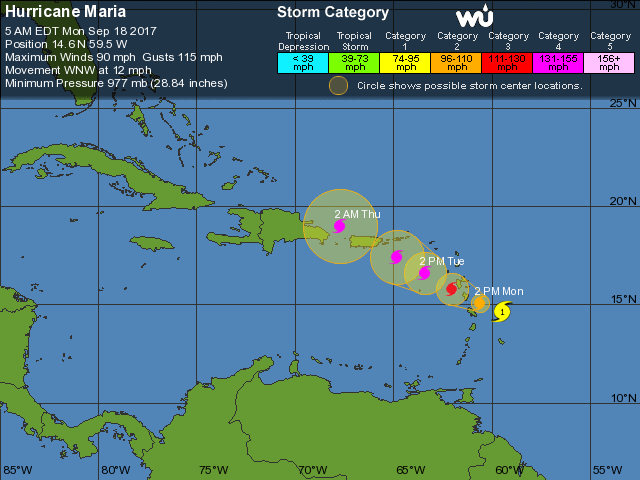
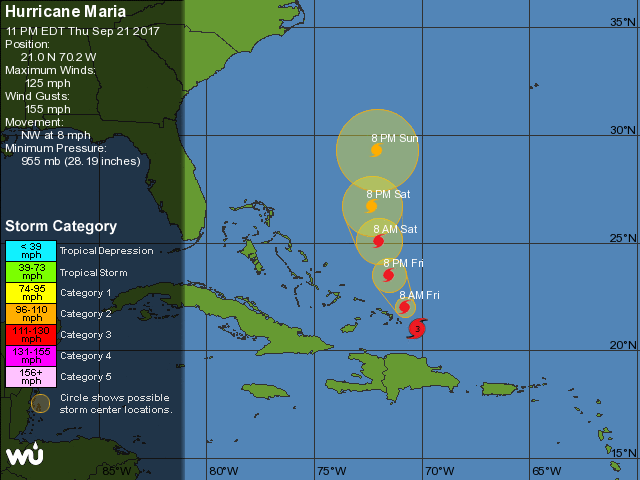
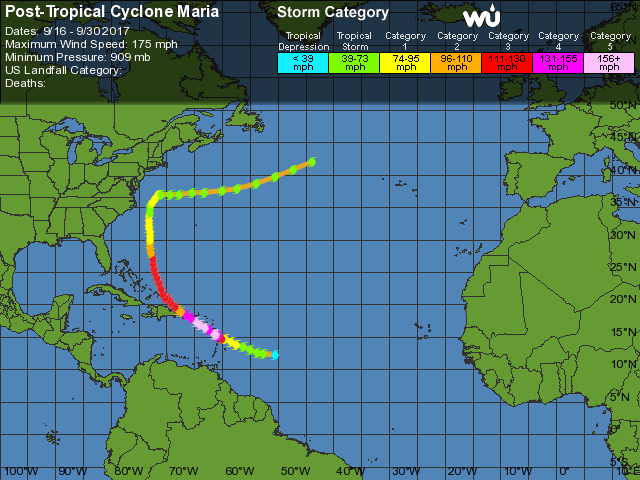
While I was watching the news last month I realized the weather report was basically a discussion of solutions to PDE. In particular, I was paying attention to the hurricane season (which is not yet over) and the case of tropical cyclones.

  • all weather is a solution to a partial differential equation. which one is used in weather prediction? This is likely to get very technical I was able to find several models:

    ECMWF, GFS, GFDL, UKMET, HWRF, NOGAPS etc. (as discussed here)

  • In a sense I don't really care what the actual PDE just the general shape and qualitative features.

    • We are studying the time evolution of some partial differential equation on the two-sphere: $\phi_t:S^2 \to S^2 $ which could be model by some type of random flow
    • Observing vortex solutions and in particular we are interested in the location of the center, a "radius" metric of some kind, and a measure of the vorticity (which is a kind of index).
    • Locally all hurricanes look about the same. Our prediction is going to be the Minkowski sum of a path and a circle of growing radius: $$ \phi_t \approx \phi_0(t) + t \, S^1 $$ This flow is mildly chaotic as we could predict the flow from one day to the next, but not over weeks or months.

These predictions took hours to achieve by clusters of computers. What enables us to approximate solutions to PDE in this common-sense way without actually solving anything?

9/18

enter image description here

9/25

enter image description here

Final

enter image description here

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ Why did you tag "dg" and "gt" and not "ap"? And I don't understand your question "What enables us to approximate solutions to PDE in this common-sense way without actually solving anything?" at all. What do you mean by "without actually solving anything?" and by "approximate solutions ... in this common-sense way"? Isn't your question answered by the fact that fluid dynamics have locally well-posed initial value problems? $\endgroup$
    – Willie Wong
    Commented Oct 9, 2017 at 17:12
  • $\begingroup$ The fluid in this case is "air". I have never taken a formal PDE course so this could likely have an elementary answer. However, I ask you if well-posed PDE can be discussed using convex geometry. In certain very simple equations, such as free particle, the time-evolution really is the Minkowski sum of a straight line and a cone in spacetime. @Williewong $\endgroup$
    – john mangual
    Commented Oct 9, 2017 at 17:36
  • $\begingroup$ I still don't understand your question. Suppose you have a smooth function $f$ defined on $[0,1]\times \Sigma$ where $\Sigma$ is a compact smooth manifold. If you further know that at $t = 0$, the inverse image of $[1,\infty)$ under $f$ is compactly included in some open subset $\Omega\subset \Sigma$, then by continuity there exists some $T$ such that for every $t\in [0,T]$ the inverse image of $[1,\infty)$ is contained in $\Omega$. This is independent of solving any PDEs. (Wellposedness gives you that regular initial data gives regular solutions so the above applies.) $\endgroup$
    – Willie Wong
    Commented Oct 9, 2017 at 19:19
  • $\begingroup$ What does "regular" mean in this context? I guess smooth or $C^1$ or whatever we like. Even better question, if $T$ is finite how can you have an inverse image $f^{-1}$? Because you have $[0,T]$ but also $ [0, \infty] $ and yet $ \Sigma $ is compact. Is $[0, \infty] \subset \Sigma $ ? Back to my original question, we don't quite know the initial value, or the PDE or the evolution operator or the final solution but we can guess the vortex should move along some path and the radius of uncertainty should get wider with time. $\endgroup$
    – john mangual
    Commented Oct 9, 2017 at 19:29
  • $\begingroup$ Think of $f:[0,1] \times \Sigma \to \mathbb{R}$; then $f^{-1}[1,\infty)$ is a set in $[0,1]\times \Sigma$. You can look at its sections at constant $t$. // Uncertainties in initial value is taken care of by the fact that wellposedness includes "continuous dependence of solution on initial data" as one of the key ideas. If you really are just asking about why qualitative features seem to persist over small periods of time with some sort of uncertainty propagation, then it really is just that the equations are provably locally well-posed. $\endgroup$
    – Willie Wong
    Commented Oct 9, 2017 at 21:16

0

Reset to default

You must log in to answer this question.