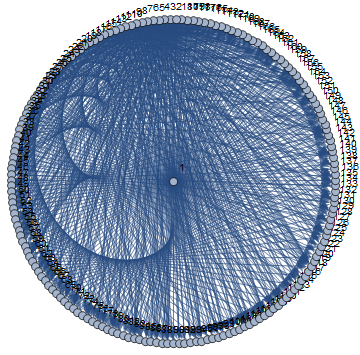
This is a fuzzier follow-up to this question. Again, we construct the graph whose vertices are integers from $1$ to $n,$ and two vertices are connected whenever one of the corresponding integers divides the other, and then we lay the graph out radially, so the vertex corresponding to $1$ is at the center of the circle, and the others are clockwise around the circumference This is what we get (for $n=180$). There are obviously patterns, but how do we explain them?
1 Answer
$\begingroup$
$\endgroup$
2
These curves are arcs of epicycloids. In particular, the one tangent to the vertical line is half of the cardioid. If, for a positive integer $k$, you draw a line from $\alpha$ to $k\alpha$ modulo $2\pi$ for all $\alpha$, the envelope will be the complete epicycloid. (For $k$ negative, you get hypocycloids.)
This observation is due to Luigi Cremona.
-
$\begingroup$ What's the reference for the Cremona attribution? $\endgroup$ Commented Sep 13, 2017 at 2:05
-
$\begingroup$ Unfortunately I don't have a reference. I found the attribution on websites. $\endgroup$ Commented Sep 13, 2017 at 5:36