7
$\begingroup$

Given a simple closed curve in the plane, there is a homeomorphism from the unit open disk to the interior of the curve. The homeomorphism can be taken conformal, this is the uniformisation theorem.

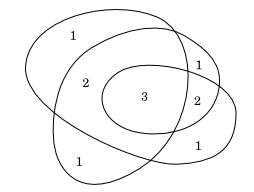
Is there a version of this result for closed curves that are not simple?

For example, given a $C^1$ closed curve $\gamma: S^1 \rightarrow {\bf R}^2$ with finitely many self-intersections, all of them transverse, is there a continuous map (maybe even conformal) from the unit open disk to the plane, such that the number of preimages of any point in ${\bf R}^2\backslash \gamma(S^1)$ is equal to the absolute value of the number of turns the curve makes around the point?

$\hskip 1in$ non simple closed curve

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ What would such a map look like for the figure-8 curve? $\endgroup$
    – Mikhail Katz
    Commented Jul 6, 2017 at 14:03
  • $\begingroup$ @Katz Twist the disk in three space, project it on a plane. So there is a line dividing the disk that projects exactly on the double point. Perhaps asking for all the number of turns to be of the same sign is a necessary condition for a conformal map. $\endgroup$
    – coudy
    Commented Jul 6, 2017 at 14:11
  • 1
    $\begingroup$ @coudy: The map you describe in the comment is not conformal, and not even open. Of course there is always a continuous map: a continuous map of a circle extends continuously in the disk. So what are you really asking? $\endgroup$
    – Alexandre Eremenko
    Commented Jul 6, 2017 at 20:49
  • $\begingroup$ @Eremenko. An arbitrary extension will not necessarily give the expected multiplicity. Read again the question and look at the diagram. $\endgroup$
    – coudy
    Commented Jul 7, 2017 at 6:19
  • 1
    $\begingroup$ @goette. The question is the following. Given a C1 closed curve γ:S1→R2 with finitely many self-intersections, all of them transverse, is there a continuous map (maybe even conformal) from the unit open disk to the plane, such that the number of preimages of any point in R2∖γ(S1) is equal to the absolute value of the number of turns the curve makes around the point? $\endgroup$
    – coudy
    Commented Jul 7, 2017 at 19:50

1 Answer 1

Reset to default
3
$\begingroup$

It sounds like, to some extent, what you are asking is whether an immersed closed curve in the plane can be extended to an immersion of a disk. This would yield the multiplicities that you seek; however, such an extension is not always possible. Necessary and sufficient conditions were first given in the thesis of Blank in 1967. For instance see this paper for more on this topic and references.

Improve this answer
$\endgroup$
1

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .