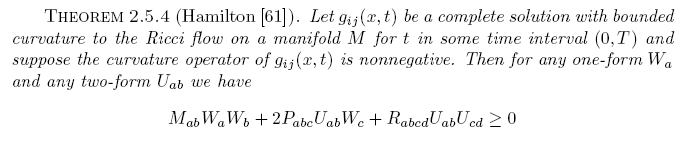This question is from my question on mathematics.
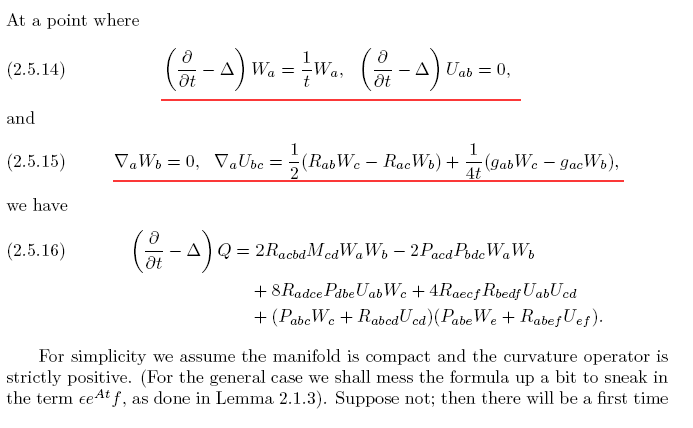
Picture below is from 231 page . For to prove $Q\ge 0$ on $M \times (0,T)$, $(\partial_t -\Delta)Q \ge 0$ and $Q\ge 0$ are needed to prove.But I can't see $Q\ge 0$ when $t=0$.
Besides, how to ensure $$ \left\{ \begin{aligned} & (\partial_t -\Delta) W_a =\frac{1}{t}W_a \\ & \nabla_aW_b=0 \end{aligned} \right.~~~~~~~~~~~~~ \left\{ \begin{aligned} & (\partial_t-\Delta)U_{ab} = 0 \\ & \nabla_aU_{bc} = \frac{1}{2}(R_{ab}W_c-R_{ac}W_b)+\frac{1}{4t}(g_{ab}W_c-g_{ac}W_b) \end{aligned} \right. $$ have solutions ? And $W_a$ and $U_{ab}$ are arbitrary , how to explain it ? (It is enough that proving the basis meets the equations ?)
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~