Thales' semicircle theorem in higher dimensions

Question

Thales semicircle theorem says that an angle inscribed in a semicircle is a right angle.

Q1. Does a cone with apex on a hemisphere and encompassing the circular base have a solid angle independent of the position of the apex?

It appears it might be true, with solid angle $(2-\sqrt{2})\pi \approx 0.59 \pi$ steradians:

---

 

---

Q2. I am seeking a proof or reference for the generalization to $d$ dimensions.

I have not found a reference, which makes me wonder if the answer to Q1 might be No...

---

Update. Thanks to TMA and @WillSawin for their analyses. Following Will, let $p=(\cos \phi, 0, \sin \phi)$ be the apex on the cone on the sphere; so $\phi=90^\circ$ places $p$ at the north pole. Below is a crude depiction of the intersection of the cone with the small green $p$-centered sphere. $\phi=(90^\circ,30^\circ,5^\circ)$ left to right:
 
And here are two enlarged views of the $\phi=5^\circ$ intersection:
 
The area of the (blue) spherical polygon is the solid angle. As Will argues, its area approaches $\frac{1}{4} (4 \pi)=\pi$ as $\phi \to 0$, so the answer to Q1 is No.

Answer 1

I think the limit of the red cone as the green point approaches the base is a quadrant, in agreement with The Masked Avenger's earlier impressions but not his later ones.

Imagine viewing the circle $(\cos \theta, \sin \theta, 0)$ from the point $(\cos \phi, 0, \sin \phi)$. What does the projection of the circle onto our field of view look like? To find out let's subtract the two points, getting:

$$(\cos \theta - \cos \phi, \sin \theta, - \sin \phi) $$

Now set $\theta = c \phi$ for some constant $c$ as $\phi$ goes to $0$. We have:

$$(\cos \theta - \cos \phi, \sin \theta, - \sin \phi) = (O( \phi^2), c \phi + O (\phi^3) , - \phi + O (\phi^3))$$

If we project onto the sphere by dividing by the length, this will be:

$$(o(1) , \frac{c}{\sqrt{c^2+1} }+ o(1), \frac{-1}{\sqrt{c^2+1} }+o(1))$$

So as $\phi$ goes to $0$ this converges to an arbitrary point on the circular arc $(0,y,z)$ with $y^2+z^2=1$ and $z<0$.

On the other hand, if you look at a fixed point $(\cos \theta, \sin \theta, 0)$ on the circle, as $\phi$ goes to $0$ the viewing direction will converge to $(\cos \theta-1, \sin \theta, 0)$. After normalizing, this gives us the circular arc $(x,y,0)$ with $x^2+y^2=1$ and $x<0$.

So the circle, viewed on the unit sphere, converges to these two circular arcs. The view of the disc must contain the quadrant $x<0, z<0$ bounded by the two arcs. It is easy to see that no other points will be in the limit. So the limit area is the area of a quadrant, or $\pi$ steradians. Since this is not the measured area at the top of the sphere, I think this shows that the answer to Q1 is "no".

Answer 2

There are several articles on estimating the solid angle of a circular disk in the physics literature. See the articles cited by the Wikipedia article on solid angles, including Gardner, R. P.; Verghese, K. (1971). "On the solid angle subtended by a circular disk." Nucl. Instr. Meth. 93 (1). pp. 163–167. That paper uses cones over approximating polygons with the same area as the circle to approximate the solid angle. Other papers mention expressing the solid angle in terms of elliptic integrals, which seems to have been considered well-known in the 1950s but not ideal for producing numerical approximations at that time. However, modern CASs can handle worse numerical integrals.

Let the point on the hemisphere be $P$. Let the equatorial disk be $D$.

Consider the right circular cones whose apex is $P$ whose axes are perpendicular to the plane of $D$. The solid angle of a cone with apex angle $2\phi$ is $2\pi (1-\cos \phi)$. The derivative of this is $2 \pi \sin \phi$. Consider the intersection of the surface of the right circular cone of apex angle $2\phi$ with the cone over $D$. This is a cone over an arc of angle $f(\phi)$ radians. The solid angle of the cone over $D$ is thus

$$\int f(\phi) \sin \phi d \phi.$$

Another way to state that is to compute the solid angle in spherical coordinates centered at $P$, which I why I used $\phi$ to denote half of the apex angle.

For $\phi \le \phi_0$, $f(\phi) = 2 \pi$, and for $\phi \ge \phi_1$, $f(\phi)=0$. Between these, we want the angle of the intersection of a circle with $D$ so that the circle intersects the boundary of $D$.

Let the distance between the perpendicular bisector and the center of $D$ be $a$. Let the distance between $P$ and the plane of $D$ be $h$. The circle $C$ has radius $r = h \tan \phi$ and is centered at a point of distance $a$ from the center of $D$. The intersection between $C$ and the boundary of $D$ occurs at angle $\theta = f(\phi)/2$ where

$$\begin{eqnarray} (r \sin \theta )^2 + (r \cos \theta - a)^2 &=& 1 \newline -2ra \cos \theta &=& 1-a^2-r^2 \newline \theta &=& \arccos \left( \frac{1-a^2-r^2}{-2ra} \right) \newline f(\phi) &=& 2 \arccos \left( \frac{1-a^2-r^2}{-2ra} \right) \newline f(\phi) &=& 2 \arccos \left( \frac{1 -a^2 - h^2 \tan^2 \phi}{-2 h a \tan \phi} \right).\end{eqnarray}$$

$\phi_0$ is the value of $\phi$ at which $C$ starts to intersect the boundary of $D$, when $a+r=1$, $a+h \tan \phi_0 = 1$, so $\phi_0 = \arctan \frac{1-a}{h}$. Similarly, $\phi_1$ satisfies $r-a=1$ so $\phi_1 = \arctan \frac{1+a}{h}$.

So, the solid angle is

$$\begin{eqnarray}\int_0^{\phi_1} f(\phi) \sin \phi d \phi =& 2\pi\left(1-\cos \arctan \left((1-a)/h\right)\right) \newline &+ \int_{\arctan (1-a)/h}^{\arctan(1+a)/h} 2 \arccos \left( \frac{1 -a^2 - h^2 \tan^2 \phi}{-2 h a \tan \phi} \right) \sin \phi d \phi.\end{eqnarray}$$

This can be simplified slightly, for example, $\cos \arctan x = \frac{1}{\sqrt{1+x^2}},$ but this can be used in a CAS. For example, we can choose $h=3/5, a=4/5$, and Mathematica reports that the solid angle is about $2.11602$, which is greater than $(2-\sqrt 2)\pi = 1.84033.$ Here is a plot from Mathematica of the solid angles as a function of the displacement from the axis $a$:

So, numerical calculations indicate that this is not constant.

Answer 3

Thales' semicircle theorem is of course a special case of the inscribed angle theorem. Let's consider a 3-dimensional analogue of the inscribed angle theorem, not just the semicircle theorem.

In the 2-dimensional case, we are looking at an angle inscribed in a circle. In 3 dimensions, what we are looking at is an oblique circular cone inscribed in a sphere. The thing is, an oblique circular cone is just a right elliptical cone cut at a slant. For our purposes, the 3-dimensional analogue of an angle is an (infinite) elliptical cone.

(You've probably seen how you can obtain an ellipse by intersecting a right circular cone with a plane - that is why they're called conic sections, after all. Cutting an elliptical cone also results in conic sections. And if you do it in just the right way, you may get a circle.)

Let's make this more explicit. Consider the oblique circular cone whose base is the origin-centered circle of radius $r$ in the $xy$-plane, and whose apex is $(x_0,y_0,z_0)$. An equation for [the infinite double cone containing] this cone is $$ \left(x-\frac{x_0 z}{z_0}\right)^2 + \left(y-\frac{y_0 z}{z_0}\right)^2 = \left(r\left(1-\frac{z}{z_0}\right)\right)^2. $$ (Check that this correctly describes the cone.) For later use, let's generalize slightly to allow the base circle to be centered at $(0,0,z_1)$, still parallel to the $xy$-plane. The equation becomes $$ \left(x - x_0 \cdot \frac{z-z_1}{z_0-z_1}\right)^2 + \left(y - y_0 \cdot \frac{z-z_1}{z_0-z_1}\right)^2 = \left(r\cdot\frac{z_0-z}{z_0-z_1}\right)^2 $$ (i.e. just replace $z$ with $z-z_1$ and $z_0$ with $z_0-z_1$). In any case, we have a quadratic equation in 3 variables. By changing to some suitable coordinates $u,v,w$, we'll be able to put this in the standard form $$ \frac{u^2}{a^2} + \frac{v^2}{b^2} = w^2 $$ for an elliptical cone. Here, we'll call $a$ and $b$ the "semi-major and semi-minor parameters", depending on which is greater.

We now return to the picture of an oblique circular cone inscribed in the unit sphere. Let the apex be $(x_0,y_0,z_0)=(\sin\sigma,0,\cos\sigma)$ (i.e. its inclination angle to the zenith is $\sigma$, i.e. latitude $\pi/2 - \sigma$). We are assuming without loss of generality that the apex has $y$-coordinate $0$. Let the base be the circle with radius $r=\sin\tau$ centered at $(0,0,\cos\tau)$ parallel to the $xy$-plane (i.e. the circle at inclination $\tau$, i.e. latitude $\pi/2 - \tau$). We'll assume that $0 \le \sigma \lt \tau \lt \pi$. This figure illustrates the setup:

By the formula above, an equation for the cone is $$ \left(x - \sin\sigma \cdot \frac{z-\cos\tau}{\cos\sigma-\cos\tau}\right)^2 + y^2 = \left(\sin\tau\cdot\frac{\cos\sigma-z}{\cos\sigma-\cos\tau}\right)^2. $$ As a first step, change coordinates by \begin{align} x' &= x-\sin\sigma \\ y' &= y \\ z' &= z-\cos\sigma \end{align} so that the apex becomes $(0,0,0)$ in $x',y',z'$-coordinates. In the new coordinates, the equation for the cone is \begin{align} \left(x' - \frac{\sin\sigma}{\cos\sigma-\cos\tau} \cdot z'\right)^2 + (y')^2 &= \left(\frac{\sin\tau}{\cos\sigma-\cos\tau} \cdot z'\right)^2 \\ ((\cos\sigma-\cos\tau)x' - (\sin\sigma)z')^2 + ((\cos\sigma-\cos\tau)y')^2 &= ((\sin\tau)z')^2 \end{align} Now establish a rotated coordinate system $u,v,w$, so that the $x',y',z'$ and $u,v,w$ coordinates of a point are related by \begin{align} x' &= \left(\cos\frac{\sigma}{2}\right) u + \left(\sin\frac{\sigma}{2}\right) w \\ y' &= v \\ z' &= \left(-\sin\frac{\sigma}{2}\right) u + \left(\cos\frac{\sigma}{2}\right) w. \end{align} After a lot of manipulation, we end up with $$ \frac{u^2}{\frac{\cos^2(\tau/2)}{\sin^2(\tau/2)}} + \frac{v^2}{\frac{\cos^2(\tau/2)}{\sin^2(\tau/2) - \sin^2(\sigma/2)}} = w^2. $$

So our conclusions are:

• For our purposes, the 3-dimensional analogue of an angle is an (infinite) elliptical cone.
• An "angle" inscribed in a sphere, as parametrized by $0 \le \sigma \lt \tau \lt \pi$ above, is an elliptical cone with "semi-minor parameter" $$ \frac{\cos(\tau/2)}{\sin(\tau/2)} $$ and "semi-major parameter" $$ \frac{\cos(\tau/2)}{\sqrt{\sin^2(\tau/2) - \sin^2(\sigma/2)}}. $$
• Two "angles" inscribed in a sphere which subtend the same circular base have the same semi-minor parameter.
• Given two "angles" inscribed in a sphere which subtend the same circular base, the one with the larger $\sigma$ has the greater semi-major parameter.
• In particular, when the $\tau$'s are the same, the one with the larger $\sigma$ has the greater solid angle.

The fact that the "semi-minor parameter" is the invariant is actually not that surprising. Let $A=(0,0,-1),B=(\sin\sigma,0,\cos\sigma),C=(\sin\tau,0,\cos\tau)$. Then angle $ABC$ is $\tau/2$ (see the figure); this is essentially just the 2-dimensional inscribed angle theorem. It's sensible that $\cot(\tau/2)$ is one of the parameters of the elliptical cone.

Answer 4

ad Q1. It is a simple, if tedious, elementary exercise to compute the solid angle subtended at a point in space by a figure in the $x$, $y$ plane as a function of its coordinates. One places the point in a small ball and computes the surface area of the stereoraphic projection of the figure on the corresponding sphere. This provides a scalar function whose level surfaces give an answer to Q1 in quite a general situation. Of course, the computation of this function involves double integrals over the given figure and it will depend on the latter whether this can be done explicitly.

Added as an edit. The corresponding two dimensional problem was very popular in classical differential geometry. An isoptic curve (other names---equioptic, isogonal) to a figure (not necessarily one dimensional) is the set of points from which the figure subtends the same angle (not necessarily a right angle). An interesting question is when these curves are equipotentials, i.e., the level curves of a harmonic function. This is not the same as asking whether the function described above is harmonic. This question is motivated by the fact that this is indeed the case for some of those few cases where the isoptics have been computed (e.g., the isoptics of an ellipse).

Answer 5

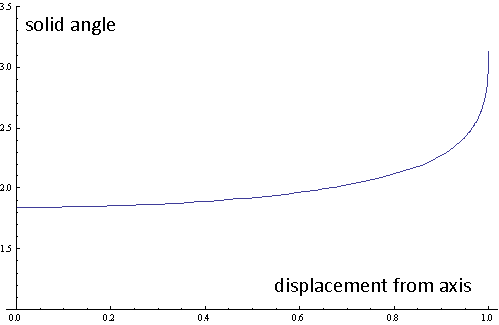
Having seen the analyses presented in the other answers and Douglas Zare's comment about difficulties in visualization, I offer a view on the problem that shows how to arrive at a qualitative result (the answer to Q1 is no), and explains my confusion (expressed in a comment to Will Sawin) on why I thought a limiting value was pi steradians, then backpedaled and said it was NOT pi steradians.  (The computations of Will and Douglas and further consideration support the guess I had initially: the solid angle eventually grows to pi steradians when the observer moves close to the disk.)

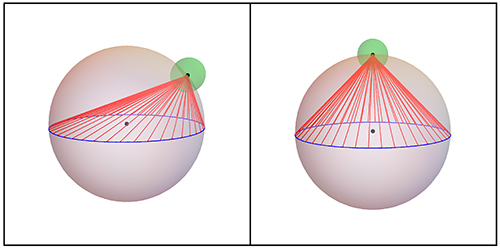
I initially thought of the pictures that Joseph presented as unintentionally misleading: the question asks about a projection onto a hemisphere, but not onto the hemisphere on which the observer is located, which is the prominent figure in Joseph's pictures.  One has to suppress Joseph's initial illustration and replace it by one where the observer is the center of a different hemisphere; the original base is now an object to be projected on this hemisphere representing the visual field of the observer.  I call this new hemisphere the "visible hemisphere".

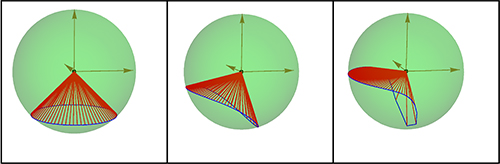
I thought first on possible projections of the observed disk onto the visible hemisphere. It seemed that the projections would get larger primarily because one got closer to the disk edge.  In fact, the disk would "block" about half the field of vision, so that would suggest a solid angle of close to pi steradians.  However, when one is at the edge, one sees the disk edge on, and projects no such shadow.  I also thought that the shape would resemble (but not match) that of an elongated disk approximated by an ellipse, which approached a wedge shape that covered half of the visible hemisphere.

I then tried a different model, which I called a "flap model".  I chose half a disk, with its diameter oriented up and down, fixed that diameter to the apex of the visible hemisphere, and observed that the (appearance of the) diameter stayed constant (thanks to Thales), and so I always had part of the observed disk stay at the same apparent position as the observer traveled.  Taking the observer's position, I moved part of the disk like a flap hinged at this diameter, and imagined that this portion grew larger as it swung closer to the observer (almost hitting the observer on their nose), but because it was a circular flap, some significant portion of the half of the hemisphere might still be visible, and also, the other half of the observed disk formed another flap that swung away from the observer and got smaller.  In particular, this set up the image in my mind that I had trouble resolving with my initial thought: if the image is a projected ellipse, why is the diameter of the disk not a diameter of the projection?  Since I couldn't immediately resolve this, I commented to Will Sawin about my "certainty" that the projection did not approach pi steradians in measure.

The confusion arises from common notions of perspective.  (I hope Joseph takes his cue and provides some illustrations.) When one takes a rectangular region at some distance from an observer, say the face of a digital clock, and rotates it around an axis of symmetry parallel to the shorter edge of the rectangle, one gets a trapezoidal shape as a projection.  (When projected to the visible hemisphere, it is a different shape, but the curves are not noticed by the observer.)  If one does not have a reference line though, one assumes the axis of symmetry is placed equidistant from the bases of the apparent trapezoid.  When the rectangle is far away, the actual axis is not far from the imagined axis, and so the assumption seems to hold, at least for government purposes.  But in fact, the actual axis is NOT equidstant from the line equidistant from the parallel bases of the trapezoid, and in order to see this, one has to perform some calculations or some very good observations.

The disparity for circles is even less apparent: we approximate the image of a rotated disk by an ellipse.  The projection however is not an ellipse, and in certain extreme cases the approximation is poor.  It was such an approximation that misled me to thinking that one could not get close to pi steradians in this situation.

I finally did mentally what I should have done in real-life:  I imagined holding an analog clock close to my face, and turning it until it almost hit my virtual nose.  I imagined the number 12 right above the number 6, and in an elliptical projection or in a scenario where the clock is (not very) far away, the 11 and 7 are closer to me but still appear closer to each other than the 12 does to the 6.  However, when the clock is about to hit my face, the 11 and 7 appear farther away than the 12 and the 6.

That the 11 and 7 will appear farther away makes sense when one places one's eye very close to the 9, and draws the lines of sight from the eye to the 12 and 6, and finds these lines run between the 11 and 7.  However, and this is the key to this posting, one does not think of this image from the initial setup of Joseph's problem.

Once one has the insight that the analog clock image does distort severely even from such simple, common, and everday projective transformations, then resolution occurs: the clock does not elongate in a somewhat elliptical fashion to occupy a quadrant, instead it distorts in a way which fills the quadrant in a nonintuitive fashion not easily described.  The equations in the other postings are a testament to the complexity of the descriptions of the resulting images.

While I hope that the post is clear about describing a resolution to the question, I think it more important to describe that a natural assumption about how an object appears should be subject to question, and that tests, not just analysis, of the extreme limits of such assumptions need to be made.  To borrow from another MO post, multiple perspectives are very useful for resolving this problem.

Answer 6

This ia modest, marginally on topic comment which I am adding in the hope that it might be of assistance. The OP interprets the Thales theorem as identifying the locus of points in a plane at which a given segment subtends the same angle (specifically a right angle) and considers the three dimensional analogue for the solid angle subtended by a disc. One could, however, take the point of view that we are given the base points of a triangle and consider the locus of the apex of the triangle under the condition that the angle there be constant, in particular a right angle. The natural generalisation is to consider a given triangle $ABC$ in space and the locus of points $P$ so that the solid angle of the tetrahedron $ABCP$ there is constant. This has the advantage that it is completely tractable.The result is rather intricate and this might give a clue as to why the original problem might not be expected to have a simple solution, certainly nothing as simple as a spherical surface.

A simple computation shows that the loci of such points are, for a suitable choice of coordinates, the level sets of the function $\frac{qz}{f(x,y,z)}$ where $$f(x,y,z)=(-qy+y^2+z^2)(a+b)+(y^2+z^2)c + abc+x(a+c)+x^2(a+b+c)+p(a-x(a+b)).$$ Here $p$ and $q$ are parameters which depend on the shape of the triangle and $a$, $b$ and $c$ are the distances of $P$ to its vertices, in our coordinates, $a=(x^2+y^2)^{1/2}$, $b=(x^2+(y-1)^2)^{1/2}$, $a=((x-p)^2+(y-q)^2)^{1/2}$.