3
$\begingroup$

Suppose one place $n$ electrons closely surrounding the north pole of a sphere, forming a perfect planar regular $n$-gon:

         

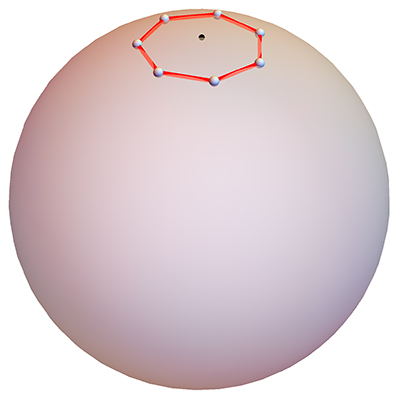
Q1. What will happen if the electrons repel one another via Coulomb's law, but are confined to the surface? Will they maintain a planar regular $n$-gon configuration? With damping will they settle on the equator?

I believe the answer to the latter two questions is Yes, but I did not attempt a formal proof. Here is my primary interest:

Q2. Suppose small random errors are introduced so that the initial configuration is not an exact planar regular $n$-gon. Will the electrons on average head toward the expected minimum energy configuration?

For $n{=}7$ illustrated above, that min configuration is conjectured to be an equatorial regular pentagon with electrons occupying the two poles. Perhaps especially with larger $n$, there are many local minima rendering it unlikely to settle in the min-energy configuration?

Improve this question
$\endgroup$
7
  • 5
    $\begingroup$ The answer to Q1 must be Yes, because the differential equation has a unique solution so it must retain the initial symmetry. For Q2 the particles must approach a stable local minimum, but in general they don't have to find a global minimum, and the probability of success might depend also on the strength of the damping. (Is a regular $n$-gon on the equator locally stable once $n>3$?) $\endgroup$
    – Noam D. Elkies
    Commented Mar 18, 2015 at 1:25
  • 2
    $\begingroup$ I did a Matlab computation which showed that for $n>4$ the regular $n$-gon on the equator is locally unstable. m=20; f3=zeros(1,m); for n=2:m ind=(0:n-1)*2*pi/n; x=[cos(ind);sin(ind);zeros(1,n)]; eps=10^(-4); y=[cos(eps);0;sin(eps)]; %force F=zeros(3,1); for i=2:n xi=x(:,i); F=F+(y-xi)/norm(y-xi)^3; end %projection F=F-(F'*y)*y; f3(n)=F(3)/eps; end f3 $\endgroup$
    – user35593
    Commented Mar 18, 2015 at 11:30
  • 1
    $\begingroup$ @NoamD.Elkies: The answer to Q1 must be Yes, because the differential equation has a unique solution so it must retain the initial symmetry. I don't think this really constitutes a proof, since there are counterexamples such as the famous Norton's dome: en.wikipedia.org/wiki/Norton%27s_dome . The problem is basically that solutions to the equations of motion in Newtonian mechanics may be nonunique, i.e., Newtonian mechanics is not actually deterministic in all cases. $\endgroup$
    – user21349
    Commented Sep 17, 2015 at 16:25
  • 1
    $\begingroup$ This seems closely analogous to packing of hard spheres, where it is known that random packing is always worse than the optimal packing. So I think it's very unlikely that such a system achieves the global minimum in all cases. $\endgroup$
    – user21349
    Commented Sep 17, 2015 at 16:27
  • 1
    $\begingroup$ @BenCrowell non-unique solutions can happen but only when the system starts at a singularity or reaches a singularity. Here the electrons start in different (albeit close) positions, and they can never crash into each other because the exert repulsive forces on each other that blow up as $r \to 0$. $\endgroup$
    – Noam D. Elkies
    Commented Sep 17, 2015 at 18:01

0

Reset to default

You must log in to answer this question.