21
$\begingroup$

Let a positive convex decreasing differentiable function $f(x)$ be defined on $\mathbb{R}$ and $\lim_{x \to +\infty}f(x)=0.$ Let the point light source be placed at $ P(x_0,y_0)$ with $ y_0>0,\,y_0 <f(x_0).$ Light is assumed to be reflected from the plot $y=f(x)$ and the $x$-axis. Does there exist a number $R$ s.t. the part of the graph $y=f(x)$ for $x>R$ is not lightened?

The model example $f(x):=e^{-x},\,P(0,0.5)$ suggests the answer is yes.

The question is migrated from SE.

Improve this question
$\endgroup$
6
  • 5
    $\begingroup$ By "migrated", you mean "crossposted". The problem is still there on m.se. $\endgroup$
    – Gerry Myerson
    Commented Feb 22, 2015 at 22:30
  • $\begingroup$ Gerry Myerson : You are not right. I asked that in SE. Having not obtained any feedback during 20 hours, I decided to repost that here. $\endgroup$
    – user64494
    Commented Feb 23, 2015 at 6:13
  • 5
    $\begingroup$ I believe that's the definition of crossposting. "Migrated" is a technical term in the stackexchange network, and is something that can only be done by moderators, and, when it is done, the question no longer appears at the original site. So, whatever you did, it's not migration. $\endgroup$
    – Gerry Myerson
    Commented Feb 23, 2015 at 6:30
  • 1
    $\begingroup$ Although I liked Bob's answer (and voted it), I doubt that it solves completely the question, because it deals with only one light ray. It proves that every light ray $R$ must bounce back at some abcissa $X(R)$. But it does not prove that $XR)$ is bounded independently on the initial direction of the ray. $\endgroup$
    – Denis Serre
    Commented Feb 23, 2015 at 9:28
  • 2
    $\begingroup$ I don't understand the replacement of $\mathbb{R}$ by $\mathbb{R}_+$ in my question. Please don't make such things. $\endgroup$
    – user64494
    Commented Feb 27, 2015 at 9:05

3 Answers 3

Reset to default
14
$\begingroup$

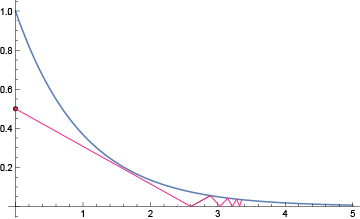
If a ray of light at angle $\alpha$ above the horizontal hits your curve $y = f(x)$ from below at a point where the tangent to the curve has angle $\beta$ below the horizontal, it will reflect at angle $\alpha + 2 \beta$ below the horizontal, and then come back up at $\alpha + 2 \beta$ above the horizontal. In particular, if $\alpha + 2 \beta = \pi/2$ it goes vertically down (and then retraces itself backwards), and if $\alpha + 2 \beta > \pi/2$ it goes backwards (i.e. to the left).

Let the $n$'th reflection on the curve take place at $(x_n, y_n)$, with incoming ray at angle $\alpha_n$. Then we have $$\eqalign{\alpha_{n+1} &= \alpha_n - 2 \arctan(f'(x_n))\cr y_{n+1} + y_n &= \tan(\alpha_{n+1}) (x_{n+1} - x_n)\cr y_{n+1} &= f(x_{n+1})}$$ Thus $$\dfrac{\Delta \alpha_n}{\Delta x_n} = \dfrac{\alpha_{n+1}-\alpha_n}{x_{n+1} - x_n} = \tan(\alpha_{n+1}) \dfrac{- 2 \arctan(f'(x_n))}{ f(x_{n+1}) + f(x_n)} $$ In order for $x_n \to \infty$ with $\alpha_n$ increasing but staying below $\pi/2$, we would certainly need this to go to $0$. In the case $f(x) = e^{-x}$, that certainly won't happen, as $\arctan(f'(x_n)) \approx f'(x_n) = - f(x_n)$, while $f(x_{n+1}) + f(x_n) < 2 f(x_n)$. More likely candidates would be functions $f$ that go to $0$ very slowly, perhaps something like $1/\log(x)$.

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Nice calculation! So, just to be clear, "that certainly won't happen" implies that $f(x)=e^{-x}$ has the property that $x_n$ stays bounded---Correct? $\endgroup$
    – Joseph O'Rourke
    Commented Feb 23, 2015 at 0:12
  • $\begingroup$ @ Robert Israel : I find that approach constructive. How about the general case? $\endgroup$
    – user64494
    Commented Feb 23, 2015 at 6:10
  • $\begingroup$ Your statement "In order for $x_n \to \infty$ with $\alpha_n$ increasing but staying below $\pi/2$, we would certainly need this to go to $0$." is not true in general, since we can have a sub-index-sequence $n_i$ where $x_{n_i+1}-x_{n_i}$ summing over $i$ to a finite positive number (fast) and $\dfrac{\alpha_{n_i+1}-\alpha_{n_i}}{x_{n_i+1} - x_{n_i}}=a$ for some positive $a$. However, we can conclude that $\liminf\limits_{n\to\infty}\dfrac{\alpha_{n+1}-\alpha_n}{x_{n+1} - x_n}=0$. It is impossible for the right hand side of the last equation which gives the desired result. Do you agree? $\endgroup$
    – Hans
    Commented Oct 1, 2019 at 22:47
  • $\begingroup$ +1. Neglected to say that this is a wonderful solution. But do you agree with my minor criticism above, Robert? $\endgroup$
    – Hans
    Commented Oct 2, 2019 at 15:21
6
$\begingroup$

Just empirically, I believe the OP's $e^{-x}$ example has the property that ray reflections quickly become increasingly vertical, and so will not reach arbitrarily large $x$:

          exconvex
(I did not, however, perform the calculations to prove this.)

Improve this answer
$\endgroup$
4
  • $\begingroup$ @ Joseph O'Rourke: Thank you for your interest to the question. $\endgroup$
    – user64494
    Commented Feb 22, 2015 at 21:38
  • 4
    $\begingroup$ Just as empirically, I believe that numerical calculations are consistent with a logarithmic divergence of the $n$th reflection abscissa. $\endgroup$
    – Eckhard
    Commented Feb 22, 2015 at 23:25
  • $\begingroup$ @Eckhard: Good! Then clearly we need calculations to settle the issue for $e^{-x}$. It seems clear to me that some function will prevent the rays from bouncing to $x=\infty$, even if $e^{-x}$ does not.... Oh, I see Robert Israel has weighed in with calculations. $\endgroup$
    – Joseph O'Rourke
    Commented Feb 22, 2015 at 23:43
  • $\begingroup$ The numerics are also consistent with $x_n$ being bounded, so I'll defer to Robert Israel. $\endgroup$
    – Eckhard
    Commented Feb 23, 2015 at 0:12
3
$\begingroup$

In the paper The existence of unbounded oscillating trajectories in a problem of billiards (1962) Leontovich proved that under bell-like curve (it must be zero at $\pm\infty$) each trajectory oscillates, i.e. it crosses y-axis infinitely often. Also he proved that among all trajectories do exist finite and infinite ones.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .