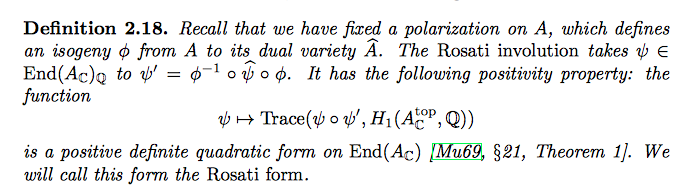2
$\begingroup$

I was reading a paper and they mentioned the Rosati form. Particularly, what they said was:

Let $A$ be an abelian surface defined over $k$ such that $ST_A^0$ (the connected component of the Sato-Tate group) $ = U(1) \times U(1)$. Then the matrices in $M_4(\mathbb{C})$ commuting with $U(1) \times U(1)$ are

$$\Bigg\{\begin{pmatrix}a&0&0&0\\0&b&0&0\\0&0&c&0\\0&0&0&d\end{pmatrix}: a,b,c,d\in\mathbb{C}\Bigg\}$$

and the $\textit{Rosati form}$ is a scalar multiple of $2ab+2cd = \frac{1}{2}((a+b)^2 - (a-b)^2 + (c+d)^2 - (c-d)^2)$.

I have done a Google search and can find nothing that references a Rosati form. I have found references to a Rosati involution. Is this the same thing? If not where could I find a definition of the Rosati form>

Improve this question
$\endgroup$
1
  • 5
    $\begingroup$ In case someone wants to take a look at the paper you were reading, you might want to give the name (and a link) of the paper. Providing such context can make answering easier. $\endgroup$
    – Joonas Ilmavirta
    Commented Sep 4, 2014 at 19:47

1 Answer 1

Reset to default
7
$\begingroup$

the Rosati form is defined in definition 2.18 of Sato-Tate distributions and Galois endomorphism modules in genus 2

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .