Surfaces that can be rolled by a ball

Question

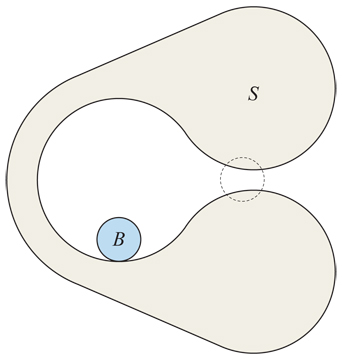
Let $S$ be a smooth solid body in $\mathbb{R}^3$, and $B$ a ball of radius $r$. Say that $B$ is in contact with $S$ if (1) they share a point $x$ that is on the surface of each, $x \in \partial S$ and $x \in \partial B$, and (2) neither penetrates the other, $\operatorname{int} S \cap \operatorname{int} B = \varnothing$. Say that $S$ can be rolled by $B$ if, for every pair of points $x,y$ on the surface of $S$, there is a path $\rho$ connecting $x$ to $y$ on $\partial S$ such that $B$ can be placed in contact at every point $z \in \rho$. In other words, the ball $B$ can roll between any pair of points of the surface without ever penetrating $S$.
          
My question is:

Characterize the bodies $S$ that can be rolled by a ball of radius $r$.

A necessary condition is that the Gaussian curvature at any point on the surface of $S$ must be $\ge -\frac{1}{r^2}$. Could that also be sufficient, or are there global obstructions such that $B$ could be in local contact but penetrate away from the contact point? Is the characterization different for surfaces of genus zero than for surfaces with handles? Any insights, speculations, or literature pointers would be appreciated. Thanks!

---

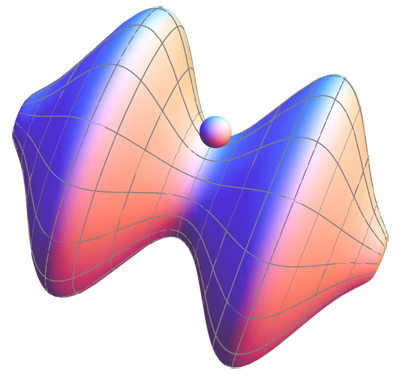
Update. Here is my interpretation of Anton's example:
          

Answer 1

You can call such bodies as "bodies of reach $\ge r$".

Clearly all the principle curvatures has to be $\ge -\tfrac1r$.

Plus you have a global condition which might be stated the following way: There is no segment between points on the surface which (1) meets the surface at normal direction at both ends (2) has length $<2\cdot r$ and (3) its interior lies in the complement of the body.

Answer 2

$S$ is the complement of the Minkowski sum${}^*$ of a connected body and the sphere of radius $r$.

---

${}^*$ Provided the Minkowski sum doesn't self-intersect.

Answer 3

1. Is not your condition equivalent to this: for every boundary point if the body, there exists a closed ball containing this point and whose interior does not intersect the interior of the body? (Of course I assume that your surface is connected). Or perhaps I did not understand what you mean by "rolling"? Why do you need two points, and a path?

2. If I understand your definition correctly, here is a reference where this notion is studied in dimension 2: http://arxiv.org/pdf/1204.4283.pdf They call such things r-convex.

Answer 4

The property you are asking for is called global radius of curvature. The notion combines the condition of lower bound on the principal curvatures as well as the global condition of no interpenetration of a neightborhood. This notion is very well adapted to the calculus of variations.

The heuristic definition from the paper of P. Strzelecki and H. von der Mosel: Global curvature for surfaces and area minimization under a thickness constraint

The main idea can be sketched as follows: Take a continuous parametric surface $X : \mathbb{R}^2\supset \mathbb{B}^2 \to \mathbb{R}^3$ (with possibly infinite area) which possesses a tangent plane on a dense subset $G \subset \mathbb{B}^2$ which may even have zero measure. Consider the radii of all spheres touching the surface $X(\mathbb{B}^2)$ in one of these points $X(\omega)$, $\omega \in G$, and containing at least one other point of the surface. We define the infimum of these radii as the global radius of curvature $\Delta[X]$ of the surface $X$ . It turns out that a positive lower bound on $\Delta[X]$ serves as an excluded volume constraint for the surface $X$.