How many roots do $\tan(z)-z^n = 0$, $\sin(z)-z^n=0, \ \cos(z)-z^n=0, $ have?

Question

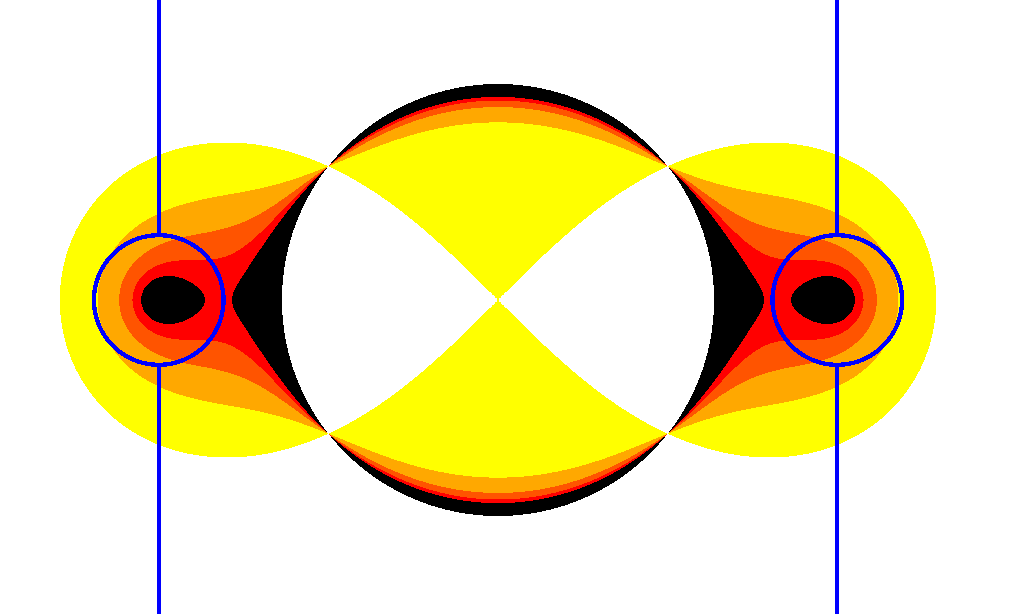
I asked this question on MSE here.

---

I am investigating the number of roots of the equations:

$$\tan(z) - z^n = 0$$ $$\sin(z)-z^n=0$$ $$\cos(z)- z^n=0$$

within the vertical strip $|\text{Re}(z)| \leq \frac{\pi}{2}$ for positive integers $n$. Numerical computations suggest interesting behavior:

---

$$\tan(z)-z^n=0$$

• The equation $\tan(z) - z = 0$ has only the trivial solution $z = 0$.
• For $n \ge 2$, the number of roots appears to depend on the value of $n$. Plots for some cases are shown below:

$\tan(z)-z$:

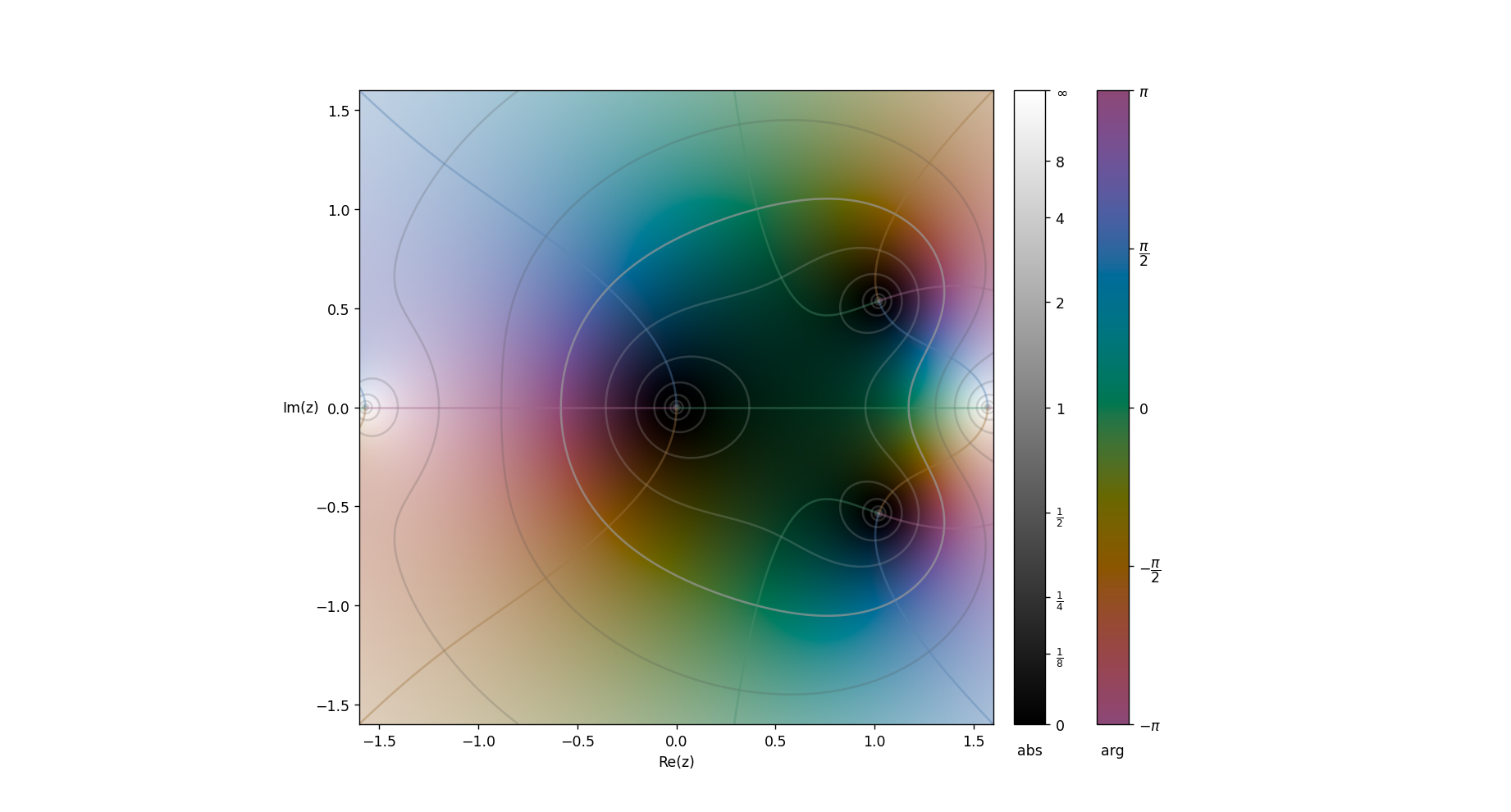
$\tan(z)-z^2$ (showing 3 roots):

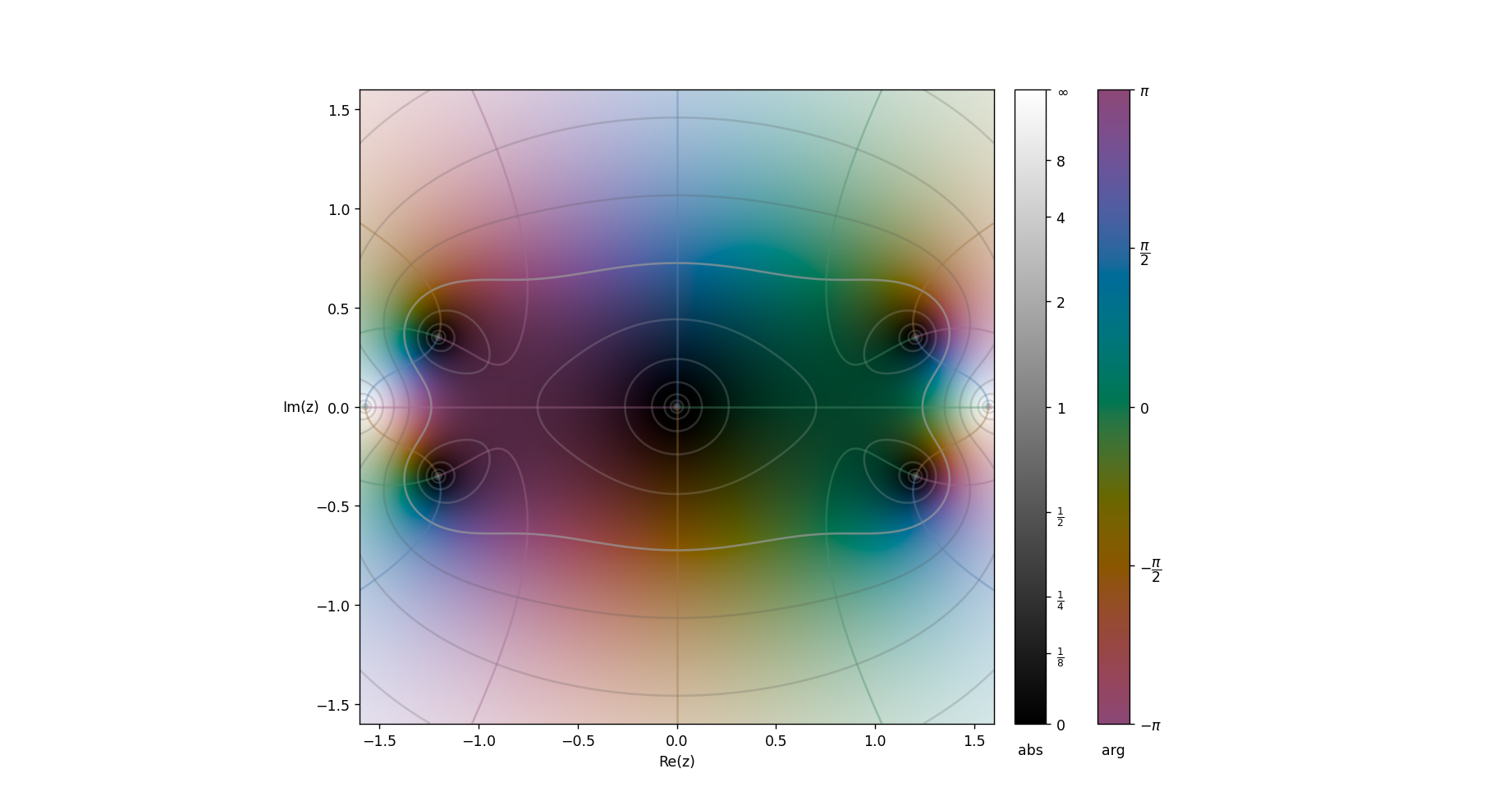
$\tan(z)-z^3$ (showing 5 roots):

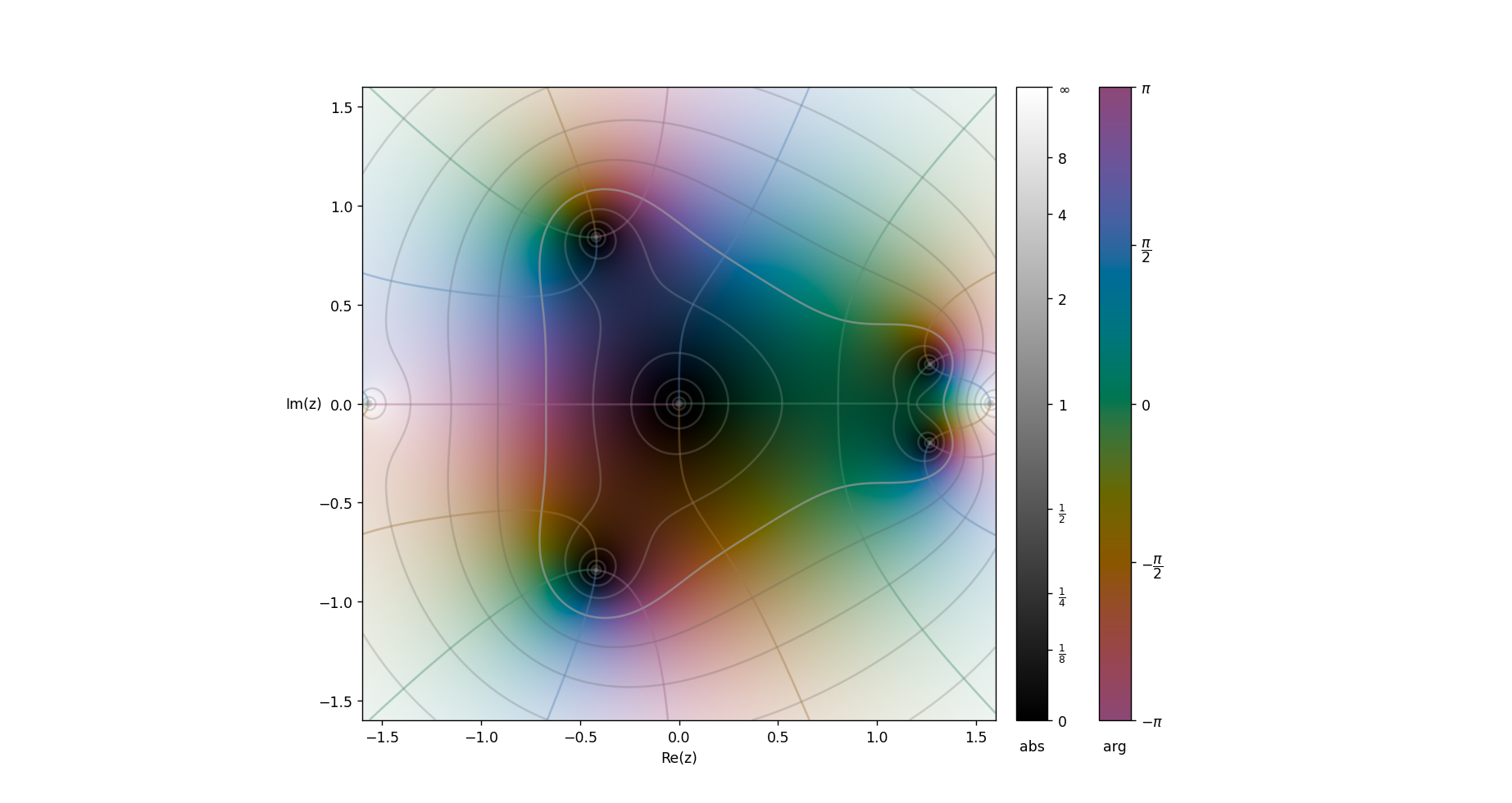
$\tan(z)-z^4$ (showing 5 roots):

$\tan(z)-z^5$ (showing 5 roots):

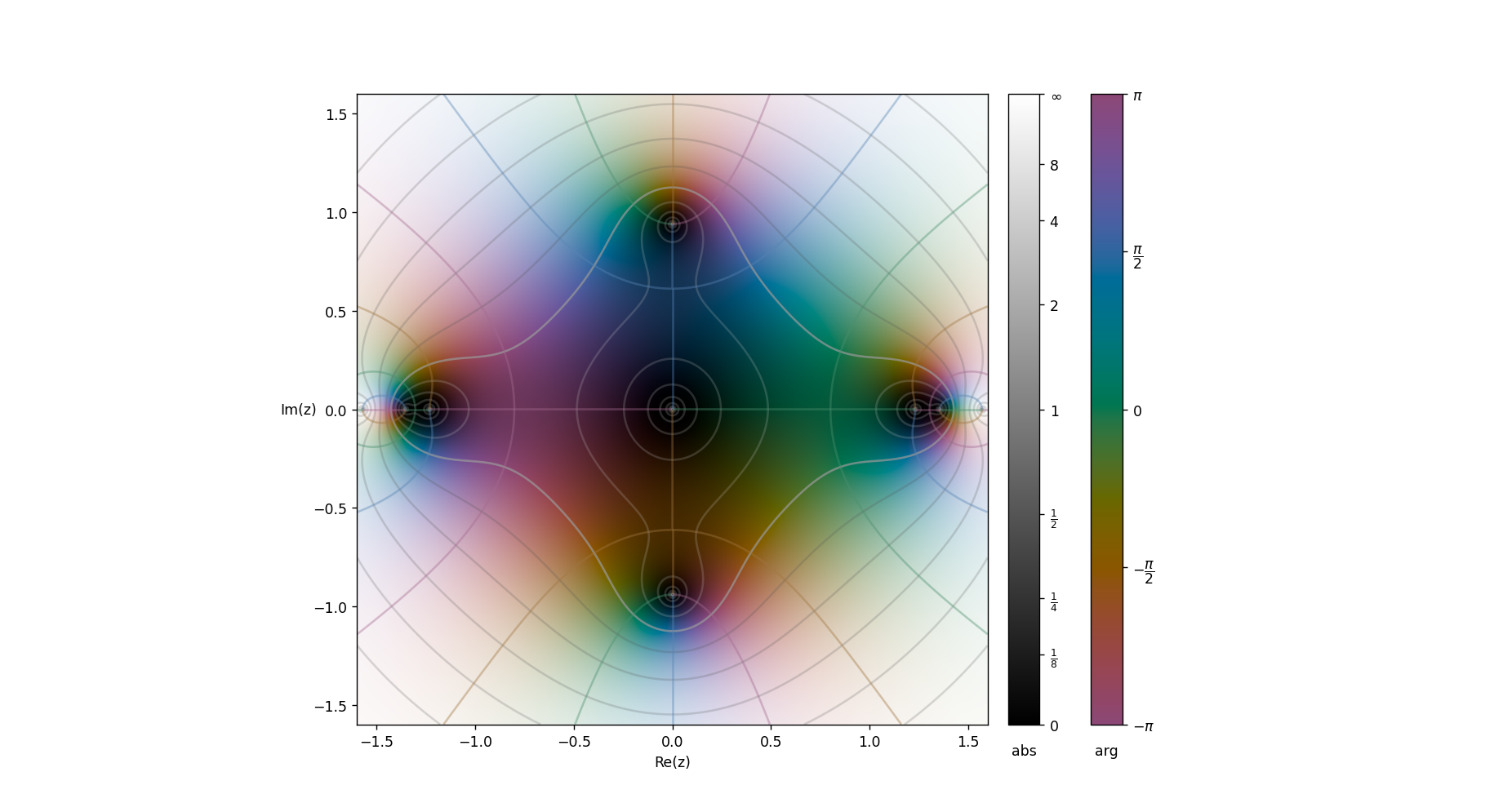
$\tan(z)-z^{11}$ (showing 11 roots):

$\tan(z)-z^{12}$ (showing 12 roots):

---

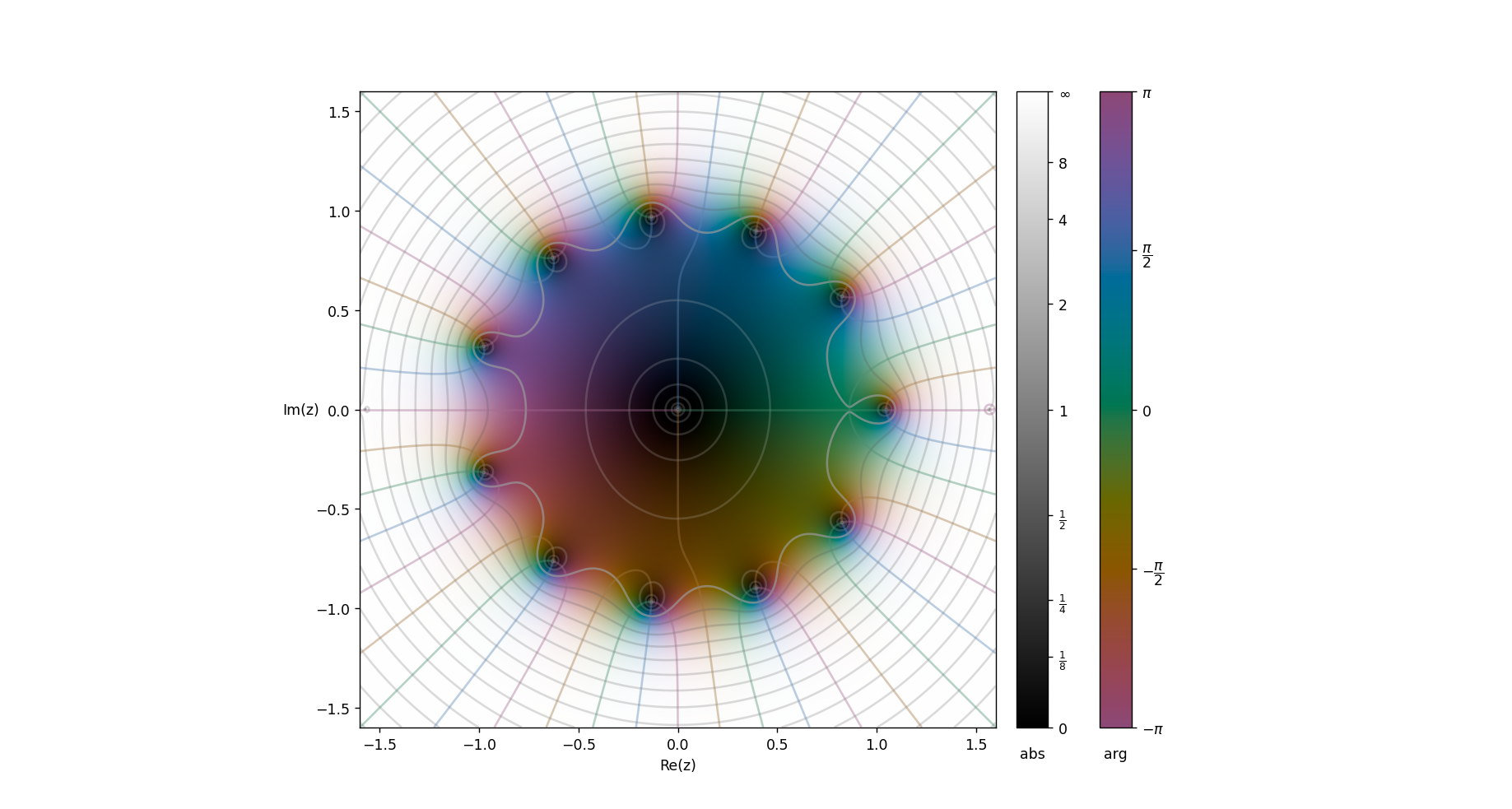
I used this python code:

import cplot
import numpy as np
print("enter  n  ")
n= int(input())

def f(z):
    res =np.tan(z)-z**n
    return res

plt = cplot.plot(f, (-1.6, +1.6, 1000), (-1.6, +1.6, 1000))
plt.show()

Define $t_n$ to be the number of roots that $\tan(z)-z^n$ have for $n \in \mathbb{N}$, $\frac{-\pi}{2}\le \Re(z)\le \frac{\pi}{2}$

The first few values are

$n $	$t_n$
1	1
2	3
3	5
4	5
5	5
6	6
7	7
8	8
9	9
10	10
11	11
12	12
13	13
14	14
15	15

$1,3,5,5,5,6,7,8,9,10,11,12,13,14,15,16$, it seems that for $n\ge5, \ a_n=n$ but I couldn't explain this behaviour or if it is true for all $n\ge5$.

---

In contrast to $\tan(z) - z^n$, the equations $\sin(z) - z^n = 0$ and $\cos(z) - z^n = 0$ always have exactly $n$ roots in the strip $|\text{Re}(z)| \leq \frac{\pi}{2}$. Also the equations $\cot(z) - z^n = 0$ and $\csc(z) - z^n = 0$ always have exactly $n+1$ roots in the strip $|\text{Re}(z)| \leq \frac{\pi}{2}$, However the equations $\sec(z) - z^n = 0$ is like the equation $\tan(z)-z^n$ i.e (have $n$ roots in the strip $|\text{Re}(z)| \leq \frac{\pi}{2}$ for $ n \ge 5$) .

How to explain this behavior?

Answer 1

Here is a sketch of an argument:

Since $\tan(z)= \frac{1}{i} \frac{e^{iz}-e^{-iz}}{e^{iz}+e^{-iz}}$, it is close to $\frac{1}{i}$ for $\Im(z)\gg 0$ and close to $-\frac{1}{i}$ for $\Im(z)\ll 0$. In particular, $|\tan(z)|$ is bounded above for large values of $\Im(z)$ and $z^n$ is bounded below for large values of $\Im(z)$, so $\tan(z)-z^n$ has no zeros outside of the region $-C\leq \Im(z)\leq C$.

We now let $\gamma$ be the contour given by the boundary of the rectangle $-\frac{\pi}{2} \leq \Re(z)\leq \frac{\pi}{2}$, $-C\leq \Im(z)\leq C$, minus two small disks of radius $\varepsilon$ around $\pm \frac{\pi}{2}$.

$|\tan(z)|$ stays bounded on $\gamma$, say by some $B$. For large enough $n$, we have that $|z^n|$ is strictly bigger than $B$ on all of $\gamma$, since $\gamma$ contains no $z$ with $|z|\leq 1$. So we can apply Rouche's theorem to see that $\tan(z)-z^n$ and $z^n$ have the same number of zeroes in our region, which is $n$.

This proves that in the region $-\frac{\pi}{2} \leq \Re(z)\leq \frac{\pi}{2}$ minus two small circles around $\pm \frac{\pi}{2}$, $\tan(z)-z^n$ has indeed exactly $n$ zeroes for large enough $n$ (where the precise numbers depend on the $\varepsilon$ you chose). However, there are also one or two zeroes in those circles: As $\tan(z)$ has a pole at $\frac{\pi}{2}$, $\tan(z)-z^n$ and $(z-\tfrac{\pi}{2})\tan(z) - (z-\tfrac{\pi}{2})z^n$ have the same zeroes. On the boundary of the circle of radius $\varepsilon$ around $\frac{\pi}{2}$, $(z-\tfrac{\pi}{2})\tan(z)\approx 1$, while $|(z-\tfrac{\pi}{2})z^n| \approx \varepsilon (\tfrac{\pi}{2})^n$, which is large for large enough $n$. So Rouche's theorem applies again to prove that $\tan(z)-z^n$ has the same number of zeroes in the interior of the circle of radius $\varepsilon$ around $\frac{\pi}{2}$ as $(z-\tfrac{\pi}{2})z^n$, which has exactly $1$. We can argue analogously for the circle of radius $\varepsilon$ around $-\frac{\pi}{2}$. We need to decide whether those two extra zeroes have real part in $[-\tfrac{\pi}{2},\tfrac{\pi}{2}]$.

Looking at real values of $z$, $\tan(z)-z^n$ is negative for $z=\frac{\pi}{2}-\varepsilon$, but $\tan(z)$ goes to $+\infty$ as $z\to \frac{\pi}{2}$ from below in the reals, so it must become positive again. So we always have a zero with real value $<\frac{\pi}{2}$ in the circle of radius $\varepsilon$ around $\frac{\pi}{2}$, which is the only one by the above argument. Around $-\frac{\pi}{2}$ we argue similarly, but because the sign of $z^n$ depends on the parity of $n$, the zero will have real value $>-\frac{\pi}{2}$ exactly if $n$ is odd (and $<-\frac{\pi}{2}$ otherwise).

Altogether we find $n+2$ zeroes in the region $-\frac{\pi}{2}\leq \Re(z)\leq \frac{\pi}{2}$ for large odd $n$, and $n+1$ for large even $n$.

EDIT: The below picture shows the minimal $n\geq 1$ for which $\tan(z)$ is dominated by $z^n$. Specifically, $n=1$ in the white region, $n=2$ yellow, $n=3$ orange, $n=4$ light red, $n=5$ dark red. So it seems that the above arguments go through for $n\geq 5$ and $\varepsilon=0.3$ (blue contour).