1
$\begingroup$

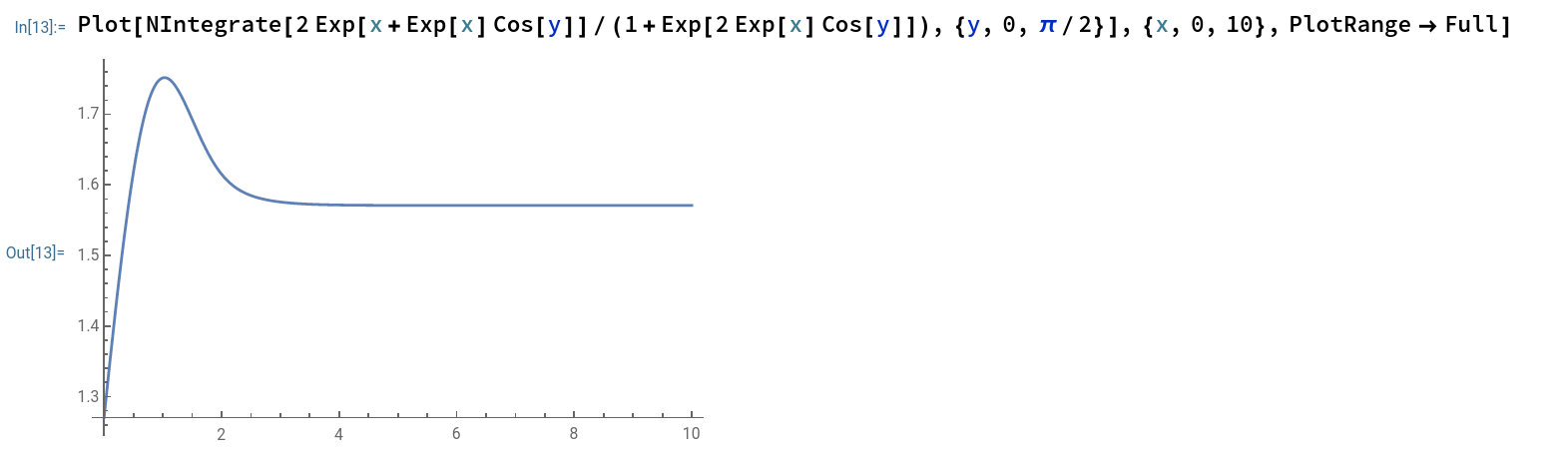
Here is a question that really has puzzled me for quite a while. I happened to see this function defined in terms of an integral $$f(x):=\int_0^{\pi/2}\frac{2e^{x+e^x\cos y}}{1+\left(e^{e^x\cos y}\right)^2}dy.$$I want to analyze the behavior of the function when $x \rightarrow \infty$.

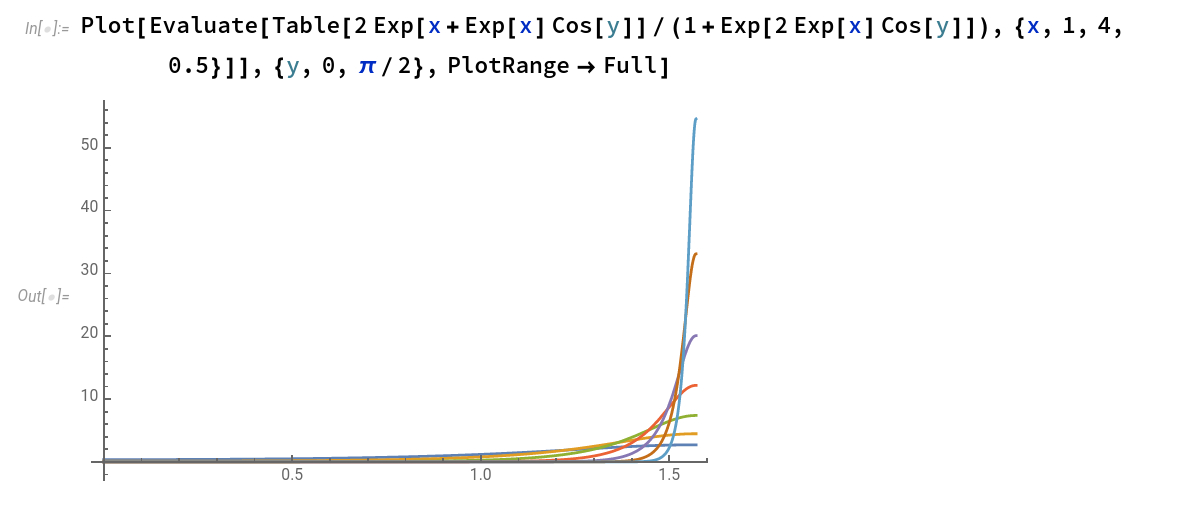
The strange thing is that when I used Mathematica to plot the function, the graph indicates that $\lim_{x\rightarrow \infty} f(x)=0$. However, it is easy to see that $\liminf_{x\rightarrow \infty}f(x) \ge \frac{\pi}{4}$, since $$\int_0^{\pi/2}\frac{2e^{x+e^x\cos y}}{1+\left(e^{e^x\cos y}\right)^2} \, dy \ge \int_0^{\pi/2}\frac{2e^{x+e^x\cos y}\sin y}{1+\left(e^{e^x\cos y}\right)^2}\, dy\\ =-\Big(\tan^{-1}\left(e^{e^x \cos y}\right)\Big)\Big|_{0}^{\pi/2}\\=\tan^{-1}\left(e^{e^x}\right)-\pi/4$$

Now I have two questions:

First, why the result from Mathematica is different from what I obtained?

Second, does $\lim_{x\rightarrow \infty} f(x)$ exist?

Maybe this question is not so suitable for mathoverflow, since it is just a calculus problem. However, I just feel so confused about the contradiction of numerical result and math. I want to understand the reason behind this situation. Any comments are really appreciated. Thank you very much.

Below is the code and picure I got from Mathematica....

enter image description here

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ you probably made a coding error in Mathematica, when I plot it the limit is about 1.57 $\endgroup$
    – Carlo Beenakker
    Commented Jun 14, 2020 at 6:58
  • 2
    $\begingroup$ You missed $2$ in antiderivative, so $\liminf$ is at least $\frac{\pi}{2}$ (which coincides with the number Carlo wrote). I bet that it won't be hard to prove that the the loss in multiplying by $\sin (y)$ is negligible as $x\to \infty$ and so the limit is actually $\frac{\pi}{2}$. $\endgroup$
    – Aleksei Kulikov
    Commented Jun 14, 2020 at 7:18
  • $\begingroup$ @CarloBeenakker, thank you very much for the comment... As you can see from my updates, my code should be fine, but when I tried to plot the the graph for $x \in [0,20]$, something went wrong... $\endgroup$
    – student
    Commented Jun 14, 2020 at 13:31
  • $\begingroup$ @AlekseiKulikov, exactly! Thank you very much! $\endgroup$
    – student
    Commented Jun 14, 2020 at 13:31

1 Answer 1

Reset to default
3
$\begingroup$

Mathematica seems to be plotting the function just fine...

plot of the function

If we look a bit at the integrand, it's clear that most of the mass is around $y = \pi/2$ as $x$ increases which should let us introduce a $\sin y$ term and use the antiderative you've already found.

enter image description here

We can try to cut the integral at $\pi/2 - 1/x$.

Let $$I = \int_0^{\pi/2 - 1/x} 2 e^x \frac{e^{e^x \cos y}}{1 + e^{2 e^x \cos y}} dy + \int_{\pi/2 - 1/x}^{\pi/2} 2 e^x \frac{e^{e^x \cos y}}{1 + e^{2 e^x \cos y}} dy = I_0 + I_1$$

Notice that $f(u) = e^u / (1 + e^{2u})$ is a decreasing function of $u$ and thus that $$I_0 < (\pi/2 - 1/x) 2 e^x \frac{e^{e^x \cos (\pi/2-1/x)}}{1 + e^{2 e^x \cos (\pi/2-1/x)}} $$

When $x \rightarrow \infty$ the $\cos (\pi/2 - 1/x)$ behaves as $1/x$ and the logistic function $1-\sigma(u)$ behaves as $e^{-u}$, so the right term behaves as $\pi e^{x - e^x /x}$ which converges to $0$.

For $I_1$, we note that if $y \in [\pi/2-1/x,\pi/2]$, $1 - \frac{1}{2x^2} < \sin y \leq 1$

$$I_1 \left(1-\frac{1}{2x^2}\right)< \int_{\pi/2-x}^{\pi/2} 2 e^x \frac{e^{e^x \cos y}\sin y}{1 + e^{2 e^x \cos y}} \leq I_1 $$

The middle term converges to $\pi /2$ and thus so does $I_1$ and so does $I$.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you very much! By the way, I got the same picture for $x$ up to 10, but when I plotted the graph for $x \in [0,20]$, it gives me the limit going to $0$, as you can see from my updates.... $\endgroup$
    – student
    Commented Jun 14, 2020 at 13:26
  • $\begingroup$ The support of the mass becomes too small and the numerical integration misses it. If you do a change of variable which blow up the region around pi/2 it'll be more stable. $\endgroup$
    – Arthur B
    Commented Jun 14, 2020 at 13:44

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .