3
$\begingroup$

While teaching Calculus 2, one of my students asked me the following

Given 3 points $x_1$, $x_2$, $x_3$. Whether there exists one function $z = f(x,y)$ which has exactly 2 extremum and 1 saddle point: 1 local minimum at $x_1$, 1 local maximum at $x_2$, 1 saddle point $x_3$.

I looked it up in Stewart and several other textbooks but have not found an answer. Any suggestions or comments are welcome.

Improve this question
$\endgroup$
9
  • 1
    $\begingroup$ @PietroMajer : Can you explain how to push them to infinity by a diffeo? $\endgroup$
    – Iosif Pinelis
    Commented Jan 8 at 19:19
  • 1
    $\begingroup$ Maybe the expression was too vague/colored; I mean just this: Suppose $f:\mathbb R^n\to\mathbb R$ has many critical points with various indices. If $\phi:\mathbb R^n\to\Omega\subset \mathbb R^n$ is a diffeo, then $g:=f\circ \phi$ has critical points in bijection with the critical points of $f$ in $\Omega$, with same index. $\endgroup$
    – Pietro Majer
    Commented Jan 8 at 19:50
  • 1
    $\begingroup$ @PietroMajer : I understand this. But how do you construct a diffeo from $\mathbb R^n$ onto a given open subset $U$ of $\mathbb R^n$, say $U:=\mathbb R^n\setminus F$, where $F$ is a finite set? $\endgroup$
    – Iosif Pinelis
    Commented Jan 8 at 20:10
  • 2
    $\begingroup$ @PietroMajer : I see now: for a finite $F$, you can push points in $F$ to inifinity one-by-one. $\endgroup$
    – Iosif Pinelis
    Commented Jan 8 at 20:31
  • 1
    $\begingroup$ Or also if G is the finite set of all the others critical points, we can connect them by a path (or a tree) $\Gamma$ that avoids F, and take $\Omega$ as a nbd of $\Gamma$ $\endgroup$
    – Pietro Majer
    Commented Jan 8 at 21:05

2 Answers 2

Reset to default
3
$\begingroup$

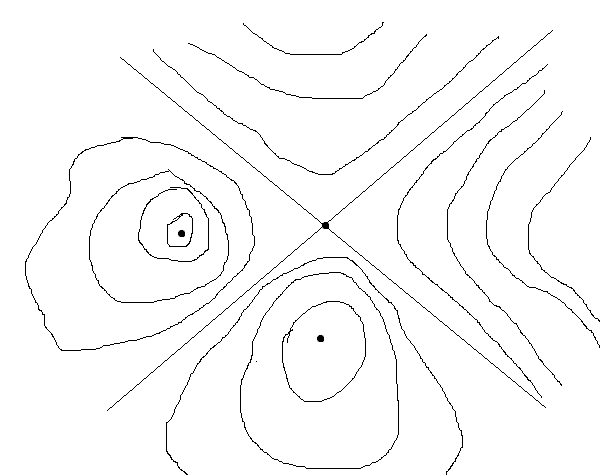
$\newcommand\R{\mathbb R}$ $$f(x,y)=\frac{2}{x^2+(y-1)^2+1}-\frac{1}{x^2+(y+1)^2+1} \tag{1}\label{1}$$ will do.

Indeed, this function $f$ has exactly three critical points: a saddle point $(0,\approx-8.54)$, a point of a local minimum at $(0,\approx-1.14)$, and a point of a local maximum at $(0,\approx1.04)$.

For any given three distinct points $P,Q,R$ in $\R^2$ to be exactly the critical points of a function -- with a saddle at $P$, a local minimum at $Q$, and a local maximum at $R$, use $f\circ g$ instead of $f$, where $g$ is a diffeomorphism of the plane moving the given three distinct points $P,Q,R$ to the respective critical points $(0,\approx-8.54)$, $(0,\approx-1.14)$, $(0,\approx1.04)$ of $f$.

Such a diffeomorphism exists, because

  • any three noncollinear points in $\R^2$ can be moved to any other three noncollinear points in $\R^2$ by a nonsingular affine transformation of $\R^2$ (which is of course a diffeomorphism);
  • any three collinear points in $\R^2$ can be moved to some three noncollinear points in $\R^2$ by a diffeomorphism; say, three distinct collinear points $(0,y_1)$, $(0,y_2)$, $(0,y_3)$ in $\R^2$ can be moved to the three noncollinear points $(e^{y_1},y_1)$, $(e^{y_2},y_2)$, $(e^{y_3},y_3)$ by the diffeomorphism $h$ defined by the formula $h(u,v):=(u-e^v,v)$ for $(u,v)\in\R^2$;
  • any diffeomorphism preserves critical points, local maxima points, local minima points, and saddle points.

Below are the calculations for $f$, in Mathematica:

enter image description here enter image description here

Improve this answer
$\endgroup$
2
$\begingroup$

Just draw a contour plot starting with a saddle: enter image description here

You can assign pretty much arbitrary value to each contour within the limits of common sense, so for an appropriate choice of values this diagram will give you exactly what you wanted.

How rigorous is that? IMHO it is absolutely rigorous and can be relatively easily translated into gluing a few smooth functions by hand or using an appropriate partition of unity, but it is true that our calculus textbook do not teach students how to translate from pictures to formulas and back and concentrate on differentiating and integrating algebraic/trigonometric expressions that Mathematica (or even chatGPT) can do much better than an average undergraduate...

Improve this answer
$\endgroup$
2
  • $\begingroup$ I agree that this is rigorous, and I did think about this possibility. However, the realization of this approach seems rather laborious, especially the gluing should be done rather carefully, and the eventual product will probably be a piecewise-defined function with complicated expressions, especially after the gluing. My efforts were directed to finding a very simple expression for a function in question. Of course, I am being apologetic here. :-) $\endgroup$
    – Iosif Pinelis
    Commented Jan 9 at 12:47
  • $\begingroup$ @IosifPinelis "Can you draw such a contour plot?" Just curve the crossing straight lines. The picture is topological, not metric, so you can make any curve escaping to $\infty$ a straight line and any 3 points lie on such curve. $\endgroup$
    – fedja
    Commented Jan 9 at 17:43

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .