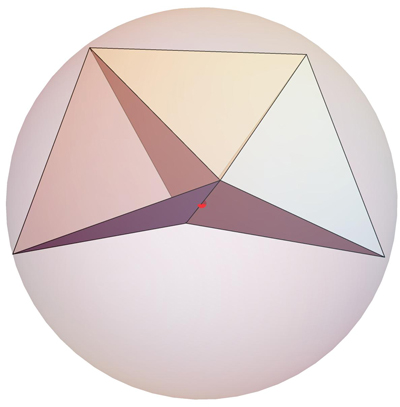
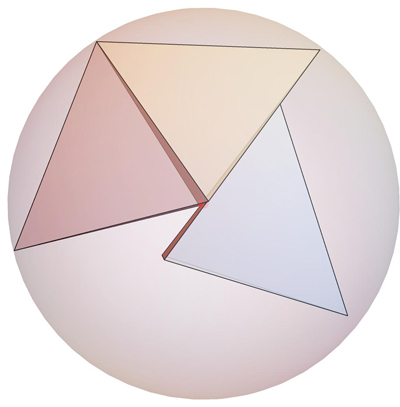
What is the smallest possible radius of a sphere which contains 3 identical plastic tetrahedra with side length 1?
$\begingroup$
$\endgroup$
6
-
$\begingroup$ I don't understand this question. Is there some condition on the location of the tetraherdra? $\endgroup$– Richard StanleyCommented Nov 5, 2023 at 0:46
-
2$\begingroup$ Presumably the OP meant: three unit regular tetrahedra with disjoint interiors. $\endgroup$– Joseph O'RourkeCommented Nov 5, 2023 at 1:05
-
$\begingroup$ What is the significance of the word, "plastic"? Does it mean you can change the shape of each tetrahedron at will? $\endgroup$– Gerry MyersonCommented Nov 5, 2023 at 2:12
-
1$\begingroup$ @GerryMyerson: I think it might just mean “solid” in this context. $\endgroup$– Sam HopkinsCommented Nov 5, 2023 at 2:20
-
2$\begingroup$ I think it means the OP is playing with plastic tetrahedra. Possibly dice, some games have used them, although they don't roll well. $\endgroup$– Will JagyCommented Nov 5, 2023 at 3:41
|
Show 1 more comment
1 Answer
$\begingroup$
$\endgroup$
4
Unclear this is best, but: $r=\sqrt{3}/2$.
JukkaKohonen's suggestion:
I made no attempt to optimize, but this certainly shows the smallest sphere has radius strictly less than $\sqrt{3}/2$.
-
$\begingroup$ Hmmm, this is nonunique. You can pivot the tetrahedra about the triply shared edge. $\endgroup$ Commented Nov 5, 2023 at 23:15
-
$\begingroup$ @OscarLanzi: Yes, you are right. $\endgroup$ Commented Nov 5, 2023 at 23:30
-
4$\begingroup$ It seems you could slide the rightmost tetrahedron down and left, along the face that it shares with its neighbor, and the radius would decrease. $\endgroup$ Commented Nov 6, 2023 at 10:23
-
$\begingroup$ @JukkaKohonen Try simultaneously pivoting the central tetrahedron about the triply shared edge of the current figure as well as sliding the left one. $\endgroup$ Commented Nov 6, 2023 at 10:34