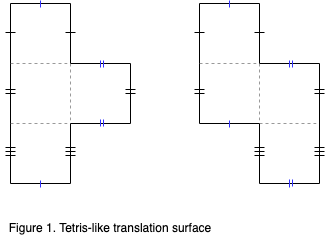
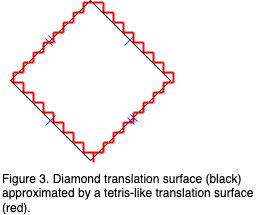
I'm reading the survey "An introduction to Veech surfaces" by Pascal Hubert and Thomas Schmidt.
At page 19 they state "In any fixed stratum, the set of square-tiled surfaces of that stratum is dense.". The reason should be that in the coordinates for the moduli space of translation surfaces given by the period map (integration of the 1-form on relative periods) the coordinates of the translation surfaces are exactly $\mathbb{Q}+i\mathbb{Q}$. So the assertion follows because of the density of $\mathbb{Q}$ in $\mathbb{R}$.
Well, it's hard for me to believe in Hubert and Schmidt's assertion.
Isn't it true that the square tiled surfaces are the "integer points" of the moduli space of translation surfaces? If I have a translation surface tiled by squares with the side of length one, isn't it true that the relative periods are contained in $\mathbb{Z}+i\mathbb{Z}$?
So my questions are:
Is Hubert and Schmidt's assertion wrong?
If so, which are the translation surfaces corresponding to rational points?
Thank you