If a polyhedron in $\mathbb{R}^3$ has local intersections, does it also have more global intersections?

Question

Consider a simplicial complex $K$. A piecewise linear map $f: K \to \mathbb{R}^n$ is an almost-embedding if $f(\sigma) \cap f(\tau) = \emptyset$ for any two disjoint simplices $\sigma,\tau$ in $K$.

Question 1: Is every almost-embedding an embedding in the case where $K$ is a simplicial 2-manifold, and $n=3$?

There is some related discussion in Segal, Skopenkov, Spieiz, "Embeddings of polyhedra in $\mathbb{R}^n$ and the deleted product obstruction", but they specifically leave this case open (2-manifolds into $\mathbb{R}^3$).

Alternatively, I would be happy with an answer to the following variant:

Question 2: Suppose $K$ is a simplicial 2-manifold where every vertex has degree at least 4, and $f: K \to \mathbb{R}^3$ is a piecewise linear map such that $f(\sigma) \cap f(\tau) = \emptyset$ for any two disjoint 2-simplices $\sigma,\tau$ in $K$. Is $f$ necessarily an embedding?

Here, triangulations with degree-3 vertices are excluded since otherwise there are some obvious counterexamples, e.g., the tetrahedron with all four vertices coplanar. (This case is already excluded from Question 1, since any vertex and the complementary triangle are disjoint.)

Here are some not-quite-counterexamples to consider:

In both cases, $K$ is a simplicial 2-sphere. On the left there is an intersection between triangles $t_1$ and $t_2$ (which share an edge $e_1$), but this map is not quite an almost-embedding because the images of disjoint edges $e_2$ and $e_3$ intersect at the point $p$. On the right, there is an intersection between triangle $t_1$, with vertices $i,k,l$, and triangle $t_2$, with vertices $i,j,l$, but this map is not quite an almost-embedding because the images of disjoint triangles $t_3$ and $t_4$ intersect at $f(k)$.

Answer 1

"Yes" for Question 1.

We need to show that interiors of distinct smplexes $\alpha$ and $\beta$ do not intersect. Assume $\mathring \alpha\cap\mathring \beta\ne\varnothing$. Then $\alpha$ and $\beta$ share a simplex, say $\sigma$. Note that $\sigma\in\partial (\alpha\cap\beta)$. Choose a point $z\in \alpha\cap\beta$ that maximize the distance to $\sigma$. Denote by $\alpha'$ and $\beta'$ the smallest subsimplexes of $\alpha$ and $\beta$ that contain $z$. Observe that $\alpha'\cap\beta'=\varnothing$.

Answer 2

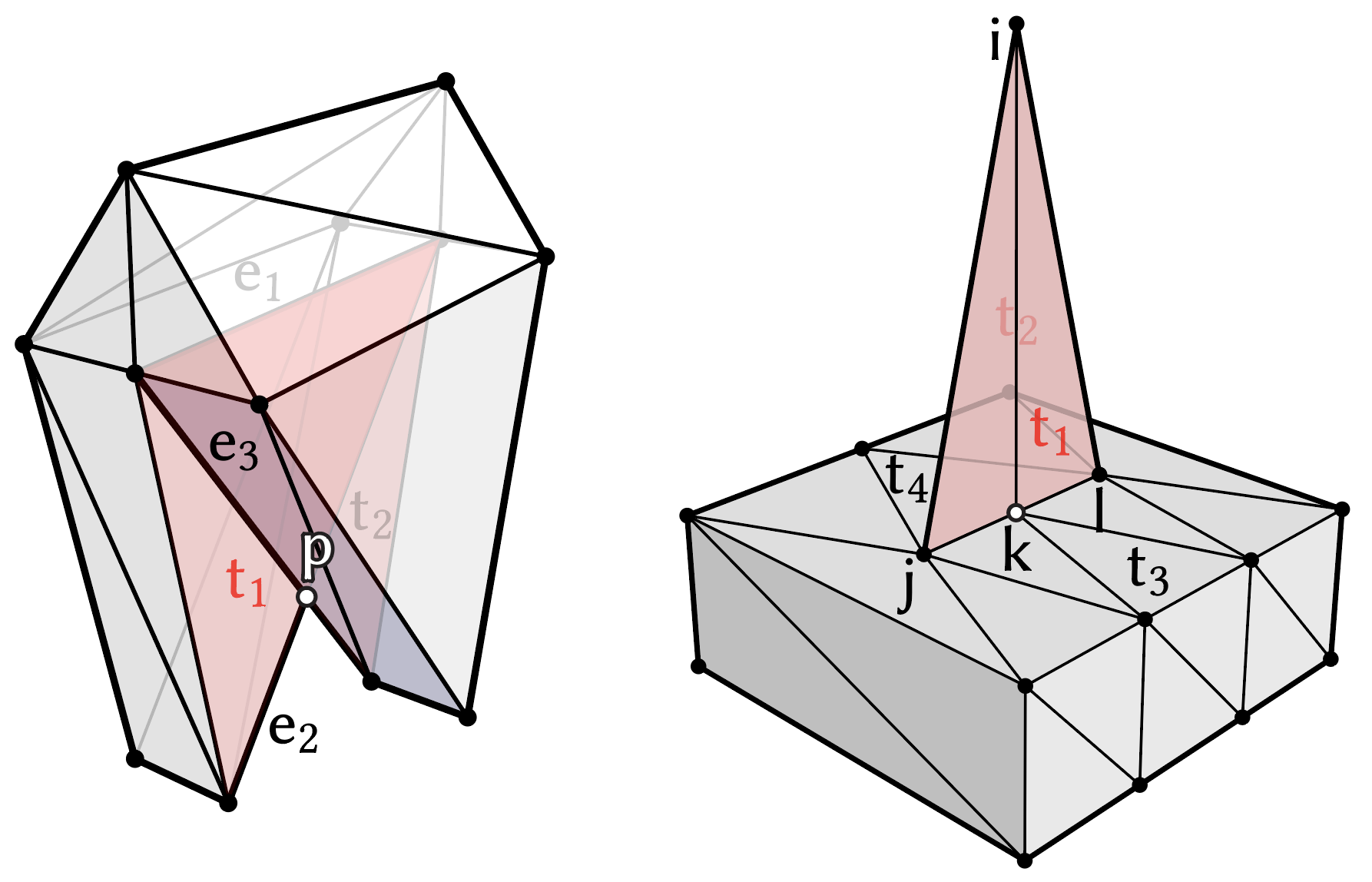
Sorry, my previous answer is based on the impression that the "manifold" can mean "manifold with boundary. In that case, the countercase can be constructed easily (see my previous answer). But for true manifold, here is my answer:

Question 1: Yes, except the simplicial 1-manifold embedded to 2/3D. But I think this case can always be viewed as a slicing of the 2 manifolds.

Question 2: I actually believe this is true. I even drafted a proof:

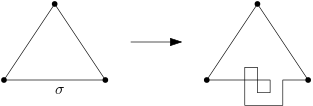
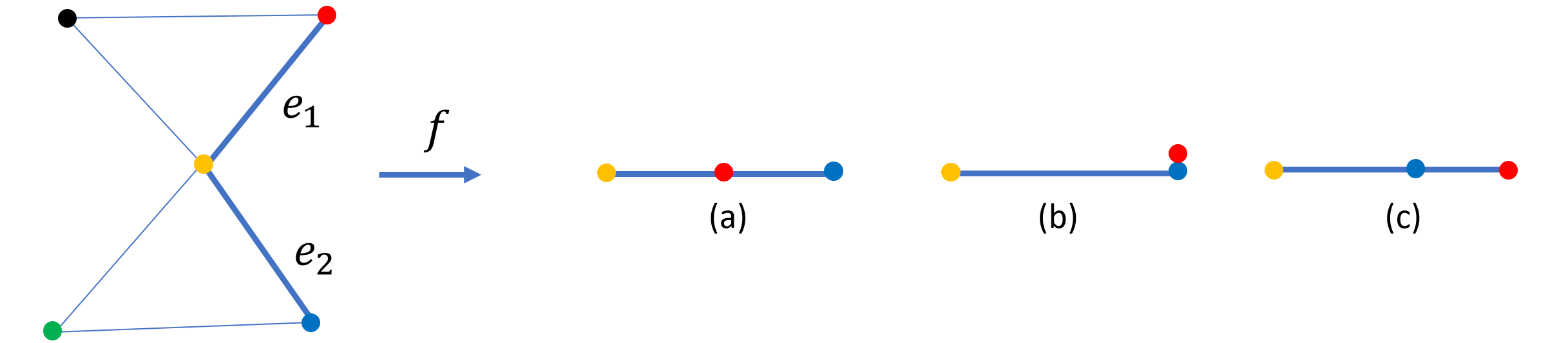
1. First let's filter out all the degenerate cases. There are 3 degenerate cases, as shown in the following figure. (a,b) correspond's to the rank of $\nabla f$ being 1; (c) corresponds to the rank of $\nabla f$ being 0. Both violate the almost-embedding definition (a: green vertex and the bottom edge; b: green vertex and the red vertex; c: all three ).

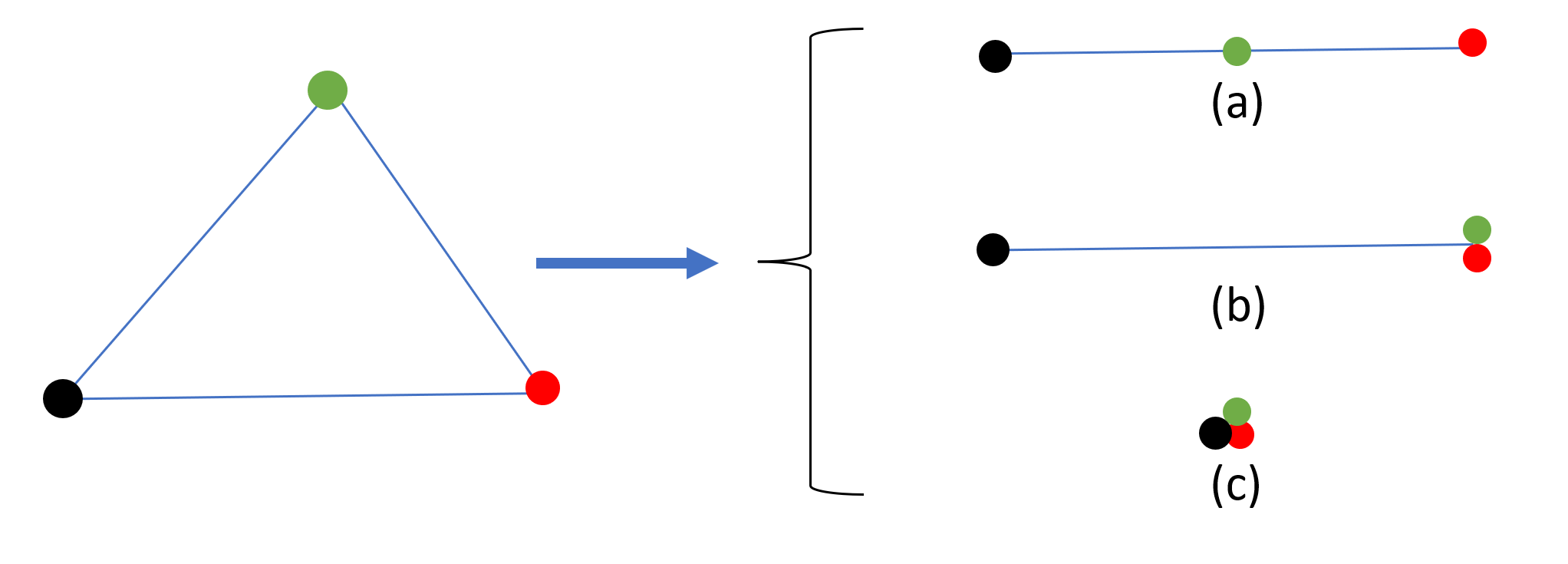
2. Then we can actually check whether there can be a local intersection that satisfies the almost-embedding definition. We only need to find 2 2-simplex (triangle): $t_1$ and $t_2$ from the embedding of the manifold, s.t., $t_1 \cap t_2\neq \emptyset$, and an emersion $f$, s.t., $f(t_1/(t_1\cap t_2))\cap f(t_2/(t_1\cap t_2))\neq\emptyset$, and $f$ is causing no intersection of non-adjacent faces.

3. $t_1 \cap t_2\neq \emptyset$ includes two cases: they can share 1 or 2 vertices (but not 3). as shown in the following figure. We can break it down to different cases and prove they all violate the definition of almost embedding.

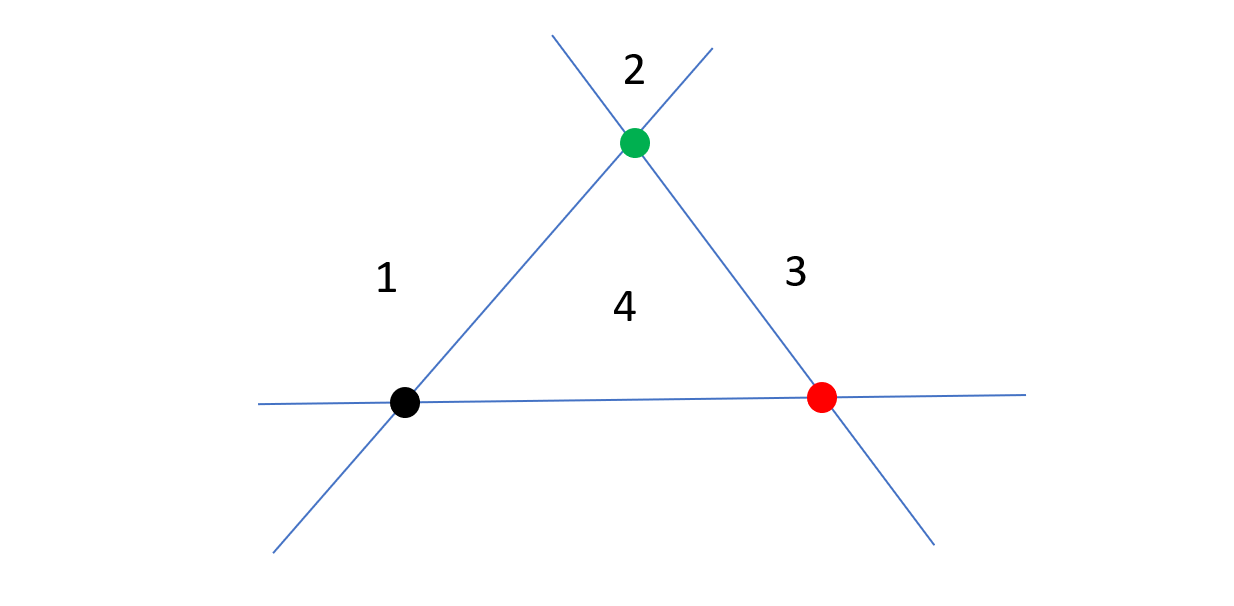
4. If $t_1 $ and $ t_2$ shares 2 vertices and $f(t_1/(t_1\cap t_2))\cap f(t_2/(t_1\cap t_2))\neq\emptyset$, $f(t_1)$ and $f(t_2)$ must be coplanar, then we can just enumerate all the intersection cases of $f(t_1)$ and $f(t_2)$, there are totally 4 cases (mode out the symmetry). All of them are not almost embedding, violating parts are marked by the red cross. 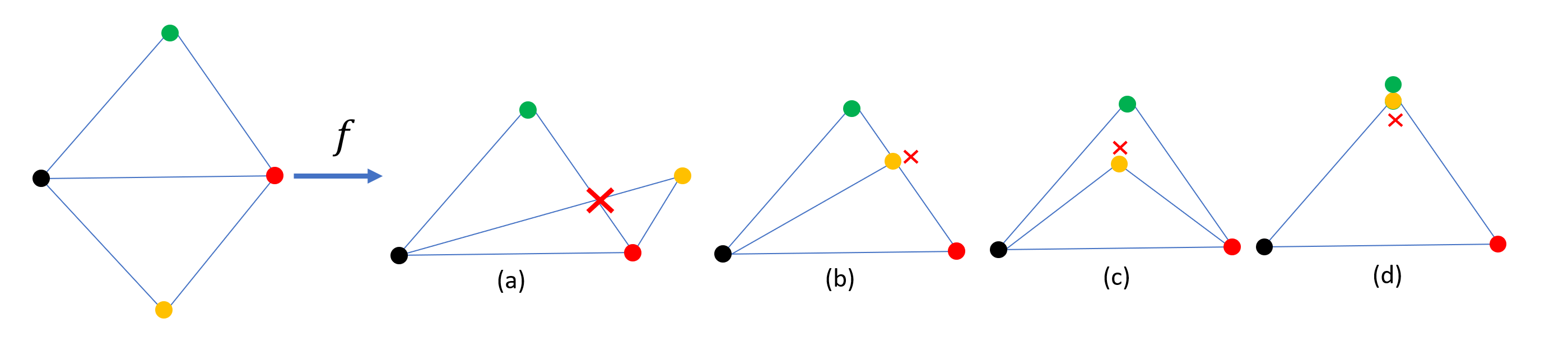We can guarantee this is an exhaustive list, because there are local intersections if an only if the yellow vertex is mapped to one of the regions 1,2,3,4 (or the boundary between them) in the following figure. Algebraic analysis can prove the intersection condition and the categorization of the cases.

5. Case 2: $t_1$ and $t_2$ only share one vertex. In this case if $f(t_1/(t_1\cap t_2))\cap f(t_2/(t_1\cap t_2))\neq\emptyset$ we can further categorize it into 2 subcases:

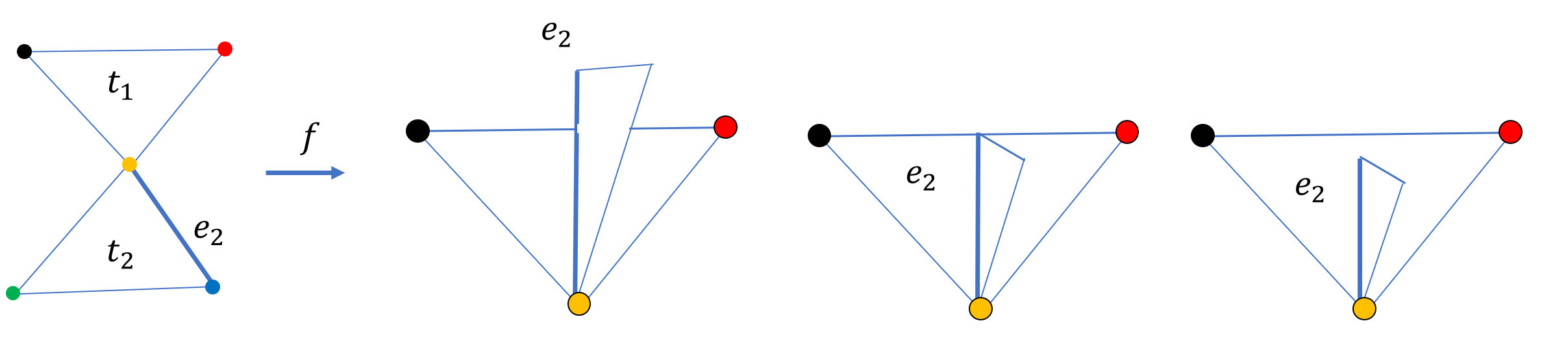
5.1. Edge intersections: when $f(e_1)^\circ \cap f(e_2)^\circ \neq \emptyset$. where $e_1, e_2$ are edges, $e_1 \in t_1$ and $e_2 \in t_2$ and $e_1 \cap e_2=$shared vertex. There are 3 cases, as shown in the figure. None of them are almost embedding.

5.2. $f(t_1)^\circ \cap f(e_2)^\circ \neq \emptyset$, where $e_2 \in t_2$ and contains the shared vertex. There are 3 cases, and non of them are almost embedding.

5.3. Every case that is neither 5.1 nor 5.2. Then e mush $f(t_1)^\circ \cap f(t_2)^\circ \neq \emptyset$. This is a very intersting case, actually, it can be handled similarly to 5.1. Denote $e_1$, $e_2$ as the edge of $t_1$ and $t_2$ opposite to the shared vertex. In this case, eigher $f(e_1) \cap f(t_2)^\circ \neq \emptyset$, or $f(e_2) \cap f(t_1)^\circ \neq \emptyset$, or or $f(e_1) \cap f(e_2) \neq \emptyset$. Either way, it's not an almost embedding.

We've proved that in every possible case, the local intersection cannot be an almost embedding. Thus question 2 is proven.

---

Below are my old answers. Have you considered the simplicial 3-manifold cases?

For Question 1: I think an almost-embedding can also be a 3D simplicial complex (tetrahedral mesh) mapped to $\mathcal{R}^3$ (for example, an identity map).

For Question 2: You might have degenerate cases or flipped cases, like this $\mathcal{R}^2 \mapsto \mathcal{R}^2 \times \{0\}$ case shown in the figure. Because the bijectivity of already adjacent simplexes is not controlled by the definition. (the website won't let me directly post images)

Answer 3

As stated, the answer to both questions is no. A counterexample is: Take any surface PL-embedded in $\mathbb{R}^3$. This can be for example boundary of the regular octahedron in order to satisfy the conditions of Question 2.

Then take any embedded $2$-simplex $\sigma$ and locally form a self-intersection in the interior of $\sigma$. One can for example `pull a finger' from the interior and then to let it intersect with other part of the interior in a circle. (The picture below shows an analogous move for 1-manifolds in the plane.) If this is performed locally, then no other simplices are affected. Therefore we have an almost embedding but not an embedding.