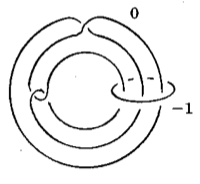
I don't know where you took the picture from, but that link is not symmetric (and it likely does not describe the correct manifold).
Indeed, the 0-framed component of the link you drew is a trefoil: its diagram has three crossings and it is alternating. If you look at Figure 2 in Akbulut and Kirby's paper, the top crossing is the opposite than the one you drew here. EDIT: the diagram does represent the unknot... It's true that it's alternating, but it has a trivial Reidemeister-1 move available. Thanks Ryan for pointing this out. The rest of the answer is (as far as I can tell) still correct.
Once you switch those two crossings, Figure 2 in Kyle Hayden's paper displays the symmetry Akbulut and Kirby refer to: the link is isotopic to a link that has a symmetric diagram, so the two components can be swapped. Note that it's not symmetric as a framed link. That is to say: this is not an instance of a cork, at least not in an obvious way (if you wanted an obvious cork, just put framing 0 on both components and you get what in the literature is called the Mazur cork).
As for the question
is it always true?
I don't know what you mean exactly when you say "always". There is no reason why a link with two unknotted components should have an isotopy swapping the two components. The multi-variable Alexander polynomial, for instance, should be able to tell you that sometimes this cannot happen. It looks to me like the link L9n19 should give such an example.