This is joint work with Ilkka Törmä (50-50, I am just more interested in points than him).
We did not see a link to cohomology, but your technical question was very interesting, and we believe we solved it.
Theorem. Let $Y$ be a mixing sofic shift and $Z$ a mixing SFT contained in $Y$. Then the following are equivalent:
- There exist a mixing SFT $X$ and a factor map $\pi : X \to Y$ such that for some subshift $Z'$ of $X$, $\pi|_{Z'}$ is a conjugacy.
- There exist a mixing SFT $X$ and a factor map $\pi : X \to Y$ such that $Z$ admits a shift-commuting continuous section $\phi : Z \to X$.
- $Y$ and $Z$ satisfy the first-order formula
$\forall s \in L(Y): \exists u, w \in L(Y): \exists a, b \in L(Z): \forall v \in L(Z): (a v b \in L(Z) \implies s u a v b w s \in L(Y)).$
Proof. 1 $\iff$ 2. is trivial and was noted in the post.
For 2 $\implies$ 3., suppose such $X$, $\pi$ and $\phi$ exist.
We may recode them so that $X$, $Z$ and $Z' = \phi(Z)$ are nearest-neighbor SFTs, $\pi$ has radius $0$ and $\phi$ has radius $1$. The recoding for $\pi$ is standard (you can do it first), others come from simple higher block coding, i.e. remembering multiple symbols at once.
Note that nearest-neighbor SFTs are equivalent to vertex shifts, i.e. configurations are sequences of vertices that form a valid path in a graph; we use this terminology. In particular, $Z'$ corresponds to a (not necessarily induced) subgraph of $X$.
Take any word $s \in L(Y)$ and choose a $\pi$-preimage $s' \in L(X)$ for it.
Extend $s'$ into a word $s' u' \in L(X)$ that can be followed by a vertex of $Z'$, and then extend it by a path of three $Z'$-vertices $a' = a'_1a'_2a'_3$ to get some $s' u' a'$.
Let $b' w' s'$ be defined similarly, but extending $s'$ to the left.
Let $u, w, a, b$ be the $\pi$-images of $u', w', a', b'$.
Note that $a, b, a', b'$ all have length $3$.
Take any $v$ such that $a v b \in L(Z)$.
Then $c v' d = \phi(a v b)$ has length equal to $|v| + 4$ (when applying a radius-$1$ map to a word, the length decreases by one on each side), $|v'| = |v|$, $|c| = |d| = 2$. Now observe that $a'$ maps to $a$ in $\pi$ and $a'$ is a word in $Z'$, so $\phi$ maps $a$ to the central vertex of $a'$; similarly for $b$ and $b'$. Therefore the first vertex of $cv'd$ is just the second vertex of $a$, and similarly the last vertex of $cv'd$ is the second vertex of $b$.
Since $X$ is nearest-neighbor, we have $s' u' a'_1 c v' d b'_3 w' s' \in L(X)$. The $\pi$-image of this word is $s u a v b w s \in L(Y)$, which proves the first-order formula.
For 3. $\implies$ 2., we may recode $Y$ so that $Z$ is an edge shift.
Let $\pi' : Y' \to Y$ be the Fischer cover of $Y$ (the minimal right-resolving cover), which is the labeled edge shift whose vertices are follower sets of words in $L(Y)$.
Let $s \in L(Y)$ be a synchronizing word, that is, one for which any $\pi'$-preimage terminates at the same vertex $q$ in $Y'$.
Let $u, w, a, b$ be given for $s$ by the assumption.
We add new vertices and edges to $Y'$: from each vertex $p$ that can be followed by $s$, a path $P$ with label $s u a$ (which merge after their first edges); a copy of the graph of $Z$; and a path $Q$ with label $b w s$ terminating at $q$.
For any edge $e$ in $Z$ that can follow $a$, we add a new edge with label $e$ from the endpoint of $P$ to the endpoint of $e$.
For any edge $e$ in $Z$ that can precede $b$, we add a new edge with label $e$ from the start of $e$ to the start of $Q$.
We claim that this new edge-labeled graph $G$ defines a mixing SFT $X$ and a factor map to $Y$ that admits a section $\phi$ with $\phi(Z)$ being the copy of $Z$ we added by hand.
Take any path $v$ in $G$; we claim that its labeling is in $L(Y)$.
We may assume that the labeling begins and ends with $s$ by extending $v$ if necessary.
Since $s$ is a synchronizing word for $Y$, we may split the path into overlapping segments that start and end with $s$, and handle each segment separately.
The labeling of $G$ has the property that any path with label $s$ terminates in either $q$ or the $|s|$th vertex of $P$.
A segment that goes through $P$, then the copy of $Z$ and then $Q$ has a labeling of the form $s u a v b w s$ with $a v b \in L(Z)$, which we assumed are all in $L(Y)$.
A segment that does not go through $P$ is entirely inside $Y'$, and thus its labeling is in $L(Y)$.
Hence we have a factor map onto $Y$.
It is clear that $X$ is mixing (since $Y'$ is), and the section $\phi$ just maps $Z$ to the copy edge-by-edge. Square.
Corollary. The condition is decidable.
Proof. Use basic automata theory. Square.
As Ilkka's comment showed, for subshifts of almost finite type, the condition is equivalent to Fischer cover being an example (and that's decidable). The following example, taken from [1], shows that the Fischer cover may not admit a section even if some other factor map does.
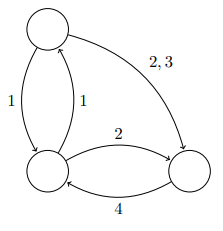
Namely, take the labels of this graph as $Y$ (so it's a mixing sofic shift), and pick $Z = \{1^{\mathbb{Z}}\}$. If we take $X$ the Fischer cover (that's what's shown in the picture), there is no preimage for the unique point in $Z$. However, it is easy to show that the above first-order condition holds.
We do not know how to extend the result to the case where $Z$ is only mixing sofic.
[1]: Ballier, Alexis, Limit sets of stable cellular automata, Ergodic Theory Dyn. Syst. 35, No. 3, 673-690 (2015). ZBL1355.37025.

