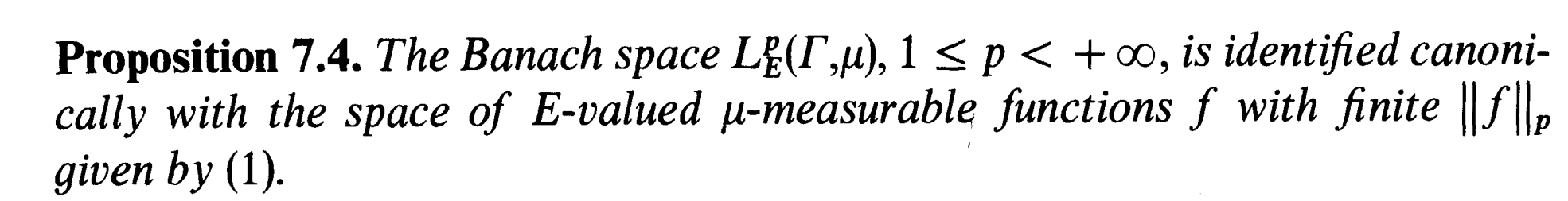As Narutaka says, there are two questions, one of measurability, and one about "custom". Yes, we should quotient by the null space. If you look at page 254 in Takesaki, then this is done (see just after displayed equation (1)). It is only the subsequent Proposition 7.4 which is stated in a slightly "lazy" way.
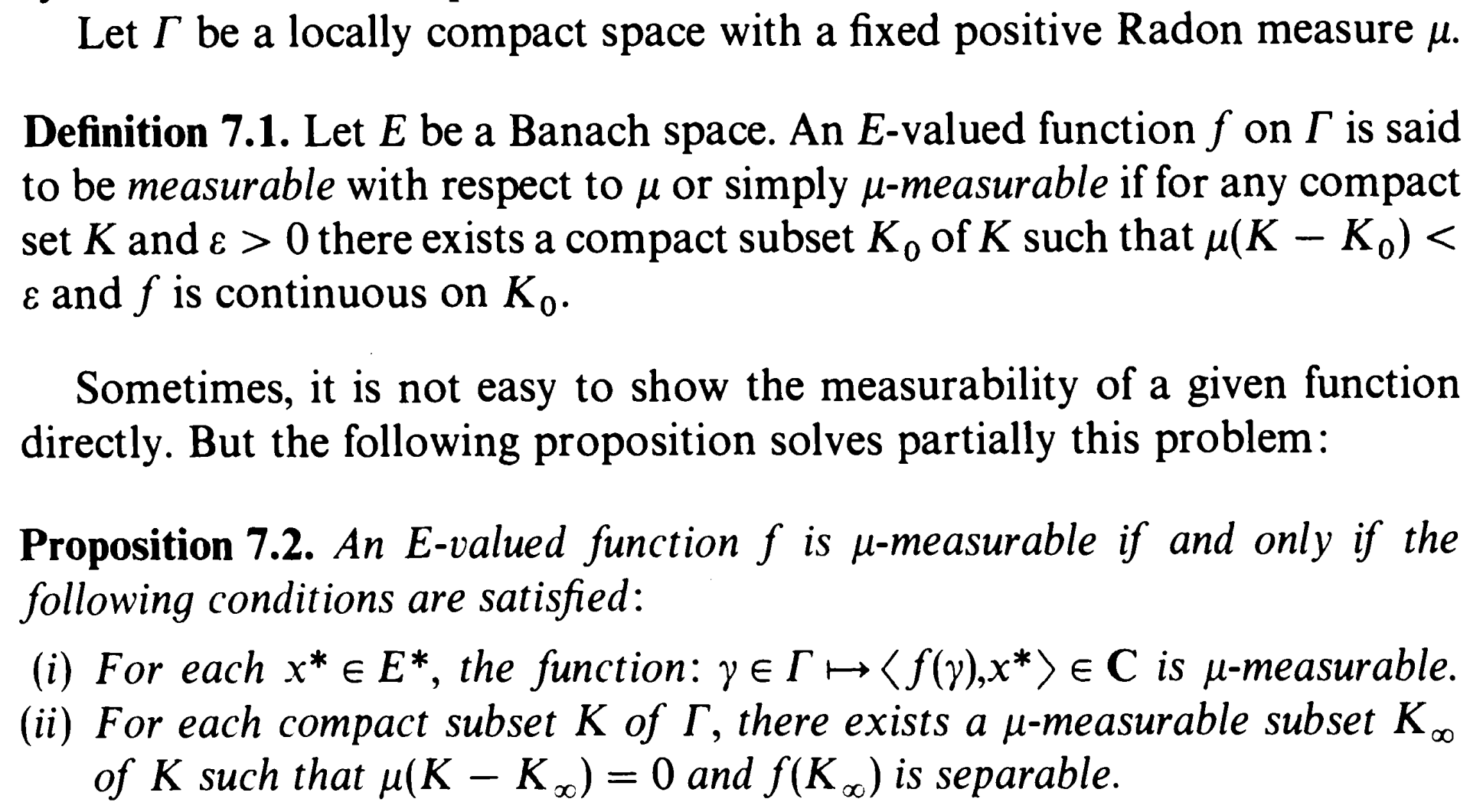
Now on to measurability. Definition 7.1 should remind us of Lusin's Theorem that up to an $\epsilon$ of measure, measurable functions (in this setting of Radon measures) agree with continuous functions.
Further, Proposition 7.2 should remind us of the Pettis measurability theorem. This means that Takesaki's definition exactly agrees with the usual notion of Strong Measurability of a vector-valued function (the almost-everywhere limit of a sequence of simple vector-valued functions) found when considering Bochner integration.
(My go-to reference is Diestel, Uhl, Vector Measures, zbmath, but this can be found in lots of books, almost anywhere vector-valued integration is considered.)
The slight problem is that Takesaki's definitions are all with respect to a compact set, instead of the whole space. But we conclude immediately that if $\Gamma$ is compact, then Proposition 7.2 shows that this notion of vector-valued integration is exactly the usual Bochner one. With a touch more work, the $\sigma$-compact case follows.
What about for general $\Gamma$? Here I again follow Cohn's Measure Theorem, Chapter 7, specifically Section 7.5. We need to work not with Borel sets, but with the large $\sigma$-algebra of all measurable sets: what Cohn calls $\mathcal{M}_{\mu^\ast}$. Then the measure is decomposable, with respect to a family of compact sets, see Proposition 7.5.3 in Cohn. The result now follows.
Finally, can we work directly with Takesaki's Definition 7.1? Yes, because for $\mathcal{M}_{\mu^\ast}$, Lusin's Theorem has a converse: this is Exercise 7.5.2 in Cohn. This shows exactly that a scalar-valued function satisfying Takesaki's Definition 7.1 is $\mathcal{M}_{\mu^\ast}$-measurable. As $\|\cdot\|$ is continuous, it is now immediate that if $f$ satisfies Takesaki's Definition 7.1 then $x\mapsto \|f(x)\|$ will be $\mathcal{M}_{\mu^\ast}$-measurable and hence its integral makes sense.
A minor personal grumble with Takesaki is that I am never quite sure what exactly he means by "Radon measure" for example. I think there is no reference given? I think this approach to measurability, as a sort of converse to Lusin's Theorem, is what Bourbaki does, but I am not super-familiar with Bourbaki.