At a workshop it was suggested that it likely remains an open problem
whether or not there is a 3- or 2 -piece
dissection
of a square to an equilateral triangle.
Can anyone confirm that this is unresolved?
Four-piece dissections are known, the most famous being
Henry Dudeney's century-old gem:
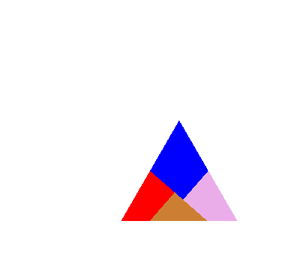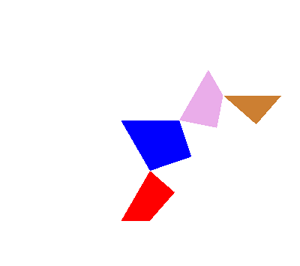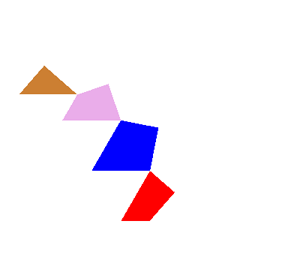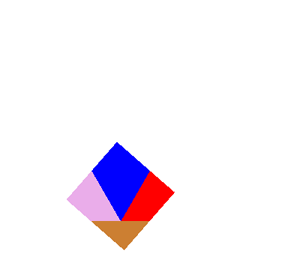
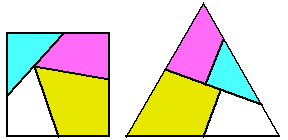
[Maple animation from this
link.]
Update (6Dec2024). Now proved impossible. "Dudeney's Dissection is Optimal." Erik D. Demaine, Tonan Kamata, Ryuhei Uehara. arXiv abs.
