16
$\begingroup$

At a workshop it was suggested that it likely remains an open problem whether or not there is a 3- or 2 -piece dissection of a square to an equilateral triangle. Can anyone confirm that this is unresolved?

Four-piece dissections are known, the most famous being Henry Dudeney's century-old gem:

                [Maple animation from this link.]

Update (6Dec2024). Now proved impossible. "Dudeney's Dissection is Optimal." Erik D. Demaine, Tonan Kamata, Ryuhei Uehara. arXiv abs.

Improve this question
$\endgroup$
7
  • 15
    $\begingroup$ 2 is not possible because the side length of the triangle is more than the diagonal of the square. $\endgroup$
    – Ken Fan
    Commented Nov 5, 2011 at 2:18
  • 6
    $\begingroup$ I just want to tell that applet to "hold still, dammit"! $\endgroup$
    – Todd Trimble
    Commented Nov 5, 2011 at 13:07
  • 5
    $\begingroup$ @Todd: Added a stable image (in a different orientation). $\endgroup$
    – Joseph O'Rourke
    Commented Nov 5, 2011 at 13:40
  • 4
    $\begingroup$ A 3-piece dissection of the equilateral triangle would have to create 4 right angles to serve as corners of the square, and there are just a few ways this can be done. At first glance, none of them recombine as a square (though you can get a rectangle). It should be pretty easy to run through the options and rule them all out. $\endgroup$
    – Anton Lukyanenko
    Commented Nov 6, 2011 at 18:34
  • 4
    $\begingroup$ I comment only to point out that the so-called Dudeney construction may not, in fact, be due to H. Dudeney. For more details, see G.N. Frederickson's "Hinged Dissections: Swinging and Twisting" (pp. 8-10). books.google.com/… $\endgroup$
    – Benjamin Dickman
    Commented Nov 28, 2013 at 6:04

1 Answer 1

Reset to default
4
$\begingroup$

In the paper, "Dissection with the Fewest Pieces is Hard, Even to Approximate" (arXiv, doi) by Bosboom et al., they write:

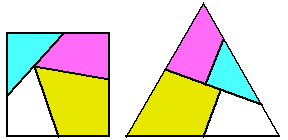
We have known for centuries how to dissect any polygon $P$ into any other polygon $Q$ of equal area, that is, how to cut $P$ into finitely many pieces and re-arrange the pieces to form $Q$. But we know relatively little about how many pieces are necessary. For example, it is unknown whether a square can be dissected into an equilateral triangle using fewer than four pieces. [emphasis added]

The authors seem to be knowledgeable about the subject, so I'd be inclined to trust their claim. As I mentioned in another MO answer, they point out that more generally, it is not known if "$k$ piece dissection," the problem of deciding whether two given polygons (say with rational vertices) admit a common dissection into at most $k$ pieces, is decidable. The trouble is that even if you restrict yourself to cuts that are piecewise-linear, there is no known a priori upper bound on how many "zigs and zags" a cut might have to take.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .