The answerThis is not an answer but an extended comment to indicate that the answer would be no without the simplicity or convexity assumptions. First observe that there are curves in $\textbf{R}^2$ with exactly $4$ critical points of curvature which self-intersect an unlimited number of times, as shown for instance in the picture below.
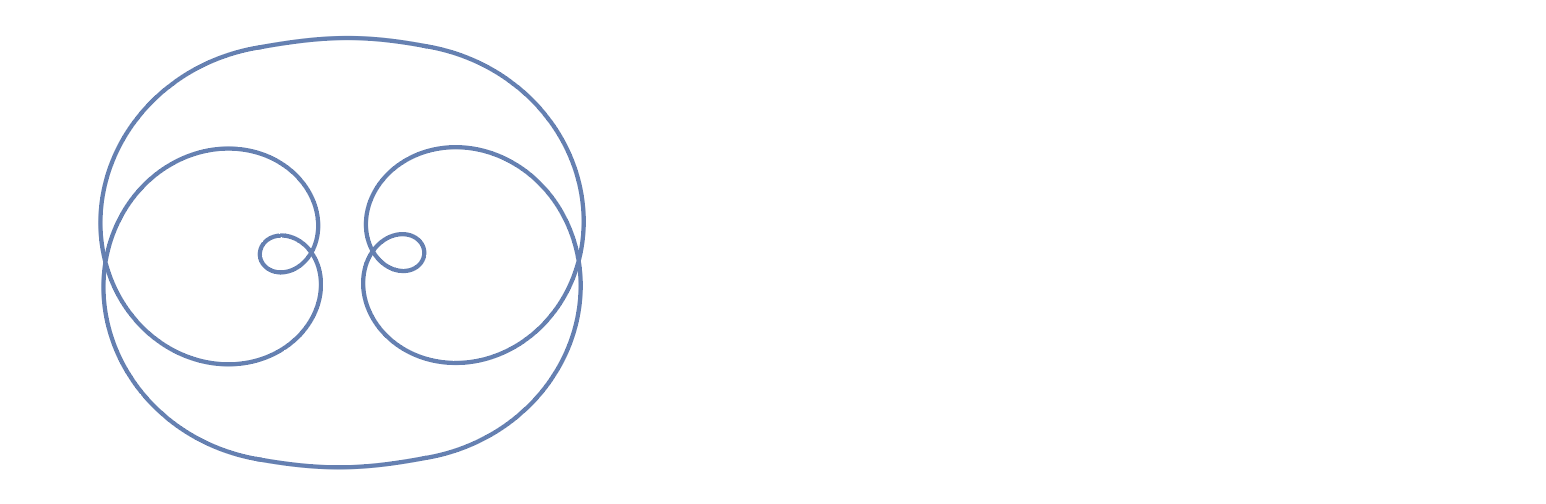 Pull this curve to $\mathbf{S^2}$ via stereographic projection, which will preserve critical points of curvature. So we obtain a spherical curve whose torsion changes sign exactly $4$ times and intersects some plane more than $4$ times. AThis example is convex but not simple. A perturbation of it yields a simple closed curve in $\mathbf{R}^3$ which still has exactly $4$ vertices butand intersects some plane more than $4$ times, but this perturbation will not preserve convexity.
Pull this curve to $\mathbf{S^2}$ via stereographic projection, which will preserve critical points of curvature. So we obtain a spherical curve whose torsion changes sign exactly $4$ times and intersects some plane more than $4$ times. AThis example is convex but not simple. A perturbation of it yields a simple closed curve in $\mathbf{R}^3$ which still has exactly $4$ vertices butand intersects some plane more than $4$ times, but this perturbation will not preserve convexity.
