Return to Revisions

The answer is no. First observe that there are curves in $\textbf{R}^2$ with exactly $4$ critical points of curvature which self-intersect an unlimited number of times, as shown for instance in the picture below. 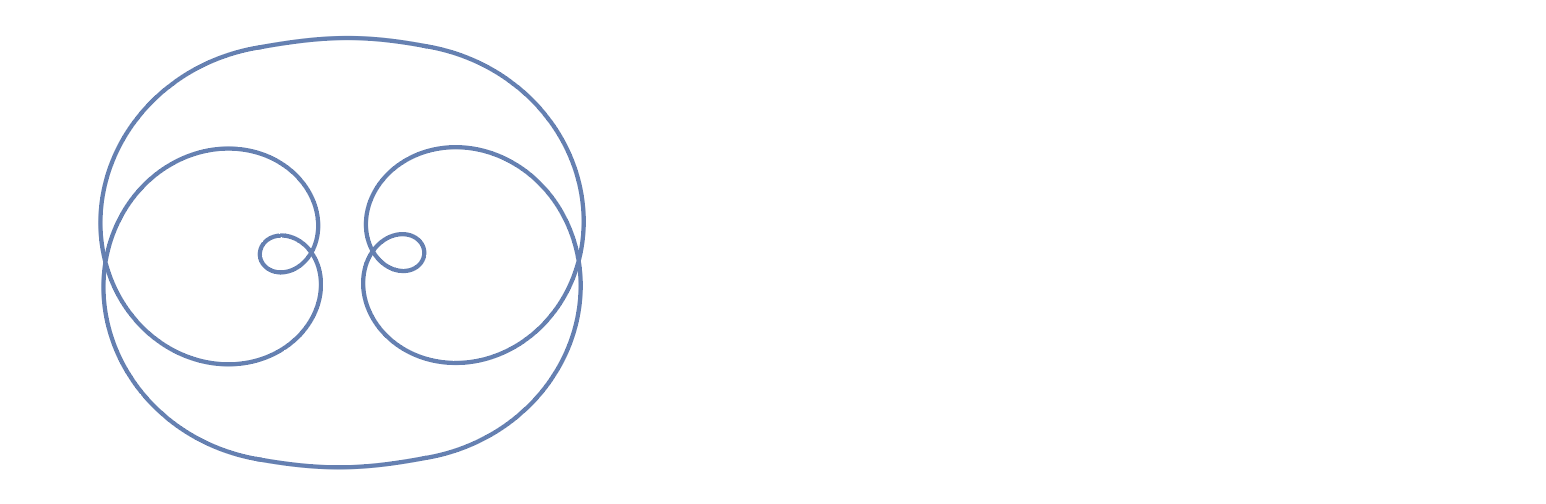Pull this curve to $\mathbf{S^2}$ via stereographic projection, which will preserve critical points of curvature. So we obtain a spherical curve whose torsion changes sign exactly $4$ times. Perturbing this curve we obtain a simple closed curve in $\mathbf{R}^3$ which has exactly $4$ points of vanishing torsion but intersects some plane more than $4$ times.