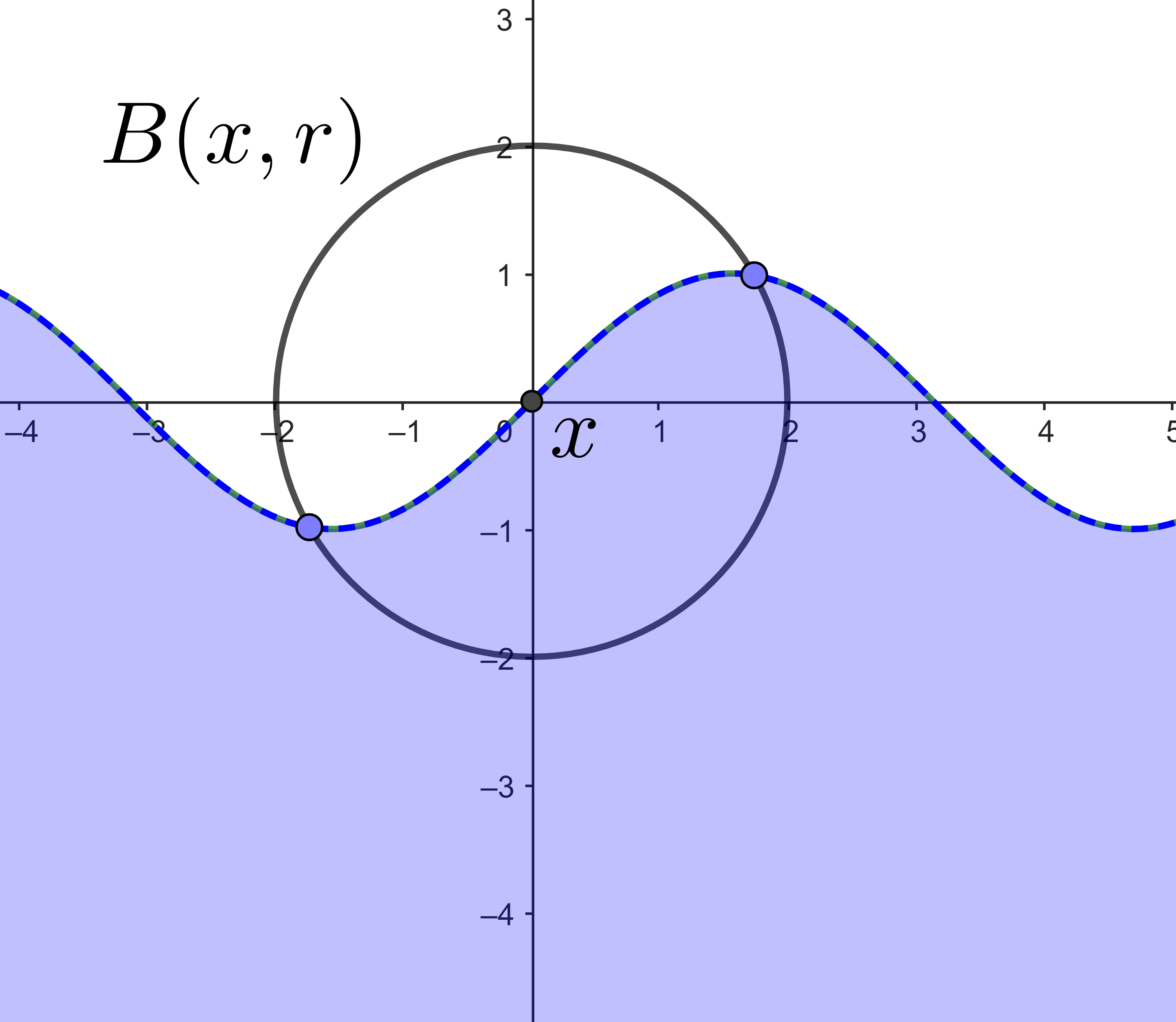
Let $\Omega\subset\mathbb{R}^N$ be an open, bounded and connected Lipschitz domain. Is it true that we can find some $R>0$ such that any $N$-dimensional open ball $B(x,r)$ with $r\leq R$ that intersects $\Omega$ has the property that $\Omega\cap B(x,r)$ is a open, bounded and connected Lipschitz domain too?
I don't know any reference that specify those facts and I decided to ask here.
EDIT: I look at the solutions and see that the problem is that we lose connectedness.
Question: If the intersection between an open ball and a Lipschitz domain is connected, then we can say that the intersection is a Lipschitz domain?