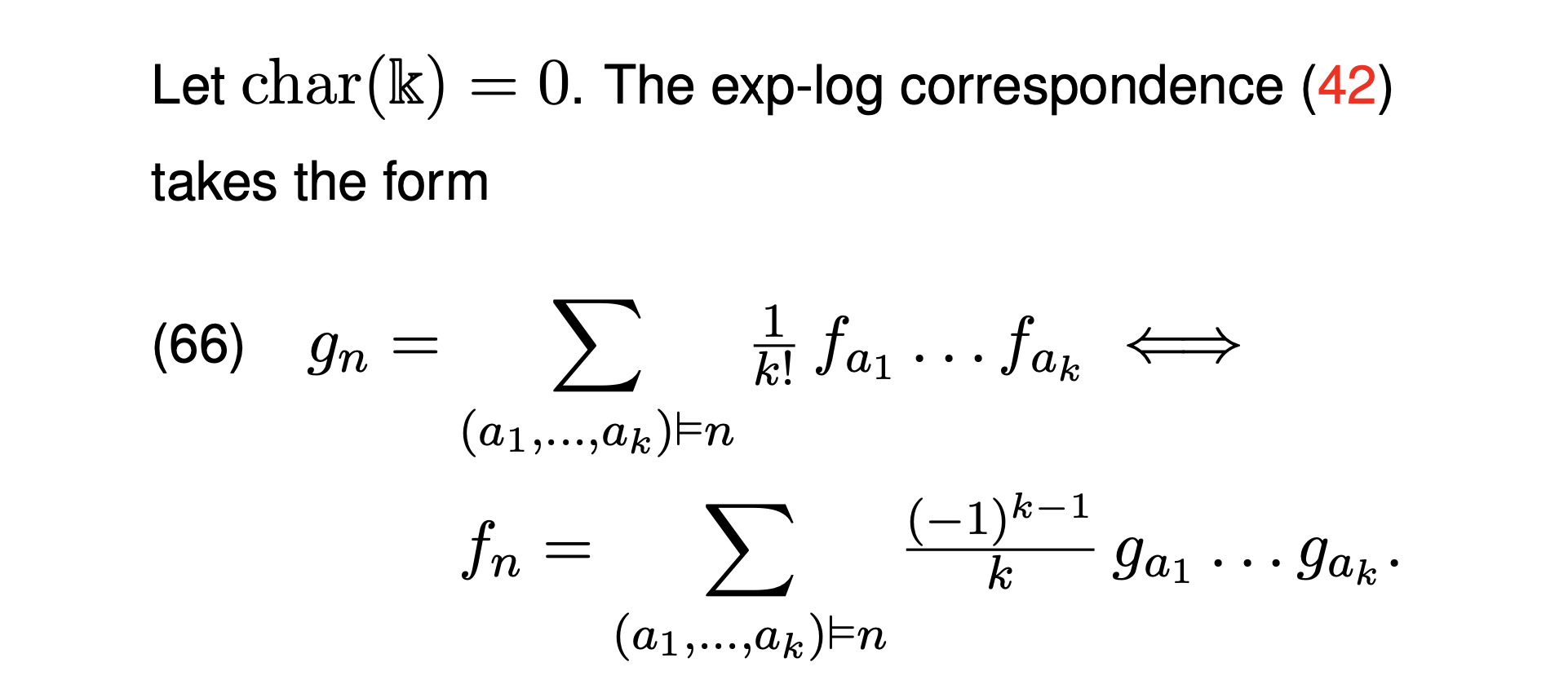I've encountered several identities in combinatorics that resemble inversion formulas, as showshown below,
Here, $f_i, g_k \ \forall i,k \in \mathbb{N}$$f_i, g_k$, $\forall i,k \in \mathbb{N}$, are coefficients of some formal power series.
I noticed that the coefficients in these sums are in resemblance with coefficients in the power series of $ln(1+x), \exp{x} -1$$\ln(1+x), \exp{x} -1$, which suggests to me that they are related in some way to Lie Groupgroup - Lie Algebra Correspondencealgebra correspondence.
However, I have not been able to find any discuss any textbook(s) that deal with these identities in full generality. I even don't have a name for such 'classes' of identities.I I would really appreciate some resources that deal with this 'particular' form of identities.