By De Giorge, Nash and Moser solutions of
\begin{equation}
div (A(x) Du) = 0
\end{equation}\begin{equation}
\operatorname{div} (A(x) Du) = 0
\end{equation}
where $Du$ denotes the gradient of $u$ and $A$ is a $\lambda,\Lambda$ elliptic matrix. That is,
\begin{equation}
\lambda|\xi|^2\le\langle A_\pm(x)\,\xi,\xi\rangle \le \Lambda|\xi|^2,
\end{equation}
for constants $0< \lambda \le \Lambda$ can be only Hölder continuous. So the classical Strong Maximum Principlestrong maximum principle, see for instance Fanghua Lin,
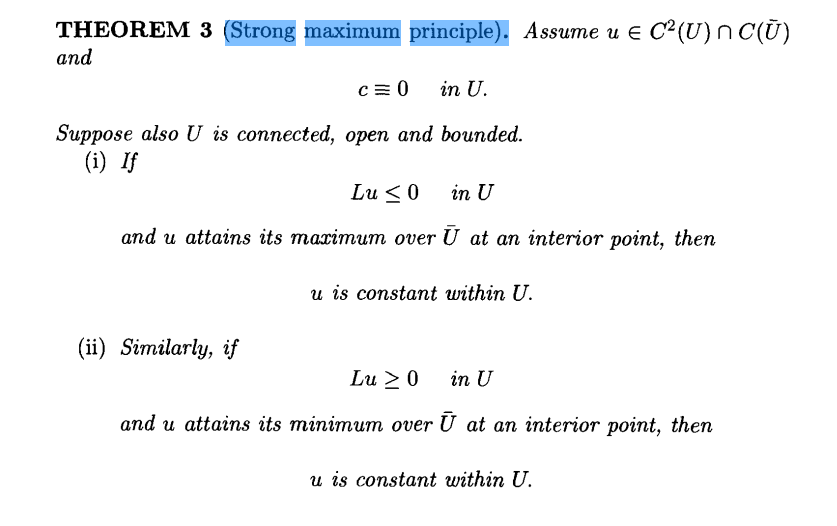 can not be applyed because the solutin can not be sufficiently smooth. If the equation
can not be applyed because the solutin can not be sufficiently smooth. If the equation
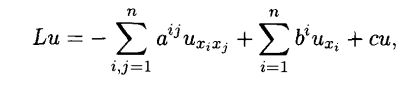
was satisfyied only in the weak sense, the SMP istill holds?
I know that already exists similar questions here but the different context difficult the undertand.
