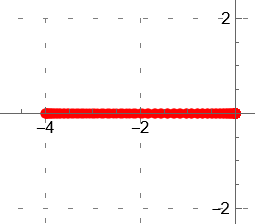
For $n\geq 1$, given a polynomial \begin{equation*} \begin{aligned} f(x)=&\frac{2+(x+3)\sqrt{-x}}{2(x+4)}(\sqrt{-x})^n+\frac{2-(x+3)\sqrt{-x}}{2(x+4)}(-\sqrt{-x})^n \\ &+\frac{x+2+\sqrt{x(x+4)}}{2(x+4)}\left ( \frac{x+\sqrt{x(x+4)}}{2} \right )^n+\frac{x+2-\sqrt{x(x+4)}}{2(x+4)}\left ( \frac{x-\sqrt{x(x+4)}}{2} \right )^n. \end{aligned} \end{equation*} Using Mathematic $12.3$, when $n$ is large enough, we give the distribution of the roots of $f(x)$ in the complex plane as follows
In this figure, we can see that the closure of the real roots of $f(x)$ may be $\left [ -4,0 \right ]$.
So we have the following questionsquestion
Question 1: all roots of $f(x)$ are real? It seems yes! But we have no way of proving it.
Question 2: How to prove the closure of the real roots of $f(x)$ is $\left [ -4,0 \right ]$?