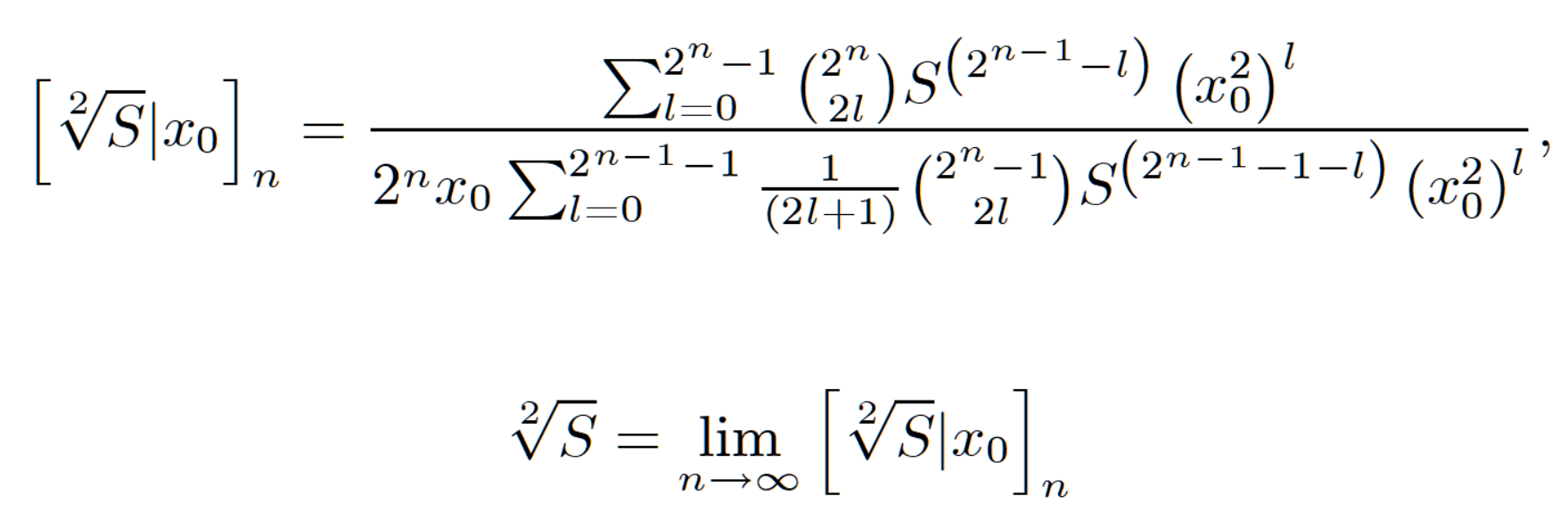I found that the square root of any prime number S can be approximated, at the n-th order, as a rational number represented by the polynomials shown below. x_0$x_0$ is an initial seed, which is a rational number chosen “close” to \sqrt{S}$\sqrt S$. I used the iterative method, not the continued fractions
My question: Is someone aware of this result? Is it already known and has been published elsewhere? If affirmative, please post the reference!