Where did you get this? I think it is wrong for most values ofIf $b$. Here is$l \ne m$, then $$ \int_0^b x J_0(x) J_0(2x)\;dx $$$$ \int_{0}^{b}\!x{{\rm J}_{a}\left(lx\right)}{{\rm J}_{a}\left(mx\right) }\,{\rm d}x={\frac {b \big( {{\rm J}_{a+1}\left(lb\right)}\;{{\rm J}_{a }\left(mb\right)}\;l-{{\rm J}_{a+1}\left(mb\right)}\;{{\rm J}_{a}\left(lb \right)}\;m \big) }{{l}^{2}-{m}^{2}}} $$
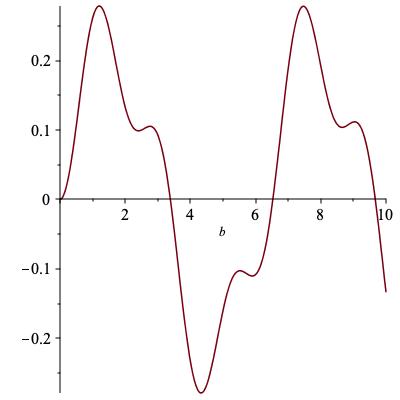 Assume $l,m$ are zeros of $J_a$ and $b=1$. Then we get the value $0$.
Assume $l,m$ are zeros of $J_a$ and $b=1$. Then we get the value $0$.
