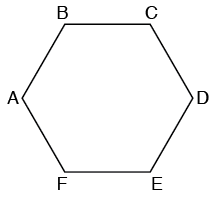
Let M$\DeclareMathOperator\SU{SU}$Let $M$ be a coadjoint orbit of dimension 6 of $SU(3)$$\SU(3)$, and let T$T$ be the maximal torus in $SU(3)$$\SU(3)$. If we denote $\mu : M \longrightarrow \mathbb{R}^2$ the moment map associated to the action of T$T$ on M$M$, then the image of the moment map is a hexagon with vertices A$A$, B$B$, C$C$, D$D$, E$E$, F are image$F$ the images of the elements $M^T$ by $\mu $.
For $P \subset \mathfrak{t}^*$ an affine space with vectorielvectorial direction $\overrightarrow{P}$, let $P^\perp := \lbrace \xi \in \mathfrak{t}, \langle y, \xi \rangle =0, \forall y \in {(\overrightarrow{P})}^\perp\rbrace $$P^\perp \mathrel{:=} \lbrace \xi \in \mathfrak{t} \mathrel| \langle y, \xi \rangle =0, \forall y \in {(\overrightarrow{P})}^\perp\rbrace $, and let $T_P$ be the sub-torus generated by $Exp(P^\perp)$$\operatorname{Exp}(P^\perp)$.
If $\Sigma := \lbrace$ P convex polytope in $\mathfrak{t}^* |\exists Z $ connected component of $M^{T_P}$ s.t $ \mu (Z)= P\rbrace $$\Sigma \mathrel{:=} \lbrace \text{$P$ convex polytope in $\mathfrak{t}^*$} \mathrel| \exists \text{$Z$ connected component of $M^{T_P}$ s.t $ \mu (Z)= P$}\rbrace$, how can I prove that $\Sigma = \lbrace $ faces of $ \mu (M)\rbrace \cup\lbrace [AD],[BE], [FC]\rbrace ? $
I have no idea how to deal with this, your help would be greatly appreciated!$\Sigma = \lbrace\text{faces of $ \mu (M)$}\rbrace \cup\lbrace [AD],[BE], [FC]\rbrace$?