Return to Question

Big update after comment by I.B.

Source Link

edited Nov 20, 2019 at 18:26

13.3k
3
48
85

-----------Update after comment by Ilya Bogdanov-------

Well, Ilya Bogdanov provided an example which gets more to the heart of the matter. So I'll flip over my cards and describe a real conjecture.

Conjecture: Suppose that $f \in {\mathbb Q}(x)$ has no poles in the closed interval $[0,1]$. Then the sequence $(\Xi^n f)$ stabilizes for $n \geq N$, where $N$ can be bounded in terms of the degrees of numerator and denominator of $f$ together with the heights of the coefficients of $f$ (in reduced form).

This is what I had in mind asking the question... trying to figure out how to quantifiably estimate how the sequence $(\Xi^n f)$ behaves (number of zeros, etc.) An effective proof of the conjecture would give new estimates on irrationality measures of logs of algebraic numbers, I think. And it fits nicely as a simple case of the Kontsevich-Zagier conjecture about periods.

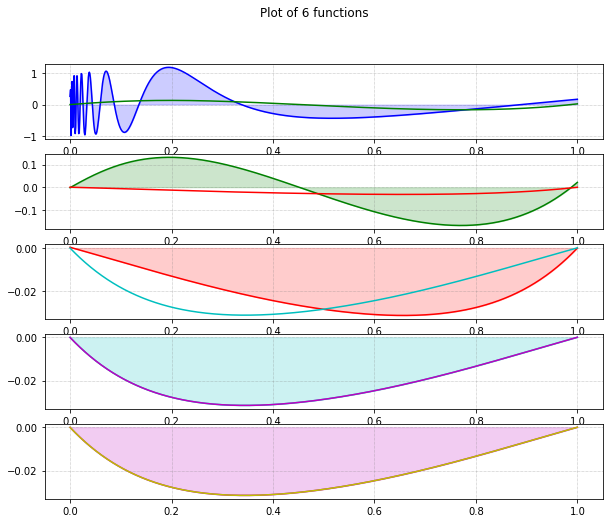
Ilya Bogdanov drives right to the simplest interesting case, which seems important to understand. First is the sequence of graphs for $1/(1+x) - 0.7$ (a function in ${\mathbb Q}(x)$). $1/(1+x) - 0.7$ after applying $\Xi^n$." />

You can see that just a few iterations suffices to show that $\log(2) < 0.7$.

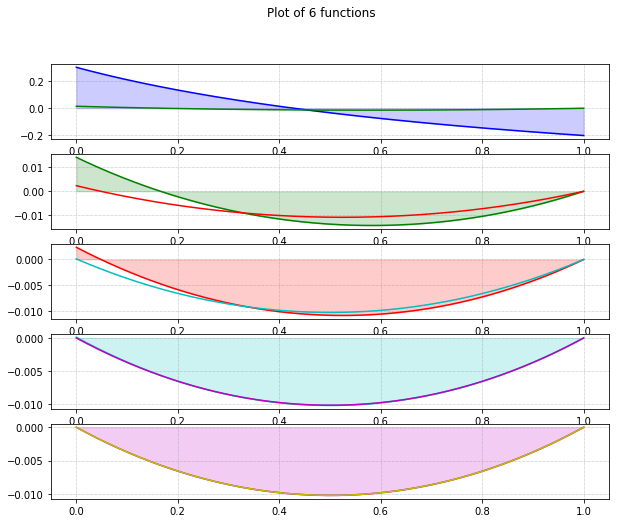
Next is the sequence of graphs for $1/(1+x) - \log(2)$ (using ordinary floating point operations).
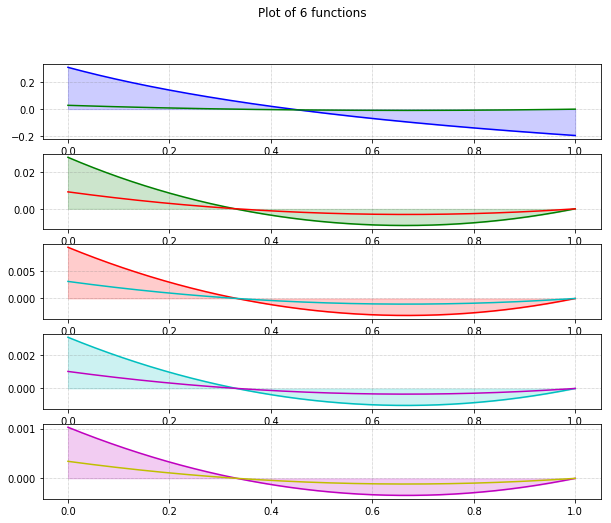$1/(1+x) - \log(2)$ after applying $\Xi^n$." />

You can see that the graph crosses the x-axis, through all of these iterations, as Ilya Bogdanov suspected. It doesn't go against my conjecture, but it does suggest that something beyond a bit of analysis of real-analytic functions is required. Also, note the changes in the y-axis in the above sequence of graphs... it seems that each graph gives about another "bit" in the approximation game.

-----------Update after comment by Ilya Bogdanov-------

Well, Ilya Bogdanov provided an example which gets more to the heart of the matter. So I'll flip over my cards and describe a real conjecture.

You can see that just a few iterations suffices to show that $\log(2) < 0.7$.

Next is the sequence of graphs for $1/(1+x) - \log(2)$ (using ordinary floating point operations).
$1/(1+x) - \log(2)$ after applying $\Xi^n$." />

Source Link

asked Nov 19, 2019 at 22:51

Marty

13.3k
3
48
85

Comparing an integral to zero, by slicing and stacking

Let $f \colon [0,1] \rightarrow {\mathbb R}$ be a nice function -- real-analytic, or maybe definable in some o-minimal structure, let's say. Let $0 < \alpha_1 < \cdots < \alpha_n < 1$ be its roots in the open interval $(0,1)$. Define $\alpha_0 = 0$ and $\alpha_{n+1} = 1$ for convenience. For $0 \leq j \leq n$, let $w_j = \alpha_{j+1} - \alpha_j$. So $sgn(f(x))$ is constant on the intervals $(\alpha_j, \alpha_{j+1})$, which have widths $w_j \leq 1$.

For all $0 \leq j \leq n$, define a new function $f_j \colon [0,1] \rightarrow {\mathbb R}$ by the formula $$f_j(x) = w_j \cdot f( w_j x + \alpha_j),$$ or $$f_j(x) = w_j \cdot f( \alpha_{j+1} - w_j x),$$ according to whether $j$ is even or odd.

These are cooked up so that $$\int_0^1 f_j(x) dx = \int_{\alpha_j}^{\alpha_{j+1}} f(x) dx.$$ (The whole parity dependence is cooked up so that some pointy bits line up in the end.)

Define $\Xi f(x) = \sum_{j=0}^n f_j(x)$, because the letter $\Xi$ looks like a bunch of slices. Thus $$\int_0^1 f(x) dx = \int_0^1 \Xi f(x) dx.$$

It seems, from a few experiments, that repeating this process eventually (typically quickly) leads to a function $F(x) = \Xi^k f(x)$ for which $F(x) \geq 0$ for all $x \in [0,1]$ or for which $F(x) \leq 0$ for all $x \in [0,1]$. Then it becomes trivial to compare the integral $\int_0^1 f(x) dx = \int_0^1 F(x) dx$ to zero.

I'd like to prove this, at least for a nice class of functions. To put my cards on the table, I'd like to prove this for rational functions (without poles on the interval) with some explicit bounds on how many iterations are necessary.. because that might give results related to Baker's Theorem, decidability of periods in the simplest cases, etc.

If you like pictures, here's the process, exhibited for the function $f(x) = x - sin \left( \frac{1}{x + 0.02} \right)$. I just wanted something wiggly :) The top graph shows the function $f$ (with area shaded between $f(x)$ and the x-axis), and the graph of $\Xi f$. Then $\Xi f$ and $\Xi^2 f$ in the graph below. Etc. Stabilization occurs when the graph of $\Xi^k f$ (on $(0,1)$) is always above or always below the x-axis.