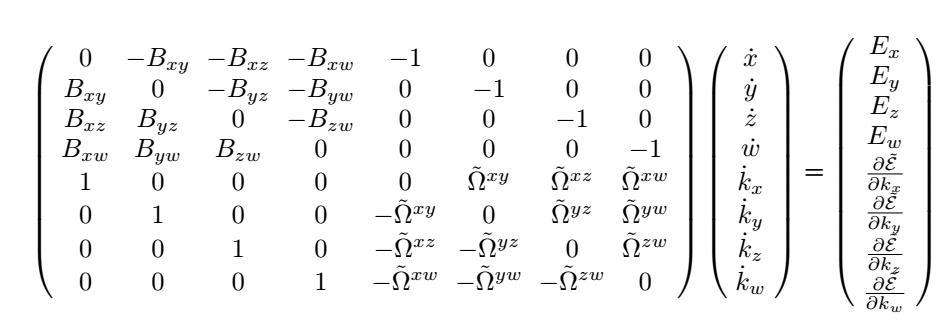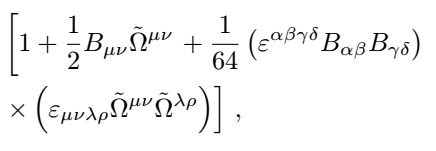I want to show that the square root of the determinant of the following antisymmetric matrix
is given by
I saw this equation in the paper Supplemental Material of Four-Dimensional Quantum Hall Effect with Ultracold Atoms, but it didn't provide a specific deduction. So I wanna know how to deduct the square root of the determinant of this antisymmetric matrix is that formula showed in Fig.2.
Fig.2 The Square Root of the Determinant of the 8-order Antisymmetric Matrix
I have tried Mathematica and Matlab to calculate the determinant directly. But as for Mathematica, the processing time is too long to wait. And though Matlab could give the result very fast but it couldn't show them all...Because because the result is too long...Thus Thus, I guess the best way to solve this problem might be mathematical deduction rather than computer. After all the matrix is antisymmetric,and and it's quite special in many aspects.