Background

Martin Hairer gave recently some beautiful lectures in Israel on "taming infinities," namely on finding a mathematical theory that supports the highly successful computations from quantum field theory in physics.

(Here are slides of a similar talk at Heidelberg. and a video of a related talk at UC Santa Cruz.)

I think that a relevant paper where Hairer's theory is developed is : A theory of regularity structures along with later papers with several coauthors.

Taming infinities

Quantum field theory computations represent one of the few most important scientific successes of the 20th century (or all times, if you wish) and allow extremely good experimental predictions. They have the feature that computations are based on computing the first terms in a divergent series, and a rigorous mathematical framework for them is still lacking. This issue is sometimes referred to as the problem of infinities.

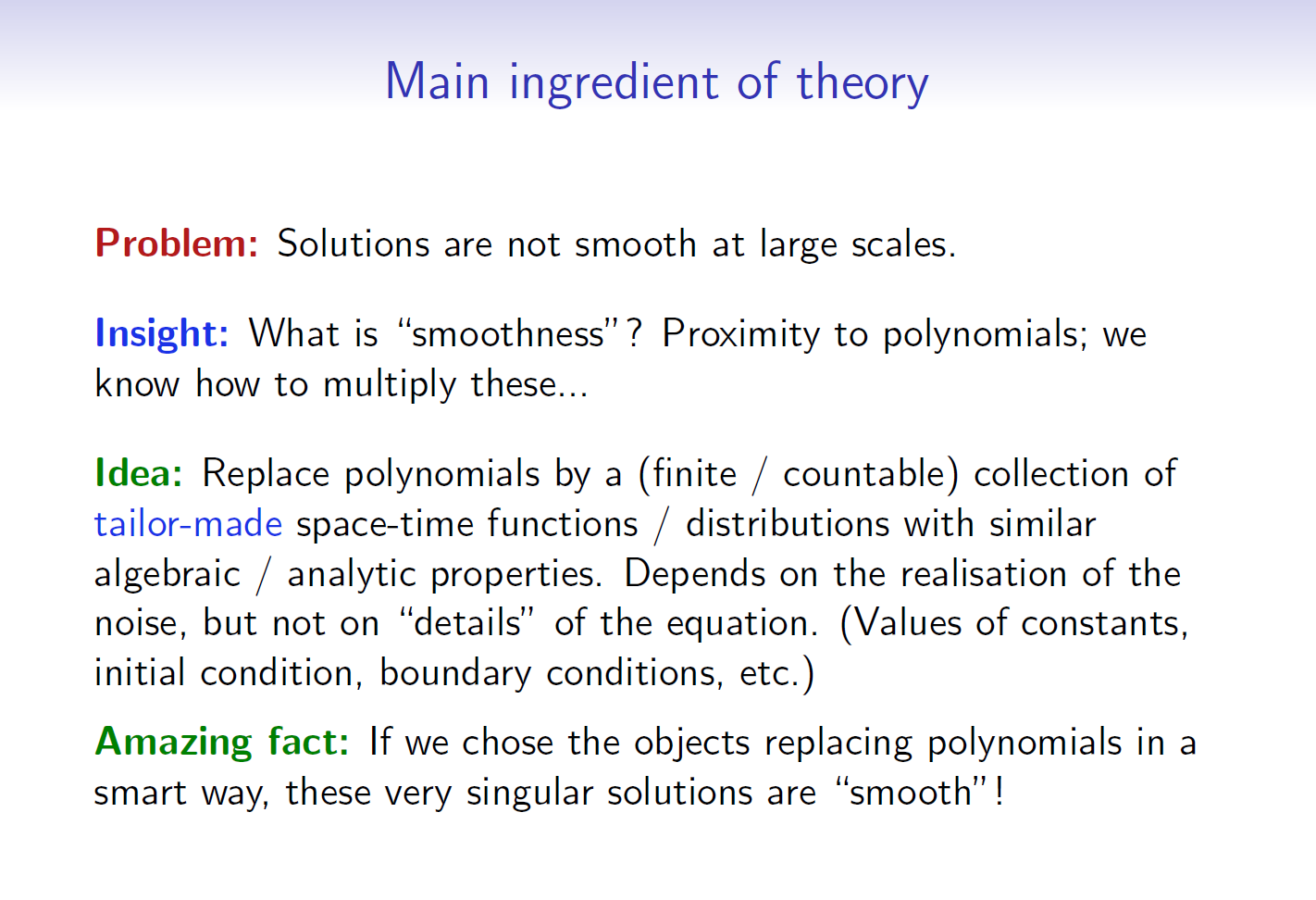
Here is one relevant slide from Hairer's lecture about the problem.

And here is a slide about Hairer's theory.

The Question

My question is for further introduction/explanation of Hairer's theory.

1) What are these tailor-made space-time functions?

2) What is the role of noise?

3) Can the amazing fact be described/explained in little more details?

4) In what ways, does the theory provides a rigorous mathematical framework for renaormalization and to physics' computations in quantum field theory.

5) How is Hairer's theory compared/related to other mathematical approaches for this issue. (Renormalization group, computations in quantum field theory, etc.)