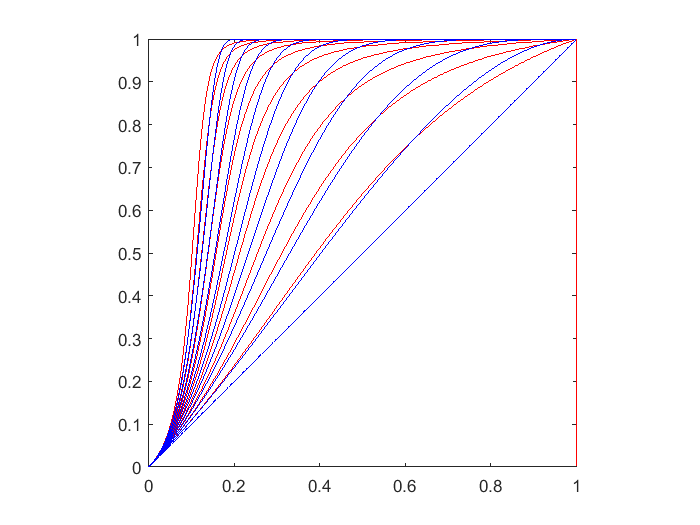
Let $f(x) := x^2 + (1-x^2)x$ and $F(x) := \log \frac{x}{1-x}-\frac{1}{x}$. It can be shown (cf. http://math.stackexchange.com/questions/1865370/https://math.stackexchange.com/questions/1865370/) that $F$ is approximately equivariant w/r/t the $\mathbb{Z}$-actions corresponding to iteration $f^{\circ N}(x)$ and addition $x+N$. That is, we have that $F(f^{\circ N}(x)) \approx F(x)+N$. This is shown in the figure below: blue curves are $f^{\circ N}(x)$ and red curves are $F^{-1}(F(x)+N)$, both for $0 \le N \le 10$.
More generally (but not generally enough to answer the question below), if we have a generic nice bijection $f$ and define $F := \int \frac{dx}{f(x)-x}$, then (waving away the distinction between a function and an antiderivative) $F(f^{\circ N}(x)) \approx F(x)+N$.
Is this approximate equivariance a manifestation of some more general phenomenon?