I have a question about a simple proposition, I suppose that this is something well-known or a special case of something well-known:
Let $D\subset\mathbb{R}^{2}$ be the closed unit disk in the plane and $f:D\rightarrow\mathbb{R}$ be a smooth function. Suppose that the restriction $f|_{\mathbb{S}^{1}}$ has exactly $n$ local extrema, but none of them is a local extremum of $f$. Then the function $f$ has at least $\frac{n}{2}+1$ local extrema inside $D$.
Note that it follows from the condition that the level line of $f$ at a local extremum of $f|_{\mathbb{S}^{1}}$ touches the boundary from inside. Some examples are given below as level lines diagrams.
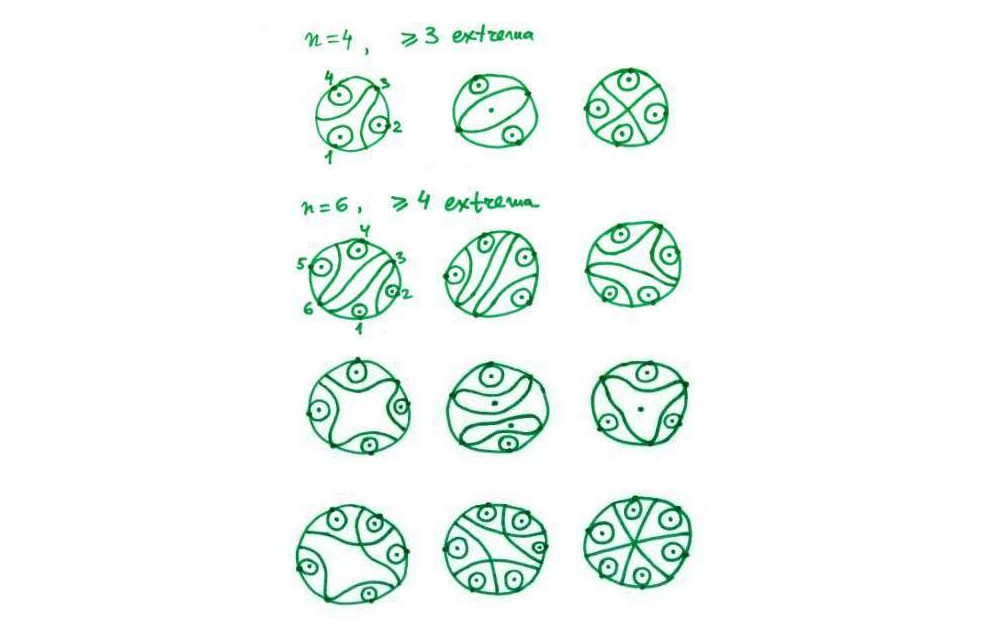
Here closed level lines correspond to local extrema of $f$ and crossing points of level lines correspond to saddles (possibly degenerated). As the condition is simple and clear and the proof is quite elementaryelementary*, I intend to propose this at a student competition. However, if this is well-known or folklore, it might not be suitable to propose it, so any references are welcome.
*/ In fact I have an elementary proof of a weaker form of the statement, claiming the existence of at least $\frac{n}{2}+1$ critical points of $f$ inside $D$.
Remarks. 1) This proposition may be completed by adding a second part: Let $\varphi:\mathbb{S}^{1}\rightarrow\mathbb{R}$ be a smooth function with $n$ local extrema, then it may be extended to a smooth $f:D\rightarrow \mathbb{R}$ satisfying the above conditions with exactly $\frac{n}{2}+1$ local extrema (and thus lying in the interior of $D$). Presumably this is true, but I don't have a proof.
- For a given even $n$, we may ask about the number of (topologically) different diagrams up to symmetry (rotation, reflection) with exactly $\frac{n}{2}+1$ local extrema and without other critical points; but it seems to be a very hard task.
