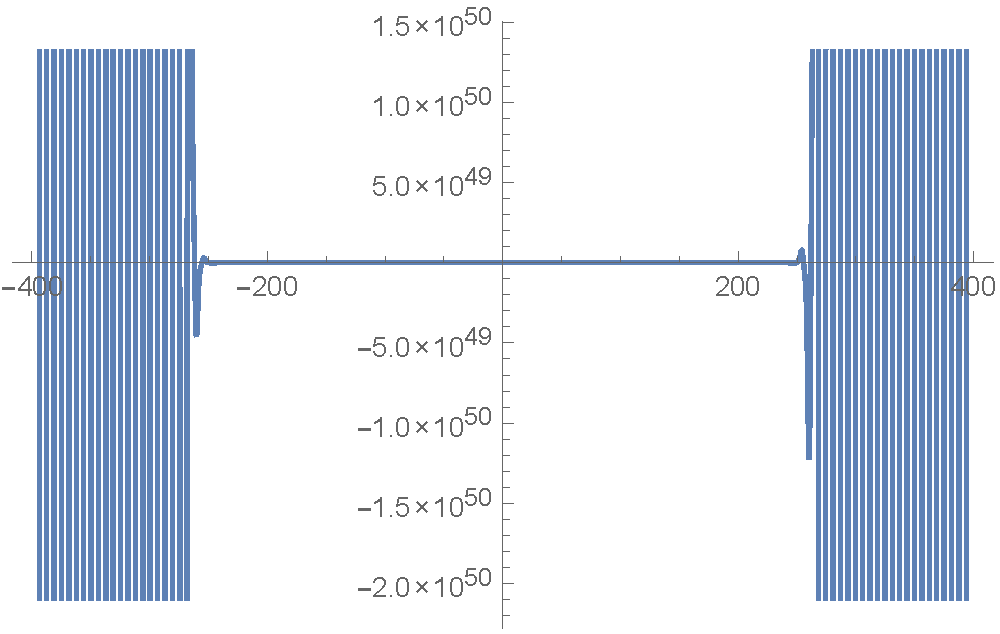
Consider the ODE $y^{\prime \prime}(x) = \cos(x) y(x),$$y^{\prime \prime}(x) = \cos(x) y(x)$ with boundary value conditions $y(0)=1, y(1)=2.$$y(0)=1$, $y(1)=2$. Solving it results in a linear combination of Mathieu functions, but what I find more interesting is its graph. So, the question is (a) is there really a phase transition around 300 (b) what is the envelope of the graph (that is,questions are the maxima/minima growing exponentially:
Is there really a phase transition around 300?
What is the envelope of the graph? That is, are the maxima/minima growing exponentially, or does something else occur?
Is the oscillating part actually periodic (which one would guess from the cos term)?
In general, or?) Is the oscillating part actually periodic (which one would guess from the cos term)? And what qualitative methods are there for answering these questions (that is,? I am sure the actual Mathieu function has been studied extensively, but just using its specific properties seems too "rigid").