The notion of derived polygon is natural and leads to remarkable convergence.
Start with a polygon, and replace it by locating a point on every edge
a fraction $\alpha$ between the two endpoints. For example, here is what happens for a random polygon of $8$ vertices and $\alpha=\frac{1}{4}$ (successive images rescaled):
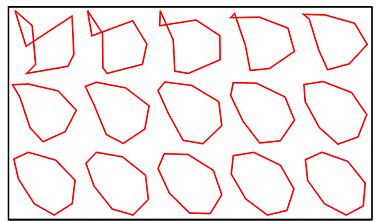
WikipediaMathWorld: "the derived polygons ... approach a shape with opposite sides parallel and equal in length, and all have the same centroid."
Now imagine generalizing this to polyhedra, let's say, convex polyhedra in $\mathbb{R}^3$ with triangular faces.
Start with a polyhedron $P$ of $F$ faces and $V$ vertices. For a given triple of positive reals $(\alpha,\beta,\gamma)$ that sum to $1$, locate a point $p$ in each face by
weighting the face's three vertices by $(\alpha,\beta,\gamma)$. (So if $\alpha=\beta=\gamma=\frac{1}{3}$, $p$ is the centroid of the face.) Now replace the original polyhedron
$P$ by the convex hull of the new points. Here is an example with $(\frac{1}{4},\frac{1}{2},\frac{1}{4})$ (again, each image rescaled):
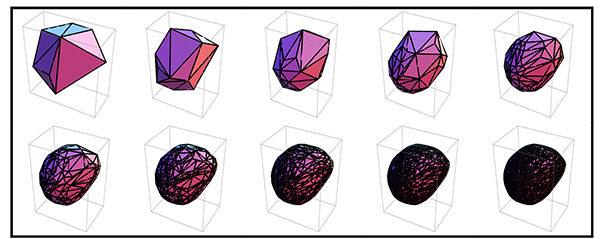
You can see what happens. At first $V=8$ and $F=10$, but next $V=10$ (because every face
generates a point), and by Euler's formula, $F=16$ ($F=2 V-4$). Etc.: the number of vertices and faces grows. Here are enlargements of the first and 10th polyhedra,
the latter of which has $1540$ vertices:

It seems that, despite the combinatorial growth in $\mathbb{R}^3$ absent in $\mathbb{R}^2$, it is likely the shape is converging to a limit that may bear
similarities to what can be established in 2D.
My question is:
Q. Has this process been studied before? If so, does the shape approach a limit largely independent of $(\alpha,\beta,\gamma)$, analogous to the situation in 2D? If so, what characteristics does this limit shape bear to the initial polyhedron?
