Seeing this image of Costa's minimal surface
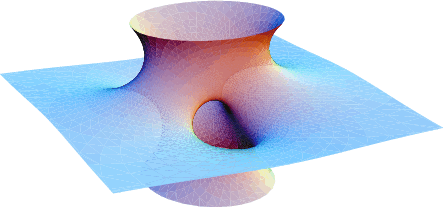
(MathWorld image)
made me wonder if the fine-grained structure
of the human lung is somehow composed of pieces of minimal surfaces?
I could not find an ideal image of a real lung;
apologies in advance if you find this offensive!
(Image link)
I searched around a bit without finding a connection. Which may mean none exists...
Does anyone know? Thanks!
I since found one possibly relevant quote in John Oprea's book *Differential Geometry and Its Applications*. (MAA, 2007), p.163:
![Oprea][5]
When the pressure difference is zero, the [Young-Laplace equation][6] leads to minimal surfaces.
