Let $g$ be a symmetric unimodal probability distribution and $H$ be the right half plane.
We call
$$f(z) = \int_{-\infty}^\infty \frac{1}{z-i t}g(t)dt$$
the dispersion function of $g$.
Now, one can relate $f$ to a variety of things; the Hilbert transform $g$, the Laplace transform of the associated characteristic function, etc. It has also appeared in coupled oscillator problems [here][1]here, [here][2]here and in my current work on coupled oscillators.
Let $L$ be a vertical line in the complex plane (without loss of generality, take the real part to be $1$) and consider the mapping $L\rightarrow f(L) \rightarrow 1/f(L)$ (see figure).
![The mappings $f(L)$ and $\frac{1}{f(L)}][3]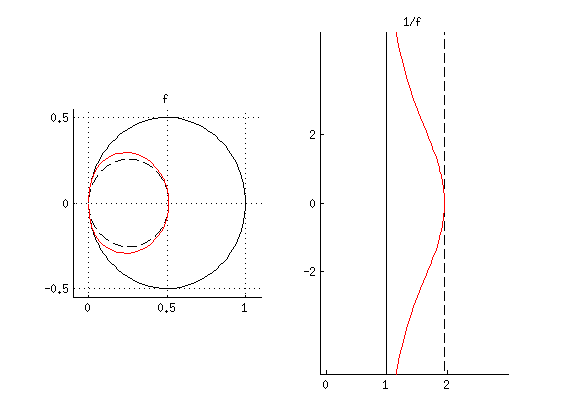
As you can see in the right hand figure (in this case, the Gaussian, but the picture is similar for the uniform and triangle) $1/f(L)$ seems to be bounded in a strip.
The heavy black line is the inequality $$ 1 \le \Re\frac{1}{f(1+i y)}$$ which is easy to prove. The dashed line, not so much.
So the question:
Is it true for all dispersion functions of symmetric distributions that: $$\Re\left[\frac{1}{f(1+i y)}\right]\le \frac{1}{f(1)}$$ which is equivalent to the following $$ \left|f(1+iy) - \frac{f(1)}{2}\right|\ge \frac{f(1)}{2}$$ and $$\frac{d}{dy}\frac{1}{f(1+iy)} \le 0\quad \forall y>0$$.$$\frac{d}{dy}\frac{1}{f(1+iy)} \le 0\quad \forall y>0.$$
We do however know that for symmetric, unimodal $g$:
$f$ is confromal on $H$
$f(\bar{z}) =\overline{f(z)}$ thus $f(x+i0)$ is real,
$f(z)$ constrained the the positive real axis is a decreasing function,
$$\Im f(z)\le 0 \quad\text{and}\quad \Im \frac{df}{dz}\ge0\qquad \forall y\ge0$$
$$|\frac{df}{dz}| < \Re \frac{f(z)}{x}$$
I have tried a variety of approaches, but iI seem to need some stronger relationship between the real and imaginary parts of $\frac{df}{dy}$.
From a distributional standpoint, picking $g$ to be a Cauchy distribution with the same modal height actually produces the dashed line, and picking $g$ to be the Dirac delta function produces the heavy black line.Maybe Maybe in some sense we can think of the space of unimodal distributions and identify the Cauchy and Dirac distributions as extremum.
Any references/thoughts/suggestions would be muchly appreaciatedappreciated.
Cheers,
Pete
[1]: http://www.springerlink.com/index/ln051rp502550471.pdf
[2]: http://www.sciencedirect.com/science/article/pii/016727899190129W
[3]: https://i.sstatic.net/Th7Y8.png
