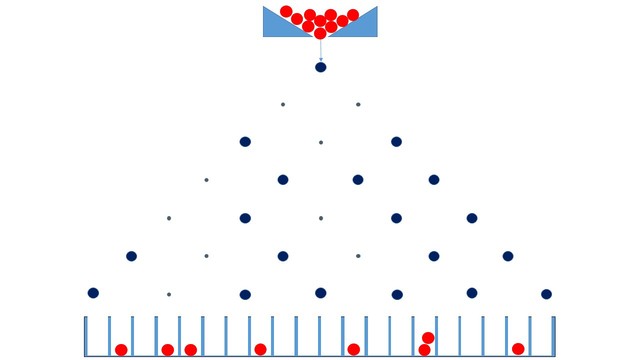
The Bean Machine, also known as a quincunx or the Galton box, is a well known triangular board that contains several rows ($n$) of staggered, but equally spaced pegs. Balls are dropped from the top one by one to avoid interference. They bounce off the pegs and stack up at the bottom of the triangle in bins. The resulting stacks of balls approach the characteristic Bell curve shape for large $n$.
Consider the following altered Bean Machine that now has all the 'prime numbered pegs' (counting top to bottom, left to right) removed:
http://s17.postimage.org/qqctbpftr/Galton_prime_box.jpgBased on what is known about the primes, is there anything that could be predicted about the resulting distribution of balls for large $n$? Is it expected to be skewed to the left or the right? Or does it nicely balance out like the normal distribution?
Thanks.