Return to Revisions

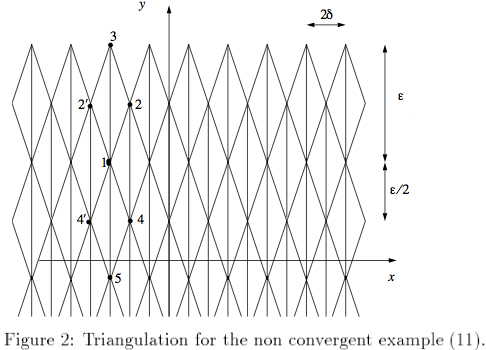
This may not be what you seek, but in the 1996 paper, "Anisotropic refinement algorithms for finite elements" by Goodman, Samuelsson, and Szepessy (.ps link), they show an example of a function $u(x,y)=\frac{1}{2} y^2$, independent of $x$, which solves $\Delta u = 1$ on $\mathbb{R}^2$. But with the triangulation shown below, with $\delta \ll \epsilon$, as $\delta \rightarrow 0$, the finite element equation approximates $\Delta u = 0$ instead of $\Delta u = 1$.