Let $H, G$ be topological groups and $\phi : H \to G$ a group homomorphism. Let $M$ be a paracompact topological space.
For any principal $G$-bundle $P \to M$, a reduction (or sometimes 'lift') of its structure group to $H$ is a principal $H$-bundle $Q \to M$ and a ($G$-equivariant) isomorphism of fibre bundles $Q \times_{\phi} G\cong P$, i.e.
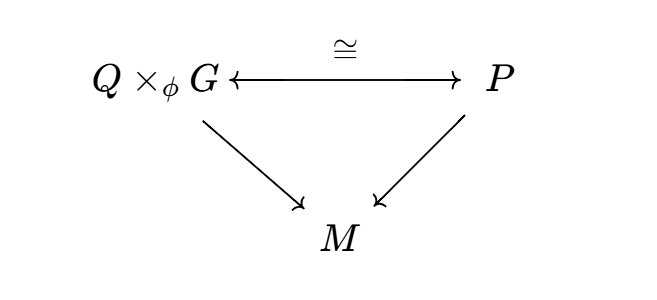
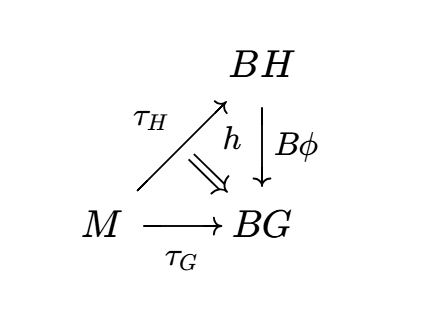
The homotopy-theoretic definition of reductions is as follows. Let $BG$ be the classifying space for principal $G$-bundles. Let $B\phi : BH \to BG$ be a map of classifying spaces corresponding to $\phi$.
Then given a classifying map $\tau_G : M \to BG$, a ($H$-)reduction of the structure group (of the principal $G$-bundle $\tau_G$ classifies, call it $P$) is a lift up to homotopy of this map to $BH$, i.e. a map $\tau_H : M \to BH$ and a homotopy $h : B\phi \circ \tau_H \to \tau_G$, so that we have the diagram
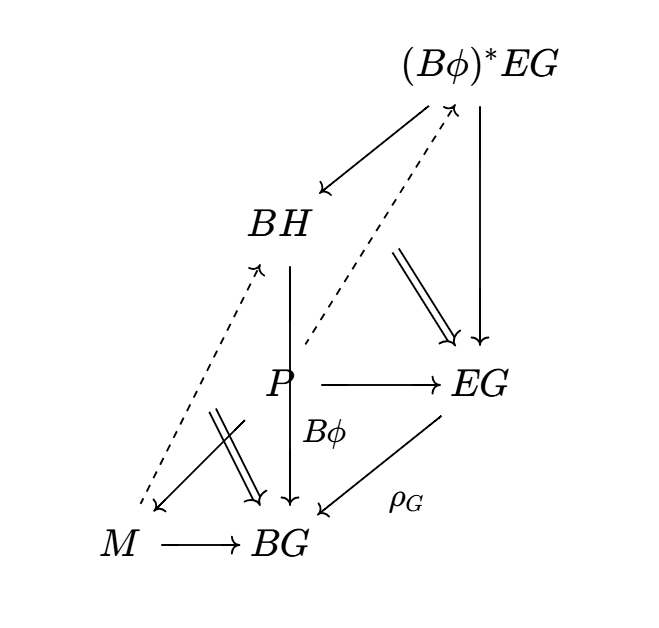
I'd like to see how the homotopy-theoretic definition is equivalent to the principal bundle definition. For example, if I spell out the principal $G$-bundle $P \to M$ here, I presumably get a diagram of the sort
(where I'm not sure if the existence of $P \to (B\phi)^*EG$ follows directly from the admission of an $H$-reduction or not)
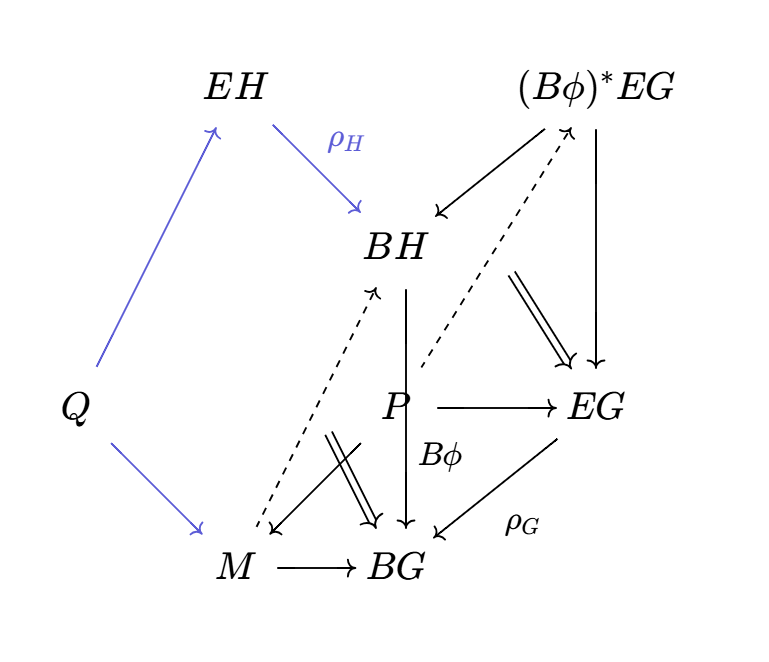
Then the (first) question would be where the principal $H$-bundle $Q \to M$ fits into the above. If I include the pertinent pullback square in blue,
... what other arrows need I add to the above diagram to get the complete story?
Edit: I am looking to understand whether each homotopy $h : B\phi \circ \tau_H \to \tau_G$ leads to an isomorphism of principal $G$-bundles $\theta_h : Q \times_{\phi} G \to P$ and vice-versa. My intuition is that these two data encode equivalent information (but I may be not be exactly right, inasmuch as a proposition of mutual existence is not a 1:1 correspondence).
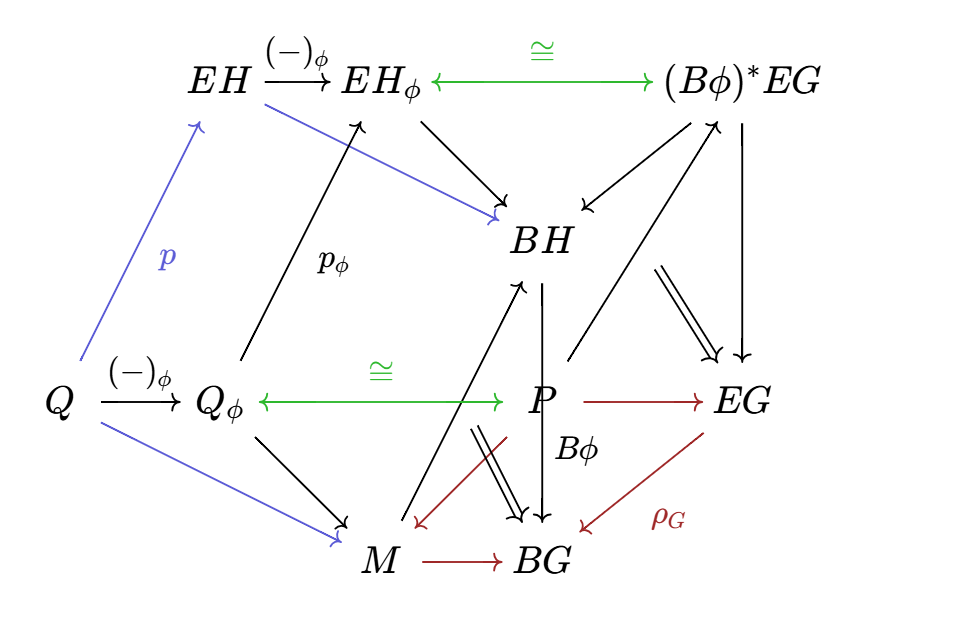
As per Baylee's answer below, it seems convincing to organise the data as follows.
The question remains how to read the above diagram to infer whether a (lift up to) homotopy $h$ is the same as an isomorphism $\theta_h$.
If further there are any references that explicate the equivalence between these two definitions, please do post them here.
Note: A reminiscent result shows up in Proposition 9.38 of Fre12, but this result remarks on (strict) lifts of $M \to BG$ (taken up to homotopy) rather than its lifts up to homotopy, which are different. I believe only the latter is relevant for the homotopy-theoretic definition I'd like to work with.