Let $H, G$ be topological groups and $\phi : H \to G$ a group homomorphism. Let $M$ be a paracompact topological space.

For any principal $G$-bundle $P \to M$, a reduction (or sometimes 'lift') of its structure group to $H$ is a principal $H$-bundle $Q \to M$ and a ($G$-equivariant) isomorphism of fibre bundles $Q \times_{\phi} G\cong P$, i.e.

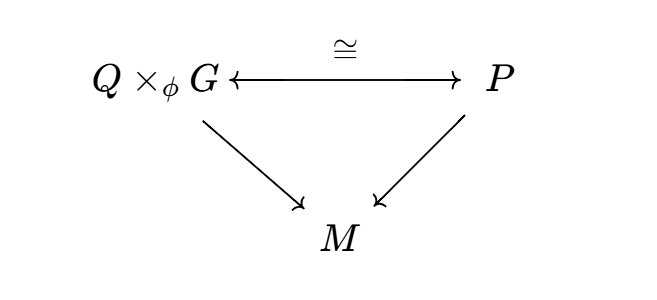
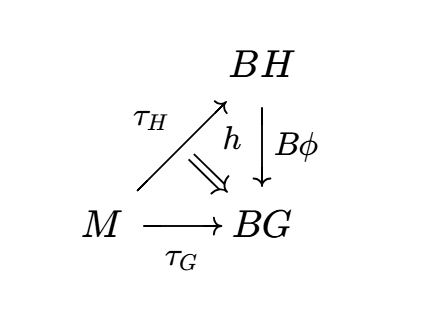
The homotopy-theoretic definition of reductions is as follows. Let $BG$ be the classifying space for principal $G$-bundles. Let $B\phi : BH \to BG$ be a map of classifying spaces corresponding to $\phi$.

Then given a classifying map $\tau_G : M \to BG$, a ($H$-)reduction of the structure group (of the principal $G$-bundle $\tau_G$ classifies, call it $P$) is a lift up to homotopy of this map to $BH$, i.e. a map $\tau_H : M \to BH$ and a homotopy $h : B\phi \circ \tau_H \to \tau_G$, so that we have the diagram

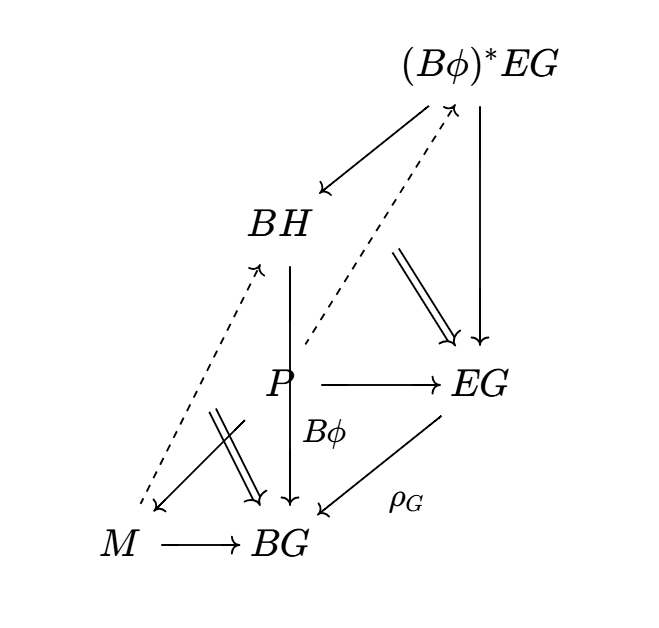
I'd like to see how the homotopy-theoretic definition is equivalent to the principal bundle definition. For example, if I spell out the principal $G$-bundle $P \to M$ here, I presumably get a diagram of the sort

(where I'm not sure if the existence of $P \to (B\phi)^*EG$ follows directly from the admission of an $H$-reduction or not)

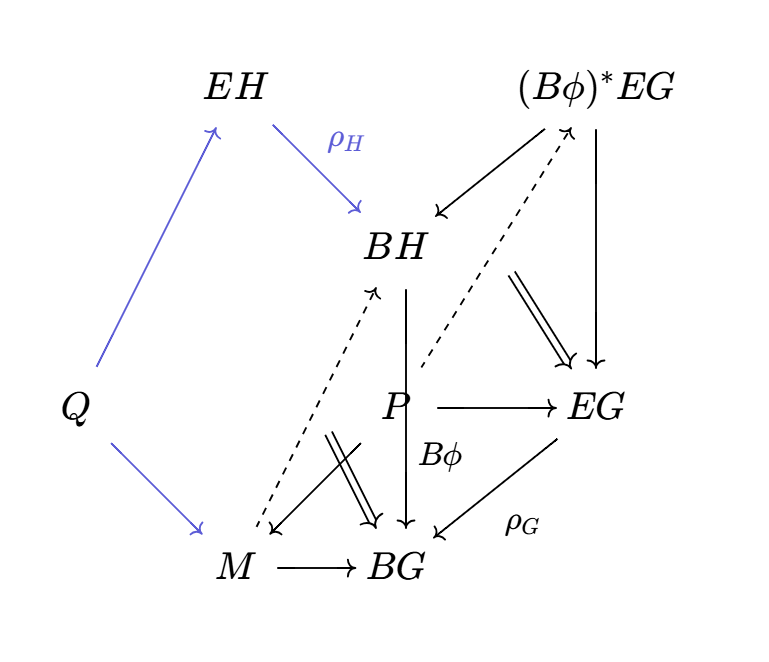
Then the (first) question would be where the principal $H$-bundle $Q \to M$ fits into the above. If I include the pertinent pullback square in blue,

... what other arrows need I add to the above diagram to get the complete story?

If further there are any references that explicate the equivalence between these two definitions, please do post them here.

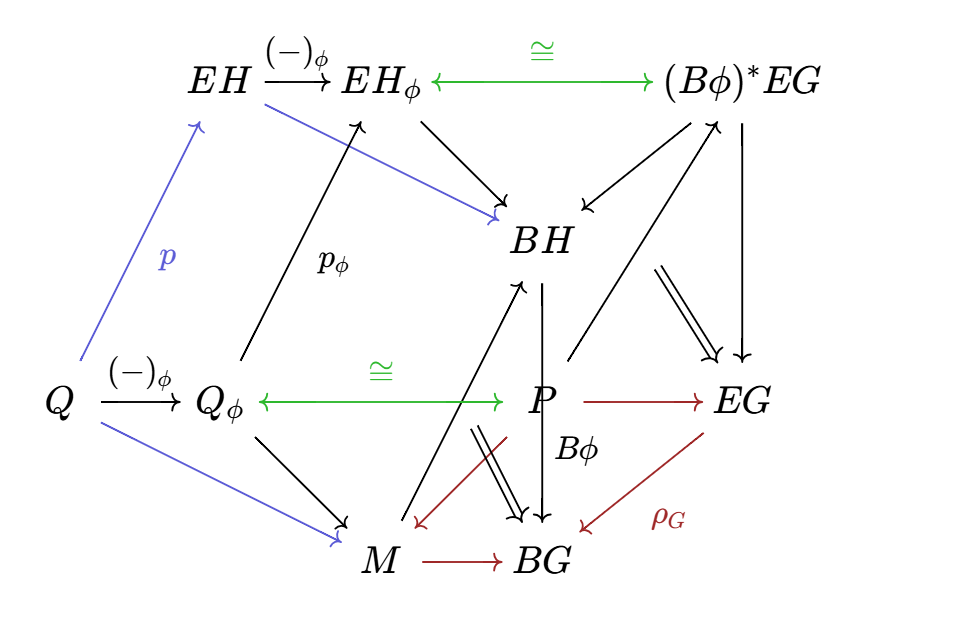
Edit: I am looking to show that each homotopy $h : B\phi \circ \tau_H \to \tau_G$ leads to an isomorphism of principal $G$-bundles $\theta_h : Q \times_{\phi} G \to P$ and vice-versa. My understanding is that these two data encode equivalent information. As per Baylee's answer below, they should be organised as follows.

The question remains how to read the above diagram to infer that a (lift up to) homotopy $h$ is the same as an isomorphism $\theta_h$.