Let $U \subset \mathbb{R}^m$ be an open domain. I'm trying to come up with a measure of its degree of reflectional symmetry and I have a question. The post in two-part, where in PART I introduce the definition and some examples, and PART II asks the question. I do believe much better definitions exist in the literature, but not remotely being a convex geometer, I've no idea about them, thus resources highly appreciated!
PART I:
The way I'm thinking about reflectional symmetry of $U$ above is as follows. We define $U$ to have a symmetry of degree $r, 1\le r \le m,$ if there exists
- a point $x_0 \in \mathbb{R}^m,$
- an orthonormal basis $\{e_1\dots e_m\}$ of $\mathbb{R}^m$ and
- a permutation $\sigma:\{1\dots m\}\to \{1\dots m\}$ so that
for every $y\in U-x_0:=\{u-x_0: u\in U\}, y= \sum_{i=1}^{m}y_ie_i \in U-x_0 \iff \sum_{i=1}^{r}-y_{\sigma(i)}e_{\sigma(i)} + \sum_{i=r+1}^{m}y_{\sigma(i)}e_{\sigma(i)} \in U-x_0.$
The intuition behind the above definition is that one needs to translate $U$ by $x_0$ before finding an orthonormal basis with respect to which the co-ordinates $(y_1\dots y_m)$ satisfy (after a permutation $\sigma$) if $(y_1,\dots y_r,y_{r+1} \dots y_m)\in U$ then $(-y_1,\dots -y_r, y_{r+1} \dots y_m),$ and vice versa.
It's clear that the degree of symmetry is invariant under an isometry of the Euclidean space.
Some examples:
For the interior of the ellipse in the plane $\{(y_1,y_2):(y_1-a_0)^2/a^2 + (y_2-b_0)^2/b^2 \le 1\}, x_0=(a_0,b_0),$ the orthonormal basis is the canonical one. Here $r=2.$
If we rotate the above ellipse, everything else stays the same but the orthonormal basis becomes the corresponding rotation applied to the canonical basis. Here $r=2.$
For the half space $\{w\in \mathbb{R}^m: w^{T}w_0 >0\},r=m-1, x_0=0,$ and the orthonormal basis is $\{e_1\dots e_{m-1},e_m:=w_0/||w_0||\}.$
For the unbounded triangular domain below (related to the example#2 in PART II below), it's depicted in the image below, where $e_2$ is the angle bisector. The symmetry is only along $e_1,$ so $r=1.$
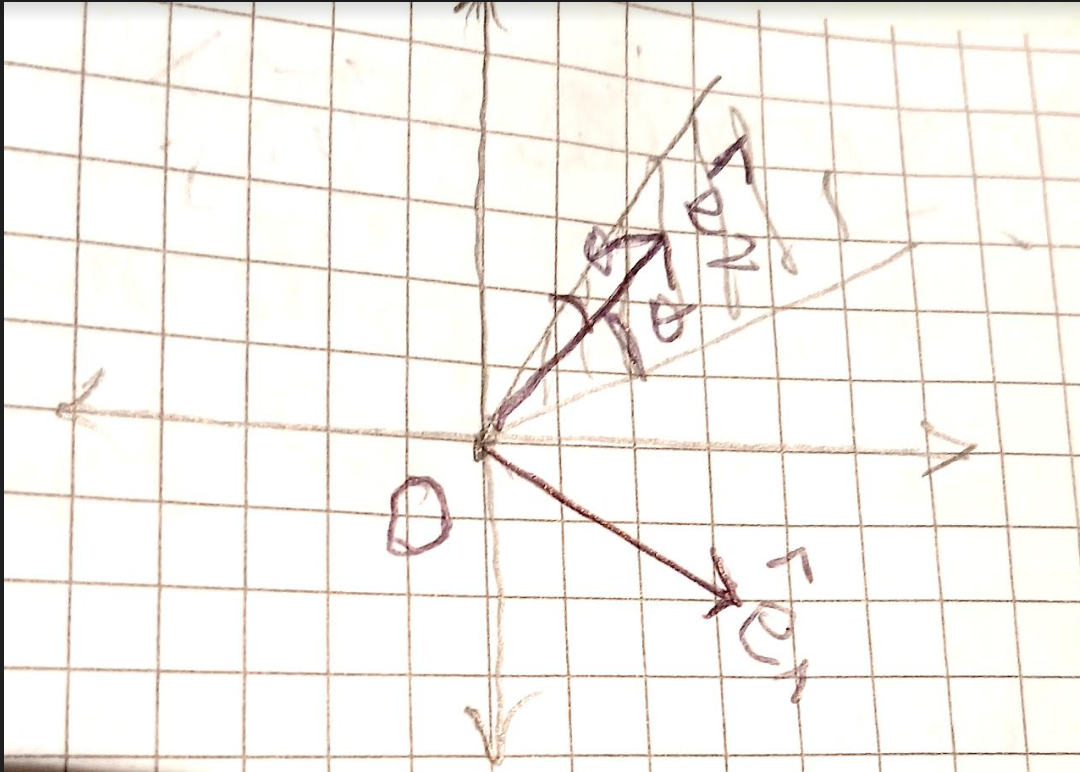
Note that the point $x_0$ above need not be unique, e.g. take in the half space example, take any $x_0$ that's orthogonal to its boundary. Also the orthogonal basis need not be unique as well: e.g. for the interior of a circle centered at the origin, one can take any orthonormal basis.
PART II:
With the above preparation, My question is: what's the degree of symmetry of unbounded convex polytopes $P_G$ that are finite intersection of the half spaces given by $\{y\in \mathbb{R}^m:y^T(A-I)v >0\}, v$ fixed, where $A\in G,$ a finite subgroup of $SO(m)$? It's clear that $P_G$ is an unbounded convex polyhedron (in higher dimensions). I think we can take $x_0:=0,$ but then how to choose the orthonormal basis for $P_G-x_0=P_G?$
I'm taking some toy examples, e.g.
- $m=2, G:={I_m}, r=m=2, x_0=0.$
- $m=2, G:=\mathbb{Z}_k, U$ is the fundamental domain of the action by rotation, containing a point $p$ . Here $r=m=1, x_0=0.$ (the above image applies in this case). Symmetry along $e_1$ but not along $e_2.$
- $m=d, G:=\mathbb{Z}_k, U$ The action is $g:(z\in \mathbb{C}, x \in \mathbb{R}^{d-2})\mapsto (e^{2\pi gi/k}z, x)\forall g\in G.$ Take the fundamental domain containing a point $p$ . Here $r=d-1, x_0=0.$ The domain is symmetric in the directions perpendicular to $p$ but not along $p.$
But currently I'm not sure how to handle general finite subgroups $G\subset SO(m).$ I feel that if the group $G$ has $k$ generators, then the degree of symmetry $r:=m-k.$ If you know any literature that does something similar and can answer the question for general $G,$ that'd be highly apprciated.

