Define $f_1(z) = \cos(z)$, $f_{n+1}= \cos(f_n (z)) $, The question is: If $\lim\limits_{n \to \infty}f_n(z)$ exist for certain $z \in \mathbb{C}$? And what is the limit for such $z$?
We know that for real numbers $z\in \mathbb{R}$, the limit exists and is the solution to the equation $x=\cos(x)$, this result is elementary. However, the complex case seems more intricate due to the unbounded nature of the cosine function on $\mathbb{C}$ and the existence of infinitely many solutions to $\cos(z)=z$.
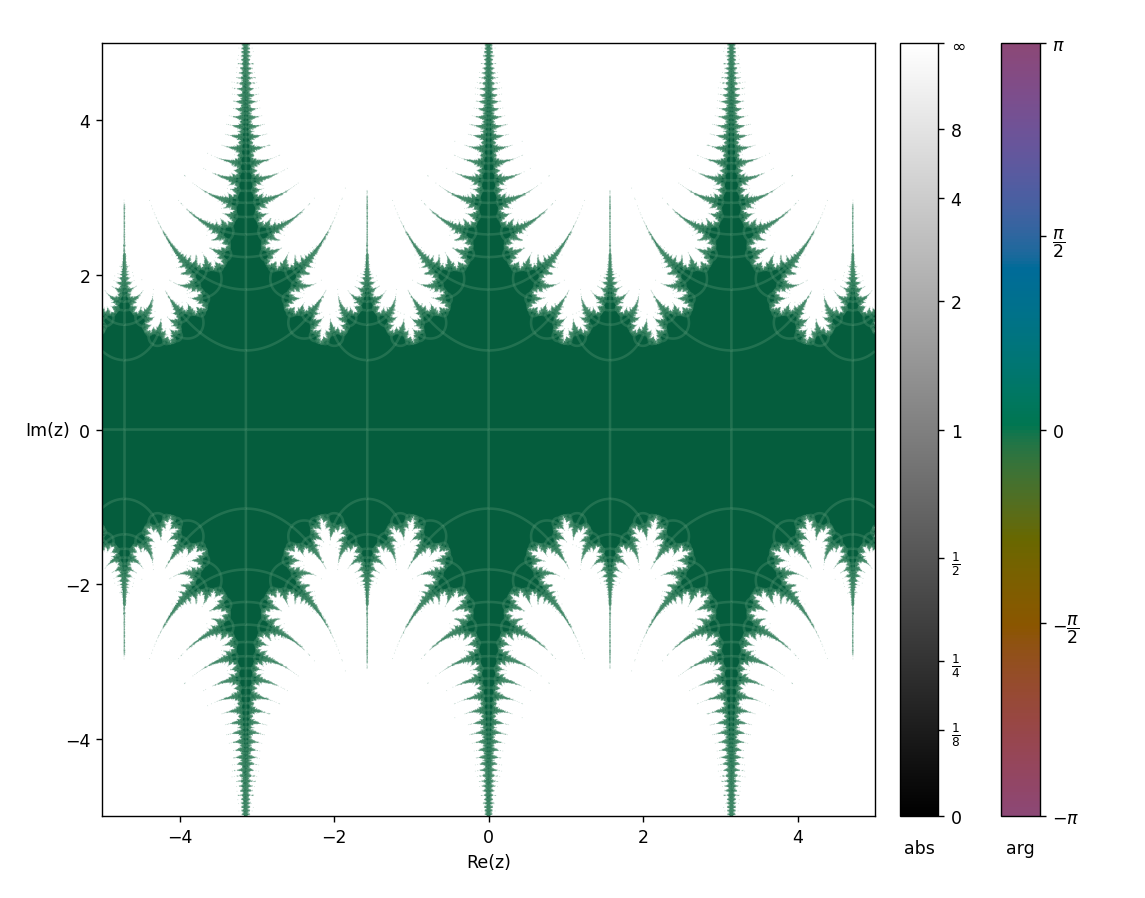
This is the graph of $f_{200}(z)$:
It seems that if the limit exist then the limit is the real solution for $\cos(z)=z$, For some reason it seems that the other fixed points of $\cos(z)$ doesn't "attract" the sequence of points, only the real solution do.