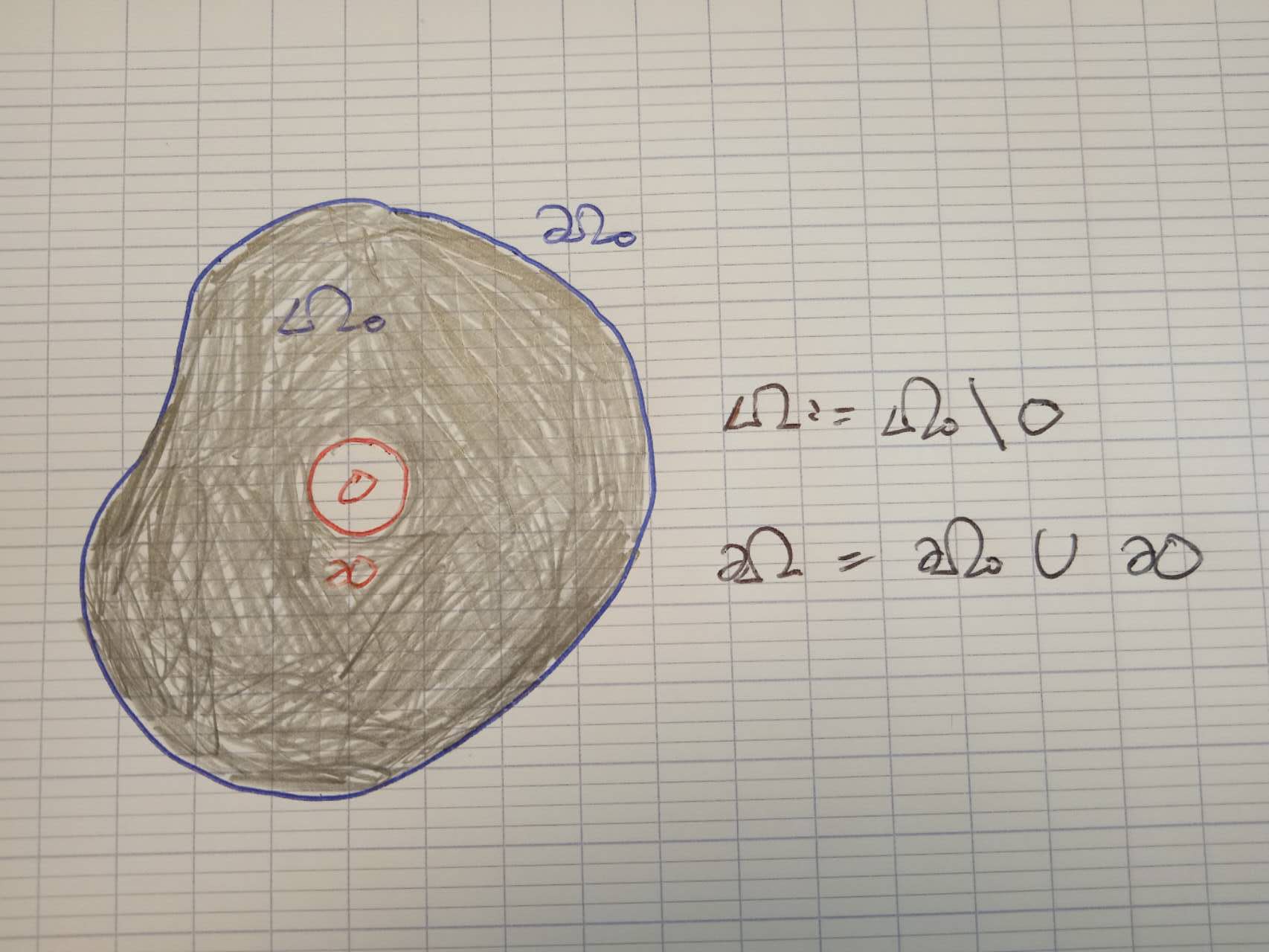
Let $\Omega_0\subset\mathbb R^d$ be open and bounded with sufficiently smooth boundary $\partial\Omega_0$. Let $O\subset \Omega_0$ be a random open subset. Set $\Omega:=\Omega\setminus O$. Consider some second order (parabolic or elliptic) PDE $u$ on $\Omega$ (together with some initial and boundary conditions). Under suitable conditions, the "solution" $u$ exists and is random (depending on the random domain $\Omega$). Is there a SPDE model for related studies of such problem?
An illustrating example is as follows. Let $\Omega_0$ (see the figure below) represent a pool and consider a plastic duck whose trajectory is modelled by some diffusion process $X$. A kid aims to capture the duck by throwing rings (modelled by the random holes inside $\Omega_0$). For example, the child throws one ring (corresponding to the hole $O$) and the game ends once the duck hits the boundary $\partial\Omega$ with $\Omega:=\Omega_0\setminus O$. The kid wins if the duck is trapped in a disc (it hits $\partial O$ prior to $\partial \Omega_0$) and loses if the duck escapes (it hits $\partial \Omega_0$ prior to $\partial O$). We aim to use PDE techniques to analyse the winning probability.