I'm currently working on strong Whitney's embedding theorem, using Adachi's notes on Embeddings and Immersions. But I am stuck on a statement that Adachi comments without proof about adding a unique self-intersection to a smooth immersion $f:M^n\to\mathbb{R}^{2n}$ of a $n$-dimensional manifold (page 65, theorem 2.9). Simplifying we have the next problem: given an smooth immersion $f:\mathbb{R}^n\to\mathbb{R}^{2n}$ that has not self-intersection in a open neighborhood $B(0)$ of the origin, we can replace $f$ in $B(0)$ by an specific immersion $\beta:\mathbb{R}^n\to\mathbb{R}^{2n}$ with a unique self-intersection on $B(0)$, to obtain an immersion $g:\mathbb{R}^n\to\mathbb{R}^{2n}$ with a unique self-intersection on $B(0)$ and such that $g=f$ outside $B(0)$.

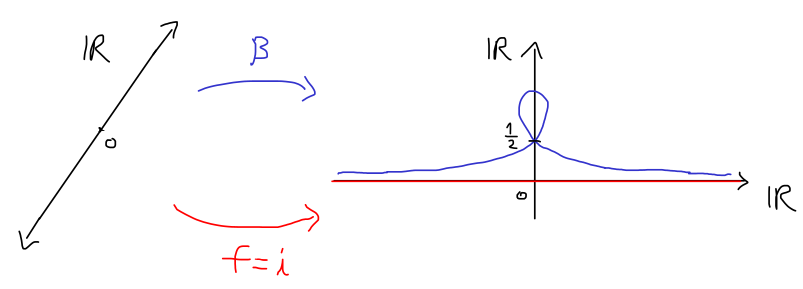
For example, when $n=1$ we can work with $f=i:\mathbb{R}\hookrightarrow\mathbb{R}^2$ the canonical inclusion and $\beta:\mathbb{R}\to\mathbb{R}^2$ the immersion given by $\beta(x)=(x-\dfrac{2x}{1+x^2},\dfrac{1}{1+x^2})$ with the unique self-intersection point at $\beta(1)=\beta(-1)=(0,\dfrac{1}{2})$ (see image below).

In this simple case it's natural for my to take a smooth Uryshon function $\lambda:\mathbb{R}\to[0,1]$ such that $\lambda=1$ in $[-2,2]$ and $\lambda=0$ in $\mathbb{R}\setminus]-3,3[$, and then define the linear combination

$$g(x)=(1-\lambda(x))f(x)+\lambda(x)\beta(x)=\left(x-\lambda(x)\dfrac{2x}{1+x^2},\dfrac{\lambda(x)}{1+x^2}\right),$$

wich is a smooth map equal to $\beta$ on $[-2,2]$ and equal to $f=i$ on $\mathbb{R}\setminus]-3,3[$, with a unique self-intersection point at $g(-1)=g(1)=\beta(-1)=\beta(1)=(0,\dfrac{1}{2})$ (see figure below).

My problem is that, even though $g$ is immersion on $[-2,2]\cup(\mathbb{R}\setminus]-3,3[)$, I can't prove that it's immersion on $]-3.-2[\cup]2.3[$ (see the purple intermediate part of $g$ in the picture), because I can't have control over the derivative of the Uryshon function in this domain. I also try approximate $g$ with a immersion $\widetilde{g}$ wich coincides with $g$ on $[-2,2]\cup(\mathbb{R}\setminus]-3,3[)$, but this not ensure that the aproximation is injective in $]-3.-2[\cup[2.3]$, wich is required for have only one self-intersection.

Maybe someone has a hint to solve this in my example, or there is a better way to combine the immersions $f$ and $\beta$ instead of using the linear combination with the Uryshon function?